0
$\begingroup$

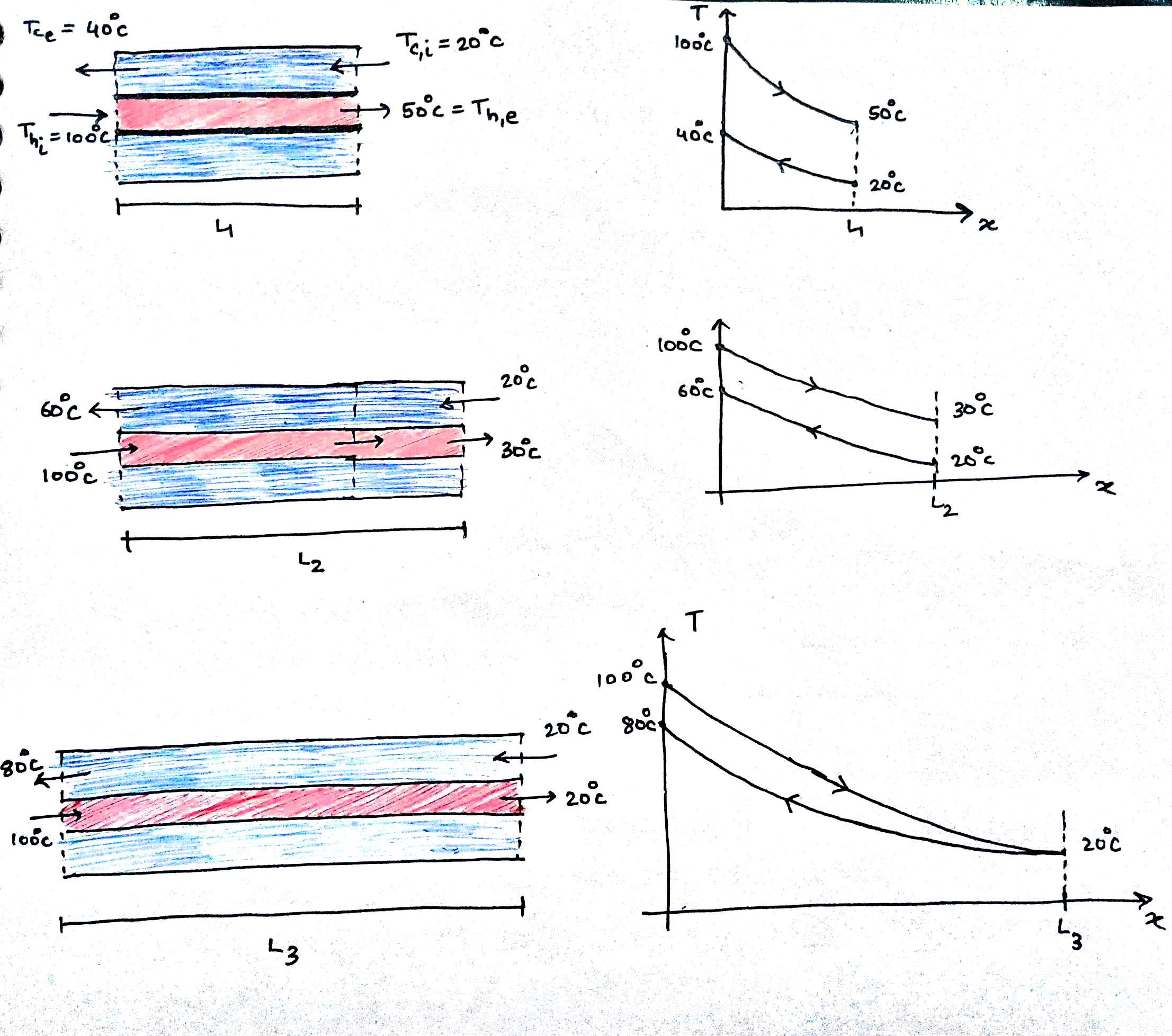
Consider a counter flow double pipe heat exchanger inside which the cold fluid enters at 20 degrees Celsius and hot fluid enters at 100 degrees Celsius. Initially the length of the heat exchanger is L1 and the temperature profiles are shown in the figure. If I continue to increase the length of the heat exchanger the temperature of the hot fluid at exit decreases and of the cold fluid at the exit increases. Let us say, at some length L3 the exit temperature of the hot fluid becomes equal to the inlet temperature of the cold fluid. What happens to the exit temperatures and temperature profiles once I continue to increase the length futher?

enter image description here

Improve this question
$\endgroup$
5
  • $\begingroup$ Are you asking if the temperatures change after reaching equilibrium? $\endgroup$
    – Adrian Howard
    Commented Aug 17, 2021 at 13:27
  • 1
    $\begingroup$ If you work it out, you'll find that L3 is infinite. $\endgroup$
    – Chet Miller
    Commented Aug 17, 2021 at 13:51
  • $\begingroup$ @AdrianHoward I was thinking that may be the 80 degrees will also rise to 100 if I keep of increasing the length. $\endgroup$
    – Harshit Rajput
    Commented Aug 17, 2021 at 13:57
  • $\begingroup$ @ChetMiller So increasing beyond L3 doesn't change the exit temperatures at all? Temperatures still remain 20 and 80. However, what limits 80 to not become 100, upon further increase. $\endgroup$
    – Harshit Rajput
    Commented Aug 17, 2021 at 14:03
  • $\begingroup$ If the length is infinitely long, what does increasing it even mean? $\endgroup$
    – Chet Miller
    Commented Aug 17, 2021 at 14:07

1 Answer 1

Reset to default
1
$\begingroup$

What we are saying here is that for an exchanger length less than infinite, the temperature difference cannot be zero. It can only approach zero as we make the exchanger longer and longer.

Improve this answer
$\endgroup$
3
  • $\begingroup$ I'm convinced that exit temperature of the hot fluid will approach entry temperature of the cold, but I don't know why it comes as a feeling to me that 80 should increase to 100. Any possible way to prove that 80 can't go up to 100, once exit and inlet temperatures of the hot and cold fluids respectively, match. $\endgroup$
    – Harshit Rajput
    Commented Aug 17, 2021 at 14:49
  • $\begingroup$ Have you considered writing down the equations and solving them to see what they tell you? $\endgroup$
    – Chet Miller
    Commented Aug 17, 2021 at 16:48
  • 1
    $\begingroup$ I guess I got it, I wrote energy balance equations for hot and cold fluids and if heat capacity rates of the fluids are unequal, there can't be an equality between the temperatures of the hot and cold fluids on both sides. $\endgroup$
    – Harshit Rajput
    Commented Aug 18, 2021 at 17:50

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.