The question really confuses me. But I will add some details that may help clarify things. You may be familiar with the balance of angular momentum: $\dot{\bf L} = {\bf T}$, where ${\bf T}$ is the applied torque to the body. In your example, ${\bf T} = {\bf 0}$ and we have torque-free motion. Therefore, ${\bf L}$ is a constant vector. It is frozen in inertial space and determined entirely by the initial angular velocities and moments of inertia. That is, if we have the inertial persepective.
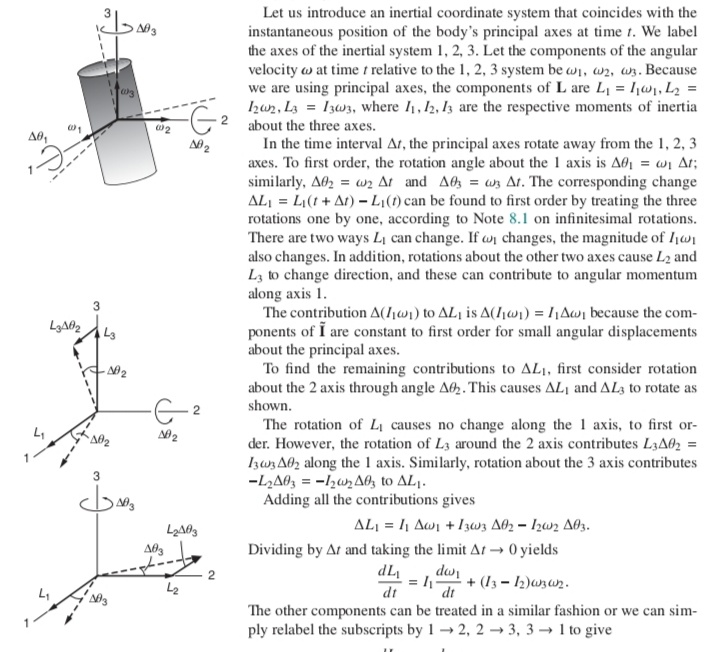
If instead, we are rotating with the cylinder, and looking out at ${\bf L}$, then ${\bf L}$ will appear to change. How do we express this concept mathematically? Well, if I affix three vectors ${\bf e}_1$, ${\bf e}_2$, and ${\bf e}_3$ which co-rotate with and are frozen in the cylinder, then I can use them as a basis to describe ${\bf L}$: ${\bf L} = L_1 {\bf e}_1 + L_2 {\bf e}_2 + L_3 {\bf e}_3$. It is important to note that, because we are changing our perspective to the co-rotating basis, $L_i$, the components of ${\bf L}$ along ${\bf e}_i$, appear to change, despite the fact that ${\bf L}$ as a vector is frozen in inertial space and unchanging.
Doing the math then, we obtain $\dot{\bf L} = \sum_i \dot{L}_i {\bf e}_i + \boldsymbol{\omega} \times {\bf L}$. And we know this quantity must be the zero vector for torque-free motions. Now using $L_i = I_i \omega_i$, we obtain Euler's equations, where $I_i$ is unchanging along body-fixed axes, and $\omega_i$ are components of $\boldsymbol{\omega}$ along ${\bf e}_i$.