With no intent to violate the rules, I wish to ask specific questions related to this general question. For that reason, I will attempt to specify the kind of general answer I seek. It is most likely that the best answer to the current question will be a reference to a discussion of purely intrinsic properties of a differentiable manifold, or something similar.
This is Box 9.1 from Misner, Thorne and Wheeler's Gravitation.
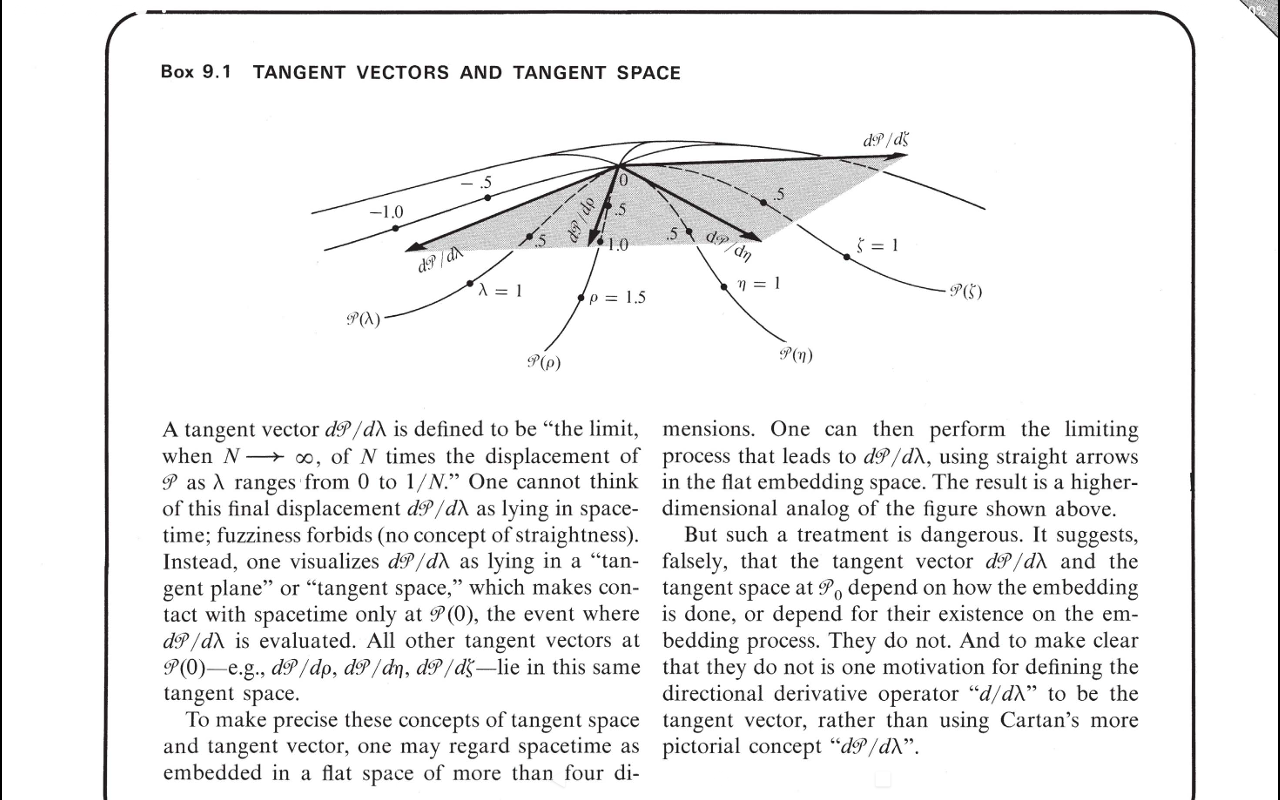
The discussion assumes a metric-free, geodesic-free spacetime. The authors never explain what properties this spacetime does posses. For example, what does it mean to multiply the displacement of $\mathcal{P}$ as $\lambda$ ranges from $0$ to $1/N$? With no concept of distance what does $\lambda$ even mean?
What properties are we to attribute to this spacetime? Must we assume that locally it approximates the spacetime of special relativity? Can we speak of open balls centered on an event? Can we speak of a neighborhood of an event becoming arbitrarily small?
The authors do speak of the possibility of a higher dimensional "flat" "embedding space", but call it extraneous.