6
$\begingroup$

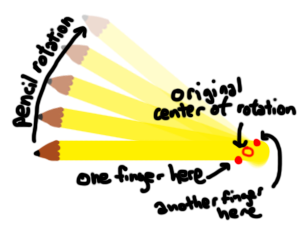
If I hold a pencil at its end and spin it, throwing it upwards, it will spin about its end, but will soon start spinning around its center. How is this?

I would draw the following torque diagram for while it's in the air:

  • Object: uniform thin rod with length $\ell$ and moments $I_{center}=\frac 1 {12} m\ell^2$ and $I_{end}=\frac 1 3 m\ell^2$)
  • Center of rotation some small distance $d$ from the end
  • Torque $m\vec g$ downward, at center of mass, with $\theta = 90°$ and $r = \frac \ell 2 - d$
  • Possibly wind resistance $\vec D$ upward, at center of mass, with $\theta = -90°$ and $r = \frac \ell 2 - d$

Thus, $\vec \tau = \sum {\vec r \times \vec F} = \sum {rF~sin\theta} = \left (\frac \ell 2 - d\right)(m\vec g - \vec D)$. I could see how this might cause it to spin, but how does the center of rotation to move?

EDIT: Here's a picture because apparently I wasn't clear. It definitely is spinning around the end (or close to it, anyway) before I release the pencil.

Pencil image

Improve this question
$\endgroup$
4
  • $\begingroup$ Because that's the pivot point when I'm holding it. $\endgroup$
    – wchargin
    Commented May 7, 2013 at 2:15
  • $\begingroup$ @007 please see my edit. $\endgroup$
    – wchargin
    Commented May 7, 2013 at 2:51
  • $\begingroup$ Ok then it's nice. You also take the holding at two points into consideration.+1 $\endgroup$
    – ABC
    Commented May 7, 2013 at 2:59
  • $\begingroup$ @007 but the holding at two points just provides the $\omega_0$; while it's in the air, the center of rotation moves without me touching it… $\endgroup$
    – wchargin
    Commented May 7, 2013 at 3:00

2 Answers 2

Reset to default
2
$\begingroup$

This looks like an example of the Tennis Racket Theorem. Some axes of rotation for a rigid body are more stable than others. If the initial rotation axis does not correspond to one of the principal axes, a wobble can grow and cause the rotation axis to move to a principal axis. This is a result of Euler's Equations of Motion and the moments of inertia.

The tennis racket theorem is a result in classical mechanics describing movement of a rigid body with three distinct angular momenta.

Improve this answer
$\endgroup$
1
  • $\begingroup$ In your last sentence I think you mean "three distinct moments of inertia about its major axes". The angular momentum is constant - it's just that for certain object, this angular momentum can exist as a combination of rotations about the three axes and their orientation in space, and that at a given moment the rotation results in an "internal" torque that changes the rotation from one principal axis to another. $\endgroup$
    – Floris
    Commented Jan 27, 2015 at 2:26
0
$\begingroup$

Not sure if I am interpreting your description of the problem correctly, but if I take the initial conditions (using nice round numbers) as a rod of $r = 1\:\mathrm{m}$ length, pivoting about one end at at $\omega = 1\:\mathrm{rad/s}$ at $t=0$, I can decompose the motion as a COM motion of $v=r\,\omega/2$ with a spin about the COM of $\omega$. If I plot the motion (ignoring gravity), I get:

enter image description here

where $t=0$ is the horizontal line at the bottom and the "pencil" is moving upwards after release. While the initial conditions are set by the spin around the end, the following motion is still mechanics about the COM.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ That graph looks accurate, but it doesn't answer my question. Over time the pencil's rotation will change so that it starts to rotate about the center of mass. My question is, why does this change in the center of rotation occur? $\endgroup$
    – wchargin
    Commented May 7, 2013 at 3:11
  • $\begingroup$ @WChargin At t=0, it was rotating around your finger and at the same time was rotating around the center of mass. Once you release it, the torque you were applying to keep one end fixed is gone and the end in your fingers starts to move, following the motion set by the COM rotation + COM velocity. $\endgroup$
    – Jason A
    Commented May 7, 2013 at 3:15
  • $\begingroup$ How can it be rotating about two points at once at time $t=0$? As the saying goes, there can only be one pivot point... $\endgroup$
    – wchargin
    Commented May 7, 2013 at 3:16
  • $\begingroup$ There can be more than one if each of the pivot points is moving with respect to each other. The COM is a moving pivot point (in the lab frame), whereas your finger is a fixed pivot point. To your fingers, the left end is moving up at $v=r\omega$ and the right end has v=0 (again at t=0). About the COM frame, the left end is moving up at $v=(r/2)\omega$ and the right end is moving down at $v=-(r/2)\omega$. Does that help? $\endgroup$
    – Jason A
    Commented May 7, 2013 at 3:23
  • 1
    $\begingroup$ @WChargin To expand upon the above, you can calculate angular momentum around any point (pivot) you want. It is just convenient when dealing with free motion to use the COM, because the COM motion and the angular momentum separate, i.e. the object spins around the COM and the COM goes about its business. You are free to choose a different coordinate system. Same physics, different numbers, same outcome. It is all relative. $\endgroup$
    – Jason A
    Commented May 7, 2013 at 3:34

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.