Phonons and Modes

Question

I am trying to understand how normal modes, optical and acoustical cases and longitudinal/transversal propagation of waves are all together related.

Lets say we are have a clystal chain (for simplicity) in 3D and we have more then 2 different atoms per unit cell.

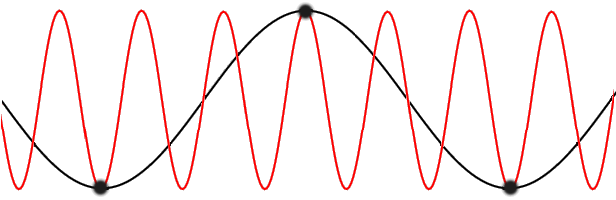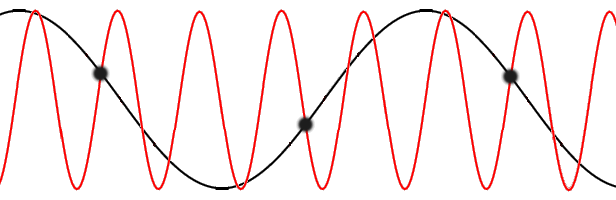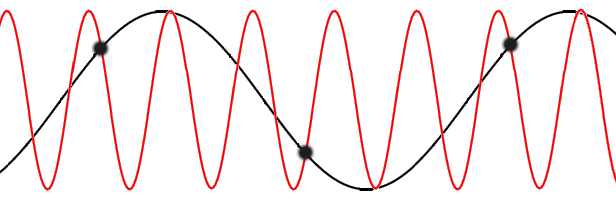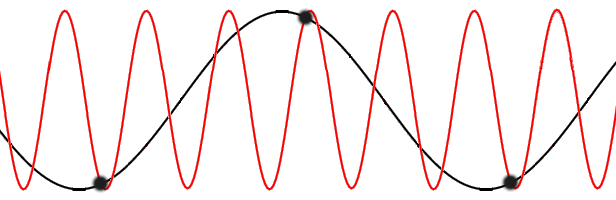
A normal mode would be, said in a simplistic way, a wave pattern + nodes that we can "see" along the chain. We have as many normal modes are we have atoms. Max normal mode would be for max wavenumber of $\pi/a$ (a lattice constant). Basically we would have a standing wave.

Now each mode (wavenumber value) can change it's energy somehow, which will result in the same pattern but with higher energy, correct?

And depending one their motion in relation to the propagation of the wave in the crystal we can say that we are having a transversal or longitudinal wave.

That much I understand, but what i don't get is how do we differ between the optical and acoustic case? (i am not using the word mode here to not create confusion with the normal modes).

In Wikipedia for the optical case it says : " Atoms ( 2 or more different per unit cell) move in opposite direction. Isn't that the case only for when the wave number is maxima? Meaning for the last normal mode, which is characterized for the max. value of wavenumber. As I said above, only for this wavenumber value the particles move in an opposite direction, which results into a standing wave, and for the optical case the atoms must move in the opposite direction? How can we have an optical case if the wavenumber isn't in it's maximal value?

Answer 1

The optical modes in crystal vibrations correspond to modes whose wavelength is smaller than the smallest separation between the atoms (in contrast to the acoustic modes, whose wavelengths are longer than the separation). This puts a limit (UV cutoff) on how energetic the modes can be which, as you correctly observe, is $\pi/a$, where $a$ is the separation between the atoms. For such modes, the quasimomentum of this mode will be less than $\pi/a$. This phenomenon can be seen through this gif (Wikipedia):

Notice that although the momentum of the mode (red) has a shorter wavelength than $a$, the lattice cannot "see" this finely, and so exhibits the behavior of a less-energetic mode (black). As a result, optical modes "decay" into acoustic modes. This is in accordance with Noether's theorem for crystals, which says that momentum is conserved modulo $\pi/a$ (alternatively, quasimomentum is conserved), and is also why band structure diagrams can be "reflected" into the first Brillouin zone.