$PdV =VdP$ is true for any reversible cycles?

Question

My Question:

Does the following equation hold, at least for reversible cycles? If not, are there any special conditions that must be met for this to be true? $$PdV=VdP$$

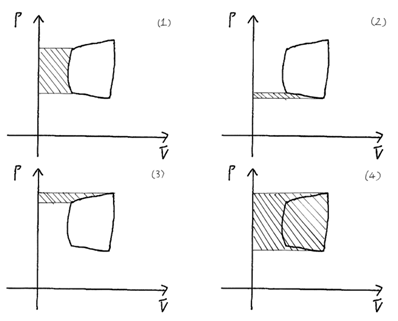
The reversible cycle can be drawn as a loop on the PV diagram. In one cycle, the work done by the cycle is the area of the region enclosed by the loop. This seems to be expressed in many thermodynamic textbooks as the following integral In other words, this may be regarded as a function of P as a function of V.

$$W=\oint P dV \tag{eq.1}$$

However, we can also consider V as a function of P, as shown in the figure below, which also seems to mathematically represent the area of the region enclosed by the loop.

$$\oint V dP = (4)- ((1)+(2)+(3))\tag{eq.2}$$

Fig.1

If I understand correctly, it seems to be mathematically possible to interpret eq1 as the integral around the differential form PdV, and eq2 as the integral around the differential form VdP. In both cases, the loop of the line integral would be mathematically the same curve.

In other words. $$PdV= VdP\tag{eq.3}$$ must always be true to say this.　However, I have never seen this equation in my textbook. So I'm confused.

Answer 1

What is true is that $$\oint P \, dV = - \oint V \, dP$$ because of the area argument you made, noting that the signs are opposite. From this, you can't remove the integral sign to conclude that $P \, dV = -V \, dP$, which is certainly not true.

The integral result is easy to show in general, as $$\oint P \, dV + \oint V \, dP = \oint d (PV)$$ and the net change of $PV$ along a cycle is zero, because it begins and ends in the same place. Of course, this argument also applies for literally any state function along any loop where state functions are always well-defined. (That includes irreversible cycles, but not cycles where you radically depart from equilibrium, like those involving free expansion.) The reason it's not emphasized in textbooks is probably just that it's not often useful.