What is this equation of volumes and pressures of the soap bubble?

Question

Initially the uncharged soap bubble exists with the radius $r$ and the surface tension $T$

Nextly the bubble has been held the potential $V$at the surface of it and the radius of the bubble became $R~~$

$p_{0}:=\text{atmospheric pressure}$

$p:=\text{inner pressure of the bubble before the given of the potential}$

The below equation is held.

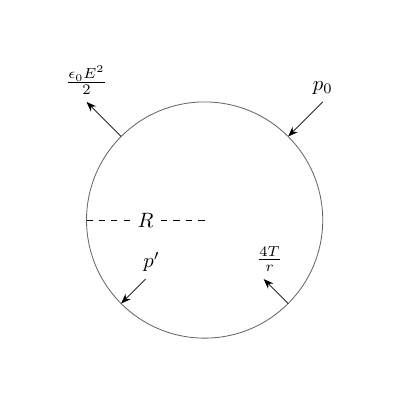
$$p-p_{0}=\frac{4T}{r}$$

$p':=\text{inner pressure of the bubble after the given of the potential}$

$$p'=+p_{0}+\frac{4T}{r}-\frac{\epsilon_{0}E^{2}}{2}$$

$=+p_{0}+\frac{4T}{r}-\frac{\epsilon_{0}}{2}\left(\frac{V}{R}\right)^{2}~~~~~~~~\text{(rightmost term represents the electrostatic energy density)}$

$v:=\text{volume of the bubble before the given of the potential}$

$v':=\text{volume of the bubble after the given of the potential}$

What I can't get currently is the below equation.

$$pv=p'v'$$

Why this equation can be held?

Answer 1

This is just a consequence of Boyle’s law which states

The absolute pressure exerted by a given mass of an ideal gas is inversely proportional to the volume it occupies if the temperature and amount of gas remain unchanged within a closed system.

or $$pV = \text{constant}$$

Now, in this case there is no mention of temperature change, and the mass remains constant, and we can assume air to be roughly an ideal gas, so that $$p_1V_1=p_2V_2$$