This question is with regards to the statement made around page 56 (1971 Edition) in Anthony French's Newtonian Mechanics. He is discussing the choice of a coordinate system where the axes are not necessarily perpendicular to each other. Here is the summary of what I read (as applied to vectors in a two-dimensional plane):
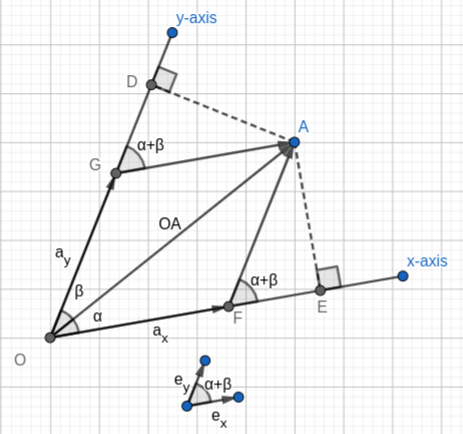
A vector $\vec{A}$ makes the angles $\alpha$ and $\beta$ with two coordinate axes (we'll still call the axes $x-$axis and $y-$axis respectively) not necessarily perpendicular to each other (i.e. $\alpha+\beta$ is not necessarily $\pi/2$). Then, $A_x = A\cdot\cos\alpha$ and $A_y=A\cdot\cos\beta$, where $A_x, A_y$ are the magnitudes of the $x, y$-components of $\vec{A}$ respectively. In the generalized two-dimensional case, we have the relationship $\cos^2\alpha+\cos^2\beta = 1$.
I have redrawn the accompanying figure:
How does French arrive at the generalized relationship: $\cos^2\alpha+\cos^2\beta = 1$ in two dimensions and $\cos^2\alpha+\cos^2\beta+\cos^2\gamma = 1$ in three dimensions?
In the case of perpendicular axes in two dimensions, it is clear why it would hold, since $\alpha+\beta=\frac{\pi}{2}$. But I am not sure how it holds in general.