Why does a laser cavity being finite imply that beam divergence occurs?

Question

I recently read that one of the reasons laser beam divergence occurs is because the radius of the cylindrical cavity is finite, and so the stimulated emission with amplification also occurs for photons travelling along directions not exactly parallel to the axis. I don't really understand why a laser cavity being finite implies that beam divergence occurs, and nor do I understand what this has to do with the photons travelling along directions not exactly parallel to the axis. So why does a laser cavity being finite imply that beam divergence occurs, and what does this have to do with the photons travelling along directions not exactly parallel to the axis?

Answer 1

When you solve Maxwell's equations, one of the easiest solutions that can occur is the solution of a plane wave $E e^{i(\vec{k} \vec{x} - \omega t) }$. Plane waves do have a straight forwards interpretation (in homogenous, isotrope media) if it comes to "direction": They "travel" only in one direction. That is: Their poynting vector points into the same direction as $\vec{k}$, and the wave fronts "travel" in that direction.

However - the wave is spatially unlimited in the directions perpendicular to $\vec{k}$.

Another solution of Maxwell's equations (at least approximately) is the "gaussian beam"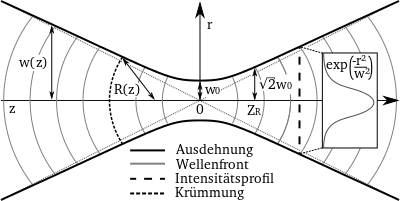

This resembles what we observe as a "light ray" mutch better than the plane wave before, and this is the model that is used to describe a laser from a cavity. As you can see, the beam widens. You don't find solutions to Maxwell's equations that don't show widening, but are spatially confined.

The easiest (and lazy) way to see is that the widening is simply a consequence of Maxwell's equations. However, we can observe a pattern here: The narrower and smaller $w0$ is, the bigger will the widening be. On the other side, with a spatially unlimited wave like the plane wave, no widening occurs at all.

This property is an analogue to the quantum mechanical observation that position and momentum (in this case along the $y$-axis) can't both at the same time be fixed with arbitrary precision. At the moment you require photons to pass through a hole, and limit their position in a direction perpendicular to the optical axis, their corresponding momentum in that direction can't with certainty be set to $0$ anymore.

Answer 2

It comes straightly from uncertainty principle. Consider such schematics of laser cavity :

Uncertainty principle states that :

$$ \Delta x \Delta p_x \geq \frac {h}{4 \pi} $$

Substituting momentum projection into $x$ axis :

$$ \Delta x \Delta (p \sin \alpha) \geq \frac {h}{4 \pi} $$

For small angles $\sin \alpha \approx \alpha$, so :

$$ \Delta x \Delta (p \alpha) \geq \frac {h}{4 \pi} $$

Photon momentum is constant so, :

$$ \Delta x p \Delta \alpha \geq \frac {h}{4 \pi} $$

This gives emitted photon angle uncertainty :

$$ \Delta \alpha \geq \frac {h}{4 \pi \Delta x p} $$

Noticing that $h/p$ is De Broglie wavelength, shortens the equation to :

$$ \Delta \alpha \geq \frac {\lambda }{4 \pi \Delta x} $$

So, the more you squeeze beam in diameter (having smaller laser cavity radius or just focusing beam with a lens into a smaller spot - the more it widens in solid angle afterwards.