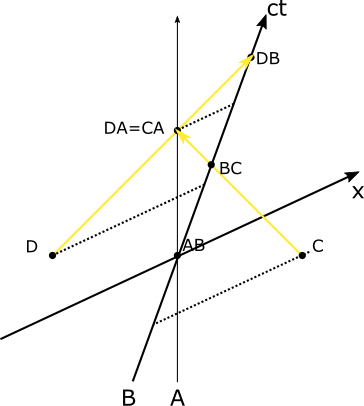
We have two observers, A who is stationary and B who is moving. We have two points C and D that are equidistant from A. At the exact moment B passes A (from A's perspective, if relevant) moving towards C (and at such a distance that in the moment that B passes A, BC = AC in terms of line length, again from A's perspective), light is emitted from C and D. B will encounter the light from C first, then from D, while A will encounter both beams simultaneously (as I understand things). My question comes about what A observes about B, especially if B 'reacts' to C (say by sneezing). The events I'm trying to get in order, from each perspective, are as follows:
- B is beside A
- C emits light
- D emits light
- A encounters C
- A encounters D
- B encounters C
- B encounters D
- B sneezes
- A observes B encountering C
- A observes B encountering D
- A observes B sneezing
- B observes A encountering C
- B observes A encountering D
Attempting to see if I have this right, then: From A's perspective it goes:
- B is beside A
- C emits light, D emits light
- B encounters C
- B sneezes
- A encounters C, A encounters D
- B encounters D
- A observes B encountering C
- A observes B sneezing
- B observes A encountering C, B observes A encountering D
- A observes B encountering D
From B's perspective:
- B is beside A
- C emits light
- D emits light
- B encounters D
- B sneezes
- A encounters C, A encounters D
- B encounters C
- A observes B encountering C
- A observes B sneezing
- A observes B encountering D
- B observes A encountering C, B observes A encountering D
... Is that close to it?