How strong of a magnetic field would I need to lift a human?

Question

I know that there was that experiment they did to lift a frog by using the diamagnetism of the water in it's body, but how strong of a magnet would you need to lift a human in the same way? To lift the frog it was 16T if that helps. Is it a simple body mass to magnet strength ratio or is there a more complex relationship?

Answer 1

How does diamagnetic levitation work?

A non-uniform magnetic field repels an object in which diamagnetism is the predominant magnetic behaviour. We will define magnetic levitation, as the process by which a magnetic field raises an object to some height and holds it in equilibrium with gravity there. In other words, we want the upwards magnetic force to balance the downward gravitational force: $$ (\mathbf m\cdot\nabla)\mathbf B=\rho V\mathbf g $$ The induced magnetic moment $\mathbf m$ in an object of volume $V$ and magnetic susceptibility $\chi$ when place an applied magnetic field $\mathbf B$ is $\frac\chi{\mu_0}V\mathbf B$. Henceforth I will dispense with the vector notation, taking all motion to be along the (vertical) z-axis. At the equilibrium point $z_0$, the magnetic force required is then $$F_B(z_0)=\frac\chi{\mu_0}VB(z_0)\ \frac{\mathrm dB}{\mathrm dz}\bigg|_{z_0}\overset{!}{=}\rho Vg $$ $$ B(z_0)\ \frac{\mathrm dB}{\mathrm dz}\bigg|_{z_0}\overset{!}{=}\frac{\mu_0}\chi\rho g\tag{1} $$ Naturally, $B(z')\ \frac{\mathrm dB}{\mathrm dz}\big|_{z'}>\frac{\mu_0}\chi\rho g$ for $0<z'<z_0$ while $B(z')\ \frac{\mathrm dB}{\mathrm dz}\big|_{z'}<\frac{\mu_0}\chi\rho g$ for $z_0<z'$ so that small deviations from the equilibrium produce simple harmonic motion, ensuring that the object remains levitating in one place.

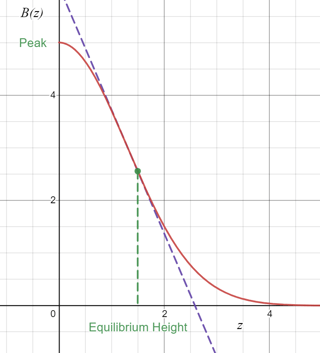
Most setups use some variation of a solenoidal coil structure, designed in a way that the magnetic field strength is strictly decreasing with height - this suggests that an inflection point on the $B(z){-}z$ graph is a suitable height for the equilibrium, as we can impose the SHM restoration condition above. The decrease in the field can be regulated, for example, by changing the density of coils in the solenoid at height $z$, roughly through the relation $\mathrm dB = \mu_0 I \lambda\ \mathrm dz$. Once a particular setup is calibrated, this dropoff profile is actually independent of the field strength at the base, so tweaking this free parameter $B_0$ (e.g. by changing the current through the solenoid) only produces a vertical scaling in the $B(z){-}z$ graph. This means that we can aim to maximise efficiency without having to make repeated measurements of the field gradient.

Here's a magnetic field profile in Desmos. In case it isn't clear, the red curve plots the magnetic field strength at different heights, with "peak" denoting the maximum field strength at the base of the setup, "equilibrium height" is the z-coordinate of the inflection point and $\mathrm dB/\mathrm dz$ is the slope of the purple line. As seen, the shape of most magnetic profiles will allow us to perform accurate linearised analysis at the inflection point.

Levitating a human

To approximate the peak magnetic field strength in a setup of height $z$: $$ \frac12\frac{\mathrm d(B(z)^2)}{\mathrm dz}\overset{!}{=}\mu_0 g\frac{\rho}{\chi} \\\Delta(B^2)\approx2\mu_0 g\frac{\rho}{\chi}\Delta z $$ $$ B\sim\sqrt{z}\tag{2} $$ From equation $(1)$ we see that, interestingly, the requisite magnetic field profile does not depend on the mass of the object - only its density and magnetic susceptibility. It is well known that the density of a human being is roughly the same as water, at $1000\ \mathrm{kg/m^3}$, although for a more detailed analysis, we would consider bone, muscle and fat densities. It is a little harder to find an authoritative source on the value of the volume magnetic susceptibility, but we can again estimate it to be the same as that of water, around $-9.0\times10^{-6}$. These numbers would be fairly uniform across most living beings, setting the right hand side of $(1)$ to around $1400\ \mathrm{T^2/m}$. One might thus naïvely think that a similar setup to the frog levitating experiment using ${\sim}16\text{ T}$ should also be able to levitate humans (and indeed, most mammals/amphibians).

The only problem is the height of the field. From our approximation in $(2)$, we see that the necessary peak magnetic strength must vary roughly as the square root of the height of the field. Since a human being is much larger than a frog in all dimensions, one would need a larger setup sustaining such a gradient, which automatically means we would need a larger $B_0$. As an upright human is around 30 times as tall as the average experimental frog, the peak magnetic field strength would need to be around $16\sqrt{30}\approx88\text{ T}$, with a corresponding upper bound on the field gradient at equilibrium of $1400/88\approx16\ \mathrm{T/m}$.

One can mitigate this by having the subject lie down horizontally, but this would need a solenoid with a correspondingly larger radius which would in turn require more power to sustain the same field, draining one's already pitiful experimental funding.

Practical considerations

For a theorist, there are absolutely no ethical considerations. Practically however, the fragility of the human body is an annoyance: being exposed to magnetic fields above $8\text{ T}$ even for a short time is incredibly dangerous for humans. To the uninitiated, the Earth's magnetic field, sufficient to rotate compass needles, is around 50 microteslas and even a powerful MRI scanner only has a strength of $1.5\text{ T}$. Additionally, a magnetic field gradient that is too large may spaghettify the subject to various extents.

It would certainly be possible to circumvent this through clever engineering, but in our simplified version of a person levitating inside a solenoid, the constraints seem insurmountable: we need

1. an equilibrium at an inflection point
2. a basal field strength $B_0<8\text{ T}$
3. a magnetic field gradient of $1440/B_0>180\ \mathrm{T/m}$ at the equilibrium height
4. since even a horizontal human is an extended body, a large enough region of stability to allow a restoring force on the maximum and minimum vertical extents of the body, keeping it in equilibrium

The second condition implies the third, but conditions 3 and 4 are in opposition to each other: a sharp gradient obviously precludes a large stability region: a tiny shift from the equilibrium will spawn anharmonic oscillations, causing the body to fly unpredictably and spectacularly out of equilibrium. So we have a very obvious tradeoff between the safety of the subject (the peak magnetic field strength) and the efficacy of the setup (the height range for stability). The solenoidal system with $B_0=88\text{ T}$ is however very efficient, since a gradient of $16\ \mathrm{T/m}$ has a tall stable region that can fit a lying-down human, allowing the pressure above and below the body surfaces to act as the harmonic restoring force back towards the equilibrium point.

Disregarding the feasibility of this toy model, we must add that there is no "one" (peak) magnetic field strength that works - you can decrease the peak magnetic field strength at the price of proportionately increasing the gradient and hence decreasing the stability range (provided of course that a sizeable portion of the object still remains within it).

Here we have used extremely basic analysis which neither rigorously calculates the stable region, nor takes into account lateral stability (see Geim's original paper). Heuristically, determining the stable region amounts to finding the range of validity of the simple harmonic condition above via energy arguments.