1
$\begingroup$

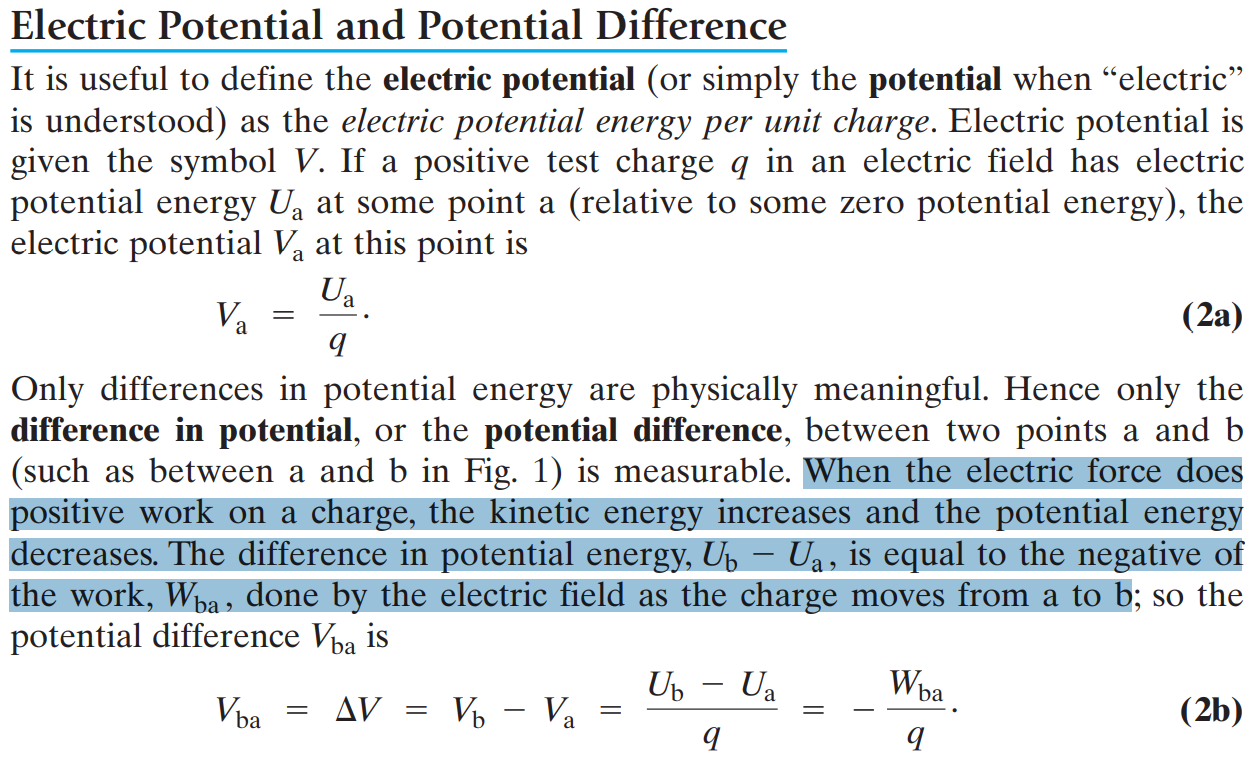
I'm studying electromagnetism and I'm a little confused on what this textbook says:

enter image description here

The highlighted part is what makes me confused. First thing, "The difference in potential energy is equal to the negative of the work done by the electric field", isn't work done by a force? How can work be done by an electric field?

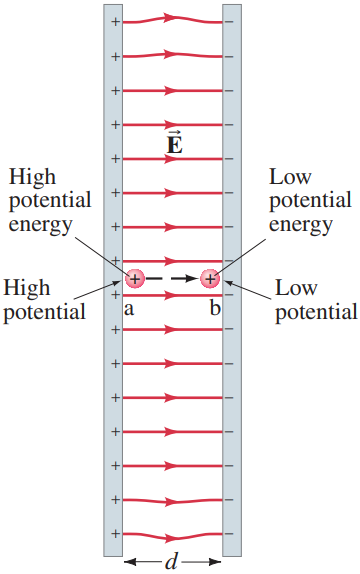
Second question, If I have two plates like this:

enter image description here

the work done by the electric force is positive since the force is in the same direction of the movement. If in the same two plates, I had a negative charge on the right side (negatively charged plate), the electric field would still go in the same direction, from positive to negative, but the electric force, being $\vec F=\vec E q$ would be in the opposite direction (since $q$ in this case is negative), and therefore this force would still do positive work again (same direction of the movement).

Therefore my question is, in the formula:
$V_{ba}=(U_b-U_a)/q=-W_{ba}/q$
"$-W_{ba}$" is the work done by what?

Could you help me out please?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

You can do work on a cart by pushing it. Actually, it is not you who does the work, but the force you create. You are still the initiator, though, so I'd argue that this use of terms still holds.

Similarly, sure it is the electric force which does work on the charge. But the electric field produces the force, so in a transitive way the electric field is the initiator.

To your last question, $W_{ba}$ is the work done by the electric field when moving the charge from point $a$ to $b$. If you add the negative sign $-W_{ba}$ as we see in the formula you show, then this is the work that must be done by you (by something external) in order to move the charge from point $a$ to $b$. Now, you don't have to do any work since the field does it for you, in fact you have to do less than no work meaning that you end up with excess energy (which is converted into kinetic energy of the charge).

If you instead asked for the work required to move the charge the opposite way, from $b$ to $a$, then you'd have to do positive work while the field simultaneously would do negative work against you.

Improve this answer
$\endgroup$
2
  • $\begingroup$ So they should have said: $U_b-U_a$ is equal to the NEGATIVE of the work $W_{ba}$ done by the electric field as the charge moves from a to b right? $\endgroup$
    – Andrea Burgio
    Commented Mar 16, 2021 at 19:21
  • $\begingroup$ @AndreaBurgio Yes... Isn't that also what the book says? $\endgroup$
    – Steeven
    Commented Mar 16, 2021 at 22:14
1
$\begingroup$

You can say the work done by the electric force or the work done by the electric field, it is a distinction that makes no difference.

It is appropriate to describe the work as being done by the field in either of your cases above because work is a transfer of energy and the electric field has energy. In both cases the energy of the field decreases and the energy of the charge increases.

It is also appropriate to describe the work as being done by the force in either of your cases above because work is force times displacement and the electric force exerts a force as the charge undergoes displacement. In both cases the direction of the force is the same as the direction of the displacement.

So say it whichever way you prefer.

Improve this answer
$\endgroup$
2
  • $\begingroup$ But how can the work done by the electric force = work done by the electric field? In the second case the electric field goes in one direction, from left to right, but the force goes in the opposite direction, from right to left. Therefore the electric force does positive work since it's in the same direction of the movement, and the electric field does negative work, since the movement is in the opposite direction isn't it? $\endgroup$
    – Andrea Burgio
    Commented Mar 12, 2021 at 15:12
  • $\begingroup$ That is not a relevant objection. The energy density of the electric field is proportional to the square of the field. In both cases the energy density decreases so energy goes from the field to the matter. This transfer of energy is work. The electric field does NOT do negative work in the second case. For forces work is $F\cdot d$. For fields work is $\Delta U$, not $E\cdot d$. $\endgroup$
    – Dale
    Commented Mar 12, 2021 at 16:11

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.