Why is electric field across a resistor constant, or voltage gradient linear?

Question

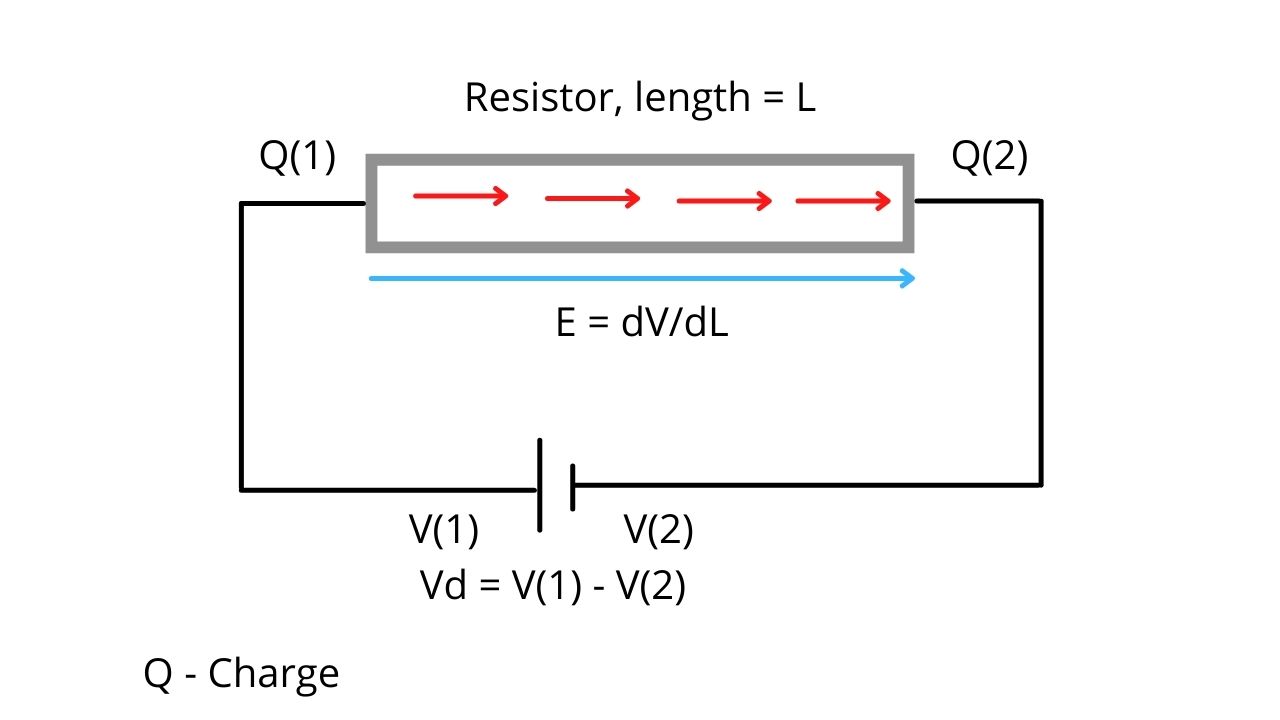
Consider this complex circuit :

1. Consider an ideal resistor with uniform cross section and made of isotropic material with constant resistivity.

2. Consider an ideal battery giving a voltage difference of $V$ across terminal.

3. Consider that the wires are superconductors with $0$ resistance.

4. The voltage drop across the length of current flow is linear, that is $\frac{\partial V}{\partial L}$ is constant.

5. This implies that Electrostatic force in the direction of current flow is constant throughout the resistor, since $\vec E = \frac{\partial V}{\partial L}$.

** Q: Is there a way to prove that the Electric Field ($\vec E$) is constant all along the length of the resistor in the direction of current flow, derive this mathematically using fundamental laws of electrostatics, or prove why the $\frac{\partial V}{\partial L}$ is linear across the length of the wire in the direction of the current flow?

How do the charges in the wire (at the edges of the resistor) exert a constant electrostatic force/electric field throughout the resistor?

---

**Note:

1. Most other texts give the analogy of a capacitor with 2 charged plates, but I feel those examples are wrong, since they assume plates of infinite surface area which is clearly not analogous to the above circuit.

2. Don't tell $E = \frac{V}{L}$ is constant at all points assuming $V = IR$ (ohm's law), because its actually the other way. $V=IR$ is probably derived from $E = \frac{V}{L}$ being constant.

Answer 1

Let's assume the following:

• The terminals (i.e., the points where the wires make contact with the resistive material) are plates having the same shape as the cross-section of the resistive material.
• The material obeys the microscopic version of Ohm's Law, $\vec{J} = \sigma \vec{E}$, and has uniform conductivity.
• We are in steady state, i.e., all quantities are constant with respect to time.

Then it can be proven as follows. (My argument is adapted from Chapter 7 of Griffiths's Electrodynamics.)

1. The potentials on the plates are $V_1$ and $V_2$.
2. At the boundary of the resistive material, we must have $\vec{J} \cdot \hat{n} = 0$, where $\hat{n}$ is an outward-pointing normal. If we didn't, then either charge would be building up on the surface or escaping the resistive material.
3. Given Ohm's Law, this implies that $\vec{E} \cdot \hat{n} = 0$ on the surface of the resistive material.
4. Since $\sigma$ is constant, and $\vec{J} = \sigma \vec{E}$, the steady-state current condition $\vec{\nabla} \cdot \vec{J} = 0$ implies that $\vec{\nabla} \cdot \vec{E} = - \nabla^2 V = 0$.
5. Thus, the potential inside the resistive material satisfies Laplace's equation $\nabla^2 V = 0$, with boundary conditions $V = V_1$ at one terminal, $V = V_2$ at the other terminal, and $\hat{n} \cdot \vec{\nabla} V = 0$ along the sides.
6. A solution to this potential problem inside the volume of interest is that the potential has a linear gradient parallel to the axis of the resistive material. By uniqueness of solutions to Laplace's equation, this is the only possibility.

Note that strictly speaking, the condition for uniform conductivity (used in step 4) could be relaxed to allow for situations where $\vec{E} \cdot \vec{\nabla} \sigma = 0$, and the result would still hold. For example, one could imagine a cylindrical object where $\sigma$ is a function of the distance from the axis, but is still translationally invariant along the axis.

Answer 2

As to your last question: There will be an excess of electrons on the negative end of the resistor, and a deficiency of electrons at the positive end. A uniform field inside requires a gradient in the charge density.

Answer 3

You said:

"Don't tell $E = V/L$ is constant at all points assuming $V = IR$ (ohm's law), because its actually the other way. $V=IR$ is probably derived from $E = V/L$ being constant."

But this is the right answer. We have $V = IR$ first, then we derive "$E = V/L$ is constant at all points (of the resistor)". Why does $V = IR$? Well it is from your assumption "Consider an ideal resistor with uniform cross section and made of isotropic material with constant resistivity." We recall that resistance is defined with $V=IR$ thus this is the answer.