Origin of probabilities in Quantum Mechanics?

Question

The non-normalized wavefunction of a general qubit is given by: $$|\psi\rangle=A|0\rangle+B|1\rangle.$$ The complex amplitudes $A$ and $B$ can be represented by two arrows in the complex plane:

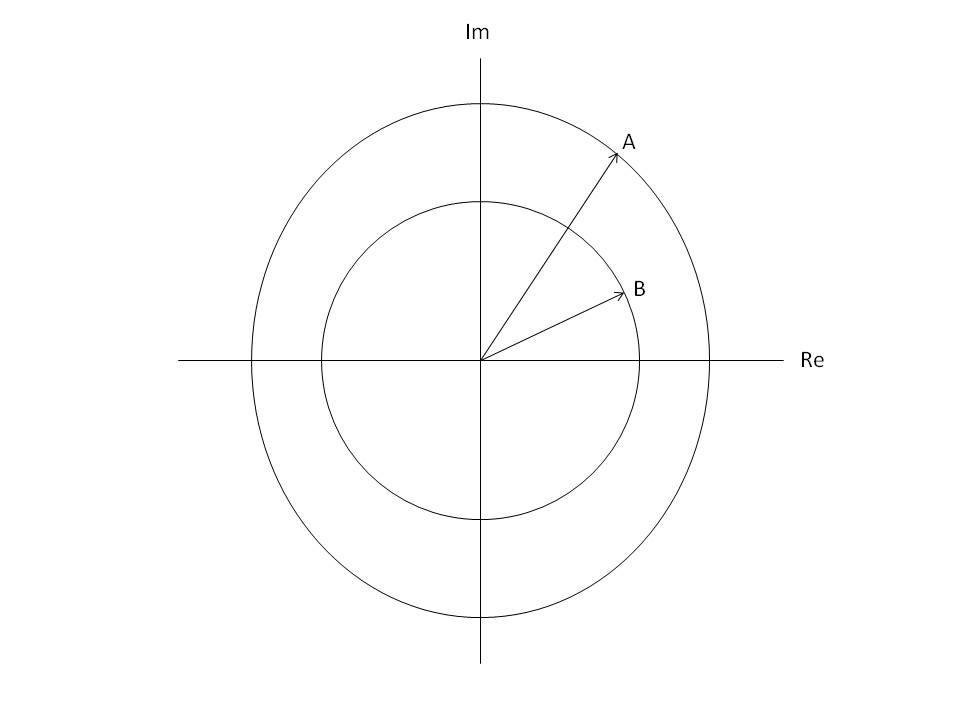
Now the wavefunction can be multiplied by any complex number $R$ without changing the physics. This will cause the arrows $A$ and $B$ to rotate and shrink/expand together with a fixed angle between them.

Therefore two sets of points will be traced out represented by a circle with area $|A|^2$ and a circle with area $|B|^2$. These represent the sets of possible values for the amplitudes $A$ and $B$.

Thus if we become entangled with the qubit then the probabilities of finding ourselves in set $A$ (measuring $0$) or set $B$ (measuring $1$) are given by: $$P(0)=\frac{|A|^2}{|A|^2+|B|^2}$$ $$P(1)=\frac{|B|^2}{|A|^2+|B|^2}.$$

Does this picture help to understand the origin of probabilities in quantum mechanics?

Correction

Let $$A=R_Ae^{i\theta_A}$$ $$B=R_Be^{i\theta_B}$$ A general normalized wavefunction is given by: $$|\psi\rangle=\frac{1}{(R_A^2+R_B^2)^{1/2}}\large[R_Ae^{i\theta_A}+R_Be^{i\theta_B}\large]$$ Assume that I multiply the amplitudes $A$ and $B$ by $$C=Re^{i\theta}$$ Then the normalized wavefunction becomes $$|\psi\rangle=\frac{1}{R(R_A^2+R_B^2)^{1/2}}\large[RR_Ae^{i(\theta_A+\theta)}+RR_Be^{i(\theta_B+\theta)}\large]$$ $$|\psi\rangle=\frac{e^{i\theta}}{(R_A^2+R_B^2)^{1/2}}\large[R_Ae^{i\theta_A}+R_Be^{i\theta_B}\large]$$ It seems that the only degree of freedom is a phase angle $\theta$ rather than an area as I asserted above.

Answer 1

So that picture is a way to understand quantum mechanics, and in fact it's a way that Feynman used to explain it to non-technical people, which you can see in his New Zealand lectures that were videotaped, and they became the book QED: The Strange Theory of Light and Matter.

However it does not shed much light on the origins of amplitudes since that is just an axiom of how the theory works. Like you still have an abstract imaginary circle and there is no real reason to connect its area with any sort of probability, and you have not motivated how these different amplitudes can be added or multiplied in that explanation.

Just to give you what a more general argument might look like, Scott Aaronson’s paper “Is Quantum Mechanics an Island in Theory Space?” argues that there are kind of only two possibilities, two probabilistic theories, one without negative probabilities that we call classical probability, and one with destructive interference which we call quantum mechanics, so that if you take it for granted that quantum systems must have destructive interference and results of certain quantum experiments cannot be known in advance (see quantum crypto for a usage of the latter) then the only ways to describe this must use complex numbers as amplitudes.

Stuff like that. It doesn't have to be that exact argument but it does need to have that sweeping character. So for example another argument could take as its starting point the 2-spinor calculus that underlies special relativity, and maybe amplitudes are the only thing which “plays nice” with those 2-spinors, something that connects QM to other phenomena in the world maybe. But it's never going to be as simple as “just look at these circles” because you have drawn those circles in an imaginary mathematical idealization universe, and the problem for physics is how do we model things in our universe with things in such a mathematical idealization, and so there is always a translation step. So the explanation has to be starting from things in our universe, if that makes sense, and how those experiments set hard limits on what can be used to model them in the universe of ideas in your head.