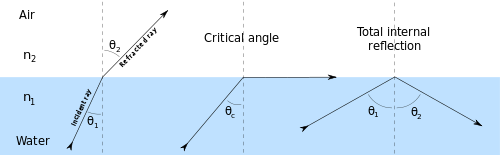
When light reaches a boundary between materials below the critical angle, some of it refracts and some of it reflects. For example, glass acts as a partial mirror with a dark background.
Assuming no light is lost in the process, how much of total light in the incident ray is refracted as a function of $\theta_1$ (but of course $\theta_c ,n_1,n_2$ and other properties of the materials will probably matter too (whether the reflection was hard or soft, for instance)).
Intuitively, the amount refracted seems to be maximum when $\theta=0$, so perhaps $A\cos(\pi\frac{\theta}{\theta_c})$ is a viable function for the fraction refracted when $\theta > \theta_c$.
I hope there's an explanation that doesn't explain this away with a single experimental constant associated with each material (that is, is theoretically derived).
