For resonance to occur, is it true that the force lags behind the motion by $\pi/2$? I saw some notes written that the motion lags behind the force by $\pi/2$ which makes no sense to me. As I watched many videos and I worked out the motion, it always happens before the force pushes. E.g. if the force is $F=\cos\omega t$ and $x = A\cos(\omega t -\pi/2)$, is it true that force lags behind the motion?
If it's $\pi/2$ , does there happen anything special?
Also, I realised that if there is no damping, we can only get 180 or 0 phase difference, which is quite counterintuitive to me. Can anyone give any example to help me feel better?

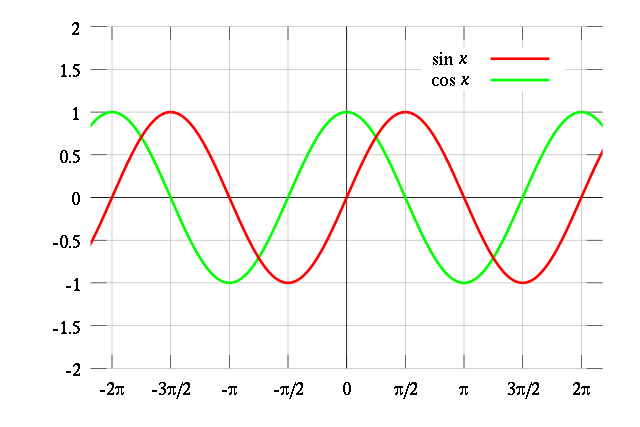 Imagine the red line being the amplitude of the swing, and the green line is your push strength.
Imagine the red line being the amplitude of the swing, and the green line is your push strength.