Problem regarding components of forces in conical pendulum

Question

For the motion of conical pendulum we can write equations as

$$T_{F}\cos\theta =mg$$

$$T_{F}\sin\theta=\frac{mv^2}{R}$$

$T_{F}$ represents tension in the string.

$v$ is the velocity of bob at this instant.

$R$ is the radius of circle.

But if we split $mg$ into its components,we can write

$$T_{F}=mg\cos\theta$$(because length of the string is constant)

What does then $mg \sin\theta$ will do? Also $mg \sin\theta$ is not in plane of circle. So what causes centripetal acceleration if I split components like this?

Answer 1

I'm asking for reason why you can split Tension(first case) but not mg(second case)

When you say 'splitting,' you really mean projecting a force along some axis.

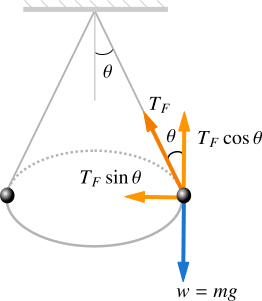
First let's cover why you 'split' the tension as you did in your first approach.

You know that the net force in the y-direction is zero; finding the y-component of tension helps you solve the problem. That's why you bother to 'split.'

Q. Why can't I split the force of gravity?

A. Of course you can! The question, is, however, along what axes?

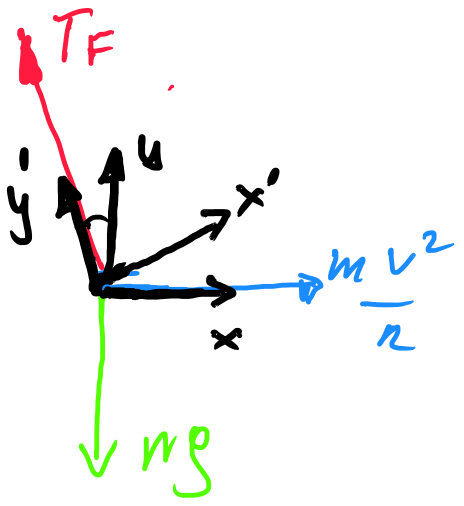
From what I understood, you decided to calculate the component of gravity force that lies on the same axis as the tension force (or the string). There is nothing wrong with that. However, the true equation you'd get would be:

$$0 = T - mg\cos\theta + F_{\rm fictitious}$$

The reference frame you chose is non inertial. Though there is no motion along the string, the string itself is accelerating. Thus, there will be what we can call a fictitious force.

The whole reason why we 'split' along x- and y-axis in this problem is because the frame is inertial, so we can solve for the tension.

Answer 2

you have three forces that must be in equilibrium, these is independent of the coordinate system that you chosen

x-y system

$$\sum F_x={\frac {m{v}^{2}}{R}}-T_{{F}}\sin \left( \theta \right)= 0$$ $$\sum F_y=-mg+T_{{F}}\cos \left( \theta \right)=0 $$

x'-y' system

$$\sum F_x'={\frac {m{v}^{2}\cos \left( \theta \right) }{R}}-\sin \left( \theta \right) mg =0$$ $$\sum F_y'=-{\frac {\sin \left( \theta \right) m{v}^{2}}{R}}-\cos \left( \theta \right) mg+T_{{F}} =0$$

in both coordinate system you obtain the same solutions

$$T_F=\frac{m\,g}{\cos(\theta)}$$ $$v=\sqrt{g\,r\,\tan(\theta)}$$

thus the choice of the coordinate system can't affected the results.

I am not sure that this answer your question?

Answer 3

I don't think you can write $T_F = mgcos \theta$, since in the first line, you've clearly (and correctly) have written $T_F cos \theta = mg$. To be more intuitive, imagine a vector in direction of $T_F$, which is a component of $mg$. For the rope's length to remain constant, this vector's magnitude should be equal to $T_F$, only in the opposite direction of $T_F$. From the image of the question, you can see that this vector's length is clearly longer than $mg$, so this must be equal to $mg/ cos \theta$ and not $mg cos \theta$.