1
$\begingroup$

I know the title looks vague and the concept also looked strange to me when I read this.

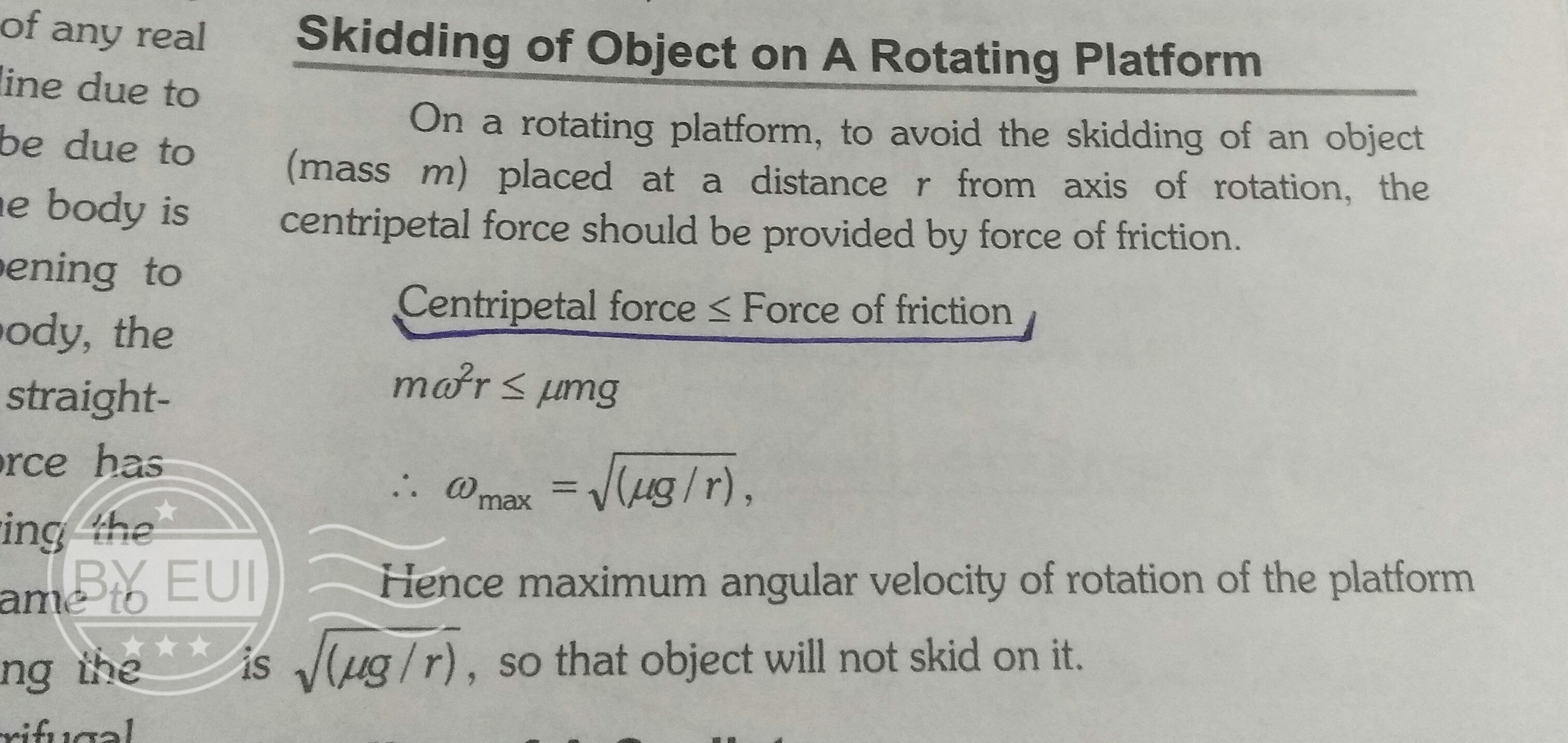
enter image description here

In the image, it is pretty clearly written that to avoid skiding friction force serves as the centripetal force. But just a line below, the equation says :

$$Centripetal\; \;force≤Force \; \; of \; \;friction $$

I don't know if it is true but I don't think it is true. If friction is the only horizontal force here then why should it be smaller than centripetal force ?

Correct me if I am wrong somewhere.

Improve this question
$\endgroup$
9
  • $\begingroup$ static friction can't exceed the required centripetal force. This is because static friction is always equal to what it needs to be to prevent relative slipping. This is analogous to asking if static friction could exceed mgsinx on a particle placed on a slope $\endgroup$
    – Vulgar Mechanick
    Commented Jan 8, 2021 at 16:16
  • $\begingroup$ @OVERWOOTCH but what is the required centripetal force ? $\endgroup$
    – Ankit
    Commented Jan 8, 2021 at 16:31
  • 1
    $\begingroup$ The book is wrong! Phrase on the right side should be "maximum possible static friction." Lesson to learn: the book isn't always right. What book is that? $\endgroup$
    – Bill N
    Commented Jan 8, 2021 at 20:28
  • 1
    $\begingroup$ @Bill N you will be surprised to hear that the name of the book is errorless physics :) $\endgroup$
    – Ankit
    Commented Jan 9, 2021 at 4:05
  • 2
    $\begingroup$ @Ankit So that's another error! :) $\endgroup$
    – Bill N
    Commented Jan 9, 2021 at 14:02

6 Answers 6

Reset to default
3
$\begingroup$

I think what the book means is "centripetal force $\leq$ maximum force of friction".

Think of the simple case of a block on a slightly inclined plane: the maximum force that friction can exert is $\mu F_\perp$, where $F_\perp$ is the component of the block's weight that is perpendicular to the plane; if this maximum force is bigger than the parallel component of the weight (which is trying to make the block slide), then friction will only exert a force equal to the parallel weight, and no more, otherwise the block would start climbing upwards.

So what I think your book means is that, in order not to have skidding, the centripetal force required should be smaller than the maximum force of friction $\mu F_\perp$.

Improve this answer
$\endgroup$
1
$\begingroup$

Centripetal force is the name given to "a force that acts on a body moving in a circular path and is directed toward the center around which the body is moving". There is no "basic" centripetal force; it is just the name given to a true force- such as gravity or friction- that acts as described in the first sentence. As other answers state, the force of friction must not be exceeded for that force to be a centripetal force.

(A centrifugal force is a fictitious force that appears in a non-inertial rotating coordinate system.)

Improve this answer
$\endgroup$
2
  • $\begingroup$ Good answer. The naming is unfortunate and misleading. In my classes I prefer to talk about centripetal acceleration being $a_c = v^2/r$ or $\omega^2 r$ and Newton's 2nd law for radial forces becomes $\Sigma F_r = ma_c$. That way there is a degree of separation between the forces and the adjective, centripetal. $\endgroup$
    – Bill N
    Commented Jan 9, 2021 at 14:59
  • $\begingroup$ @Bill N Good way to clarify "centripetal". Thanks. $\endgroup$
    – John Darby
    Commented Jan 9, 2021 at 15:46
0
$\begingroup$

Well, I Don't think it's an error, it is just terribly phrased.

It's more like the maximum centripetal force on the object will be the maximum value of static friction.

For angular velocities lower than the max, friction will adjust accordingly.

Improve this answer
$\endgroup$
1
  • $\begingroup$ I beg to differ. It's just wrong. The centripetal force is exactly equal to the force of friction. The OP's clever subject line speaks to this. $\endgroup$
    – garyp
    Commented May 26, 2021 at 10:33
0
$\begingroup$

Static friction will adjust itself (up to a maximum) to whatever value is required to prevent slipping.

Improve this answer
$\endgroup$
0
$\begingroup$

Talking about skidding. You can take circular turning into consideration where you are driving your car on circular road. Friction acts towards the centre and so does , centripetal acceleration.

Centripetal force = $\frac{mv^2}{r}$. I hope you know this. If not , then just comment.

Friction always acts in the direction opposite to direction where it can slide. For this situation , you can say that your frictional force = $\frac{mv^2}{r}$. Since Friction force = m*a where a = $\frac{v^2}{r}$.

This force is also equal to μN = μMg.

So , it is just that they have written the same thing.

The book states that frictional force $\ge$ centripetal force. You have to make sure for is static or limiting friction. Centripetal force should be less than or equal to this. Otherwise , kinetic friction may occur and then , sliding may happen.

So , I hope you know now what happens if centripetal force is greater than the force itself or frictional force.

Do let me know if you have any difficulty :)

Improve this answer
$\endgroup$
-1
$\begingroup$

Friction is reactive force, it is always same as a force that is oposing it. There is of course maximum frictional force and centrifugal force can exceed this so then you have some sliding. Since it has a role of centripetal force in this example these are same forces so to me too this seems strange. That equation up there would make more sense if it compared friction with centrifugal force with condition being that centrifugal must be less than maximal friction.

Improve this answer
$\endgroup$
8
  • $\begingroup$ No, friction is a force separate from other forces acting on an object. Consider someone pulling on a rope attached to a box, sliding across the floor. To start the box, the rope tension must be greater than the friction. If the person stops pulling, the friction stops the box. According to your answer, the box would continue to slide because the friction would go to zero. $\endgroup$
    – Bill N
    Commented Jan 8, 2021 at 20:32
  • $\begingroup$ If you stop pulling friction is still acting but when body fully stops friction is zero. $\endgroup$
    – Žarko Tomičić
    Commented Jan 8, 2021 at 22:32
  • $\begingroup$ That's not what you say in the first sentence. You say "it is always same as a force that is oposing[sic] it." Not true. $\endgroup$
    – Bill N
    Commented Jan 8, 2021 at 23:09
  • $\begingroup$ Well if a body is moving it is acting with aa force against orregular surface, so I would still say that it is a reactive force, much like normal force of the surface. $\endgroup$
    – Žarko Tomičić
    Commented Jan 9, 2021 at 7:29
  • $\begingroup$ Because, if we think of friction as a result of electric forces betwen bodies, than, when one body is close enough, interaction starts. If one body slams into another, they are interacting. $\endgroup$
    – Žarko Tomičić
    Commented Jan 9, 2021 at 7:46

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.