Polarization by reflection - Brewster's angle

Question

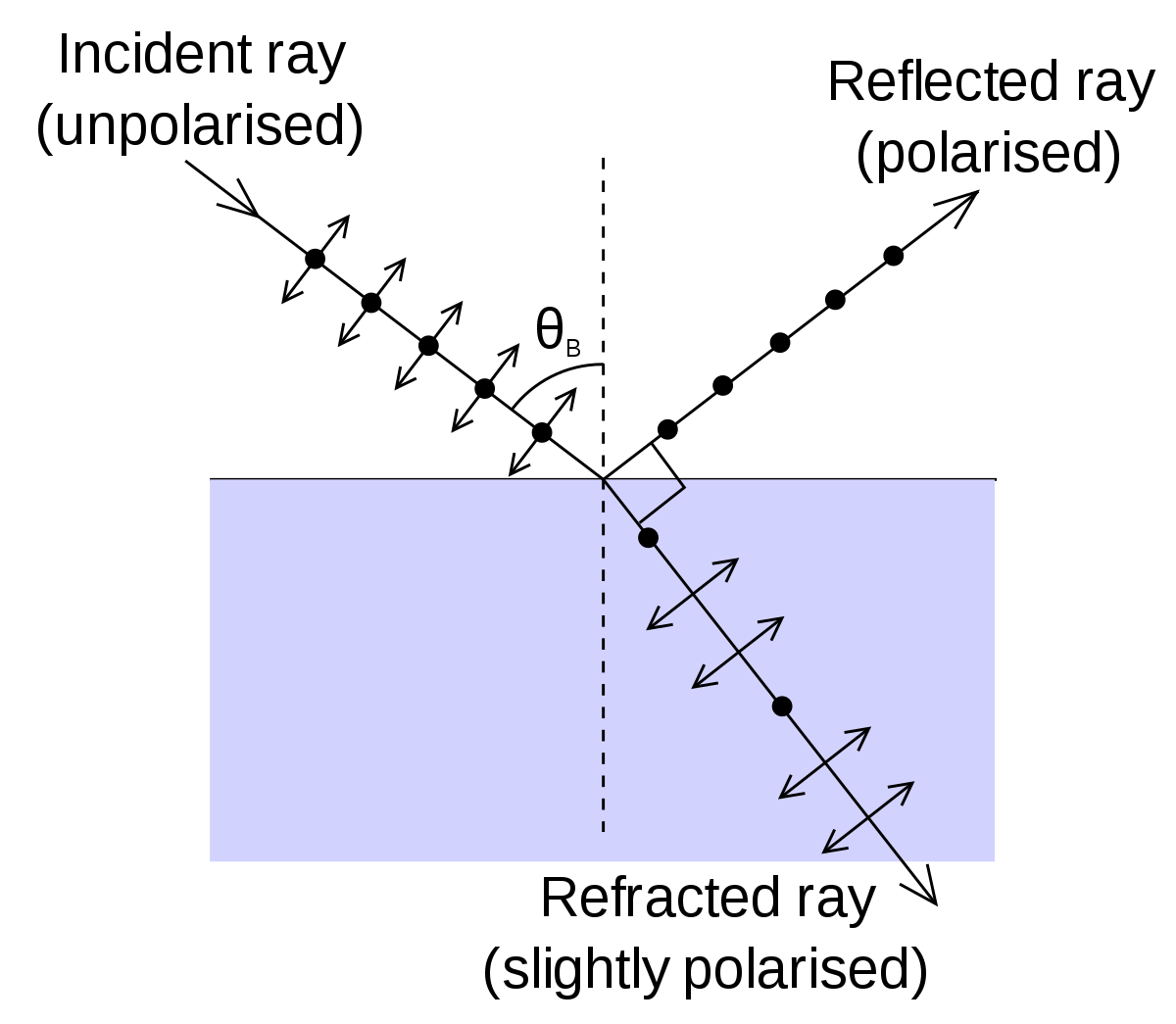
When incident light hits an interface of a dielectric surface at the Brewster angle, I understand that it will result in the generation of a perfectly polarized(horizontally) reflective ray and a partially polarized refracted ray. I do not understand, however, what happens at the interface that causes the reflective ray to become horizontally polarized.

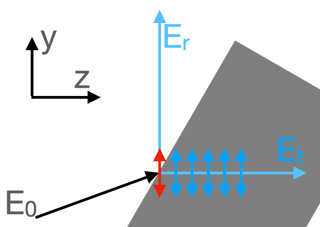
I know the basic principle that it is the oscillation of charged particles that creates the reflected beam. However, I do not understand how the "light" reemitted by these electrons results in the formation of refracted and reflected rays. I have read in several places that the reflected wave doesn't have electric field vectors parallel to the refracted wave. I am hoping that someone can elucidate what is meant by this and why this occurs.

Also, it is my understanding that the dots in the diagram represent oscillations that are "into" the page, while the arrows are parallel.

Answer 1

Let's take two parts and put them together:

1. If light is propagating along the $z$ direction, its wave vector $\vec k$ is parallel to $\vec e_z$.
2. The direction of the electric field $\vec E$ is perpendicular to $\vec k$. Thus, if $\vec k = k \vec e_z$, than $$ \vec E = E_x \vec e_x + E_y \vec e_y $$

Now let's consider an incident field $\vec E_0 %= E_x\vec e_x + E_y \vec e_y + E_z \vec e_z $, which is reflected from a substrate in the Brewster configuration. What the incident field does is to excite the electrons inside the substrate. However, the excitation is done in such a way that the oscillation of the electrons is perpendicular to the the transmitted wave vector $\vec k_t$. Since we are free to choose our coordinate system, we choose $\vec k_t = k_t \vec e_z$. However, this implies that electrons do not oscillate in the $z$ direction. Hence, neither the transmitted nor the reflected beam have a $E_z$ field component.

Now, in the Brewster configuration the reflected and the transmitted wave vector are perpendicular to each another. Hence, the reflected wave vector $\vec k_r$ is parallel to $\vec e_y$. Thus, using the fact that the electric field must be perpendicular to the wave vector, we conclude that also the $y$ component of the reflected electric field must be zero. Hence, the reflected electric field if polarised in $x$ direction.