6
$\begingroup$

Let us assume a perfectly ideal fluid in a narrow enough pipe (as to ignore the pressure in the fluid due to its height) kept on a horizontal level.

We take the pressure on both ends of the pipe same, so the liquid does not flow on its own. Initially, we provide it a force so that it starts moving with a constant velocity then we remove the force.

Now suppose the pipe gets thinner from a point.

Scenario 1

Let us assume the pipe was empty initially and then we fill it with fluid up to a mark (before the pipe gets thinner) and we apply a force on it, the fluid gains some velocity, and then we stop the force ( before it reaches thinner part).

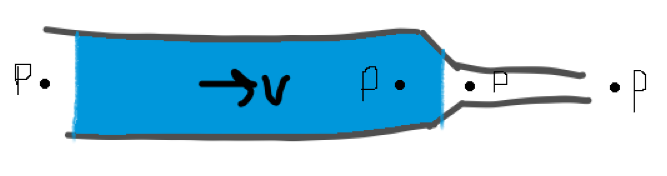
Now the fluid will flow with constant velocity and the pressure in the thin part and thick part will be the same(as pressure outside is also the same and the tube is narrow) Now when it encounters the thinner part what will happen? Will its velocity increase and if it does what is the cause(as the pressure difference is 0) or will it stop and why?

Scenario 2

We had the pipe initially fully filled with the fluid, now we apply a force which creates an external pressure and the fluid starts flowing like it does normally as there is a pressure difference. 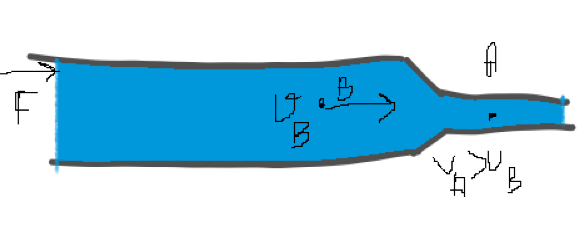enter image description here

Now we stop applying the force. (before the end of the liquid reaches the thinner part) Now, the pressure difference will disappear. (as now again the pressure on both sides becomes same and for the same cross-sectional area of the tube the pressure along the horizontal line is same and the tube is narrow) What will happen? Will the fluid stop flowing? If it does what will cause it? If it doesn't then what will happen at the thinner part? What will happen to the velocity in the thicker part and thinner parts?

Will the flow be streamlined when the fluid flows through the junction to thinner part in both the scenarios?

My thoughts Scenario 1: I think that the velocity will not increase as there is no difference in pressure at the junction which will provide it a force. But then if the liquid continues through the thinner part and velocity is the same then won't the equation of continuity be violated? Scenario 2: I'm clueless about this one.

Improve this question
$\endgroup$
5
  • $\begingroup$ You are aware that Bernoulli’s equation only applies to steady state flows, right? $\endgroup$
    – Chet Miller
    Commented Dec 10, 2020 at 17:11
  • $\begingroup$ @ChetMiller, yes but the equation of continuity must follow i think. If you are talking about the addon question, it has nothing to do with the original question. $\endgroup$
    – alphagamma
    Commented Dec 11, 2020 at 9:26
  • 1
    $\begingroup$ Please delete the add on. It should be a separate question $\endgroup$
    – Dale
    Commented Dec 11, 2020 at 12:27
  • $\begingroup$ As I said, the usual form of the Bernoulli equation applies only yo steady flows, and not to flows where the velocity is changing with time as reckoned by an observer in the rest frame of the flow channel. So the pressure at the two ends do not have to be equal. $\endgroup$
    – Chet Miller
    Commented Dec 11, 2020 at 15:12
  • 1
    $\begingroup$ @Dale , sure, i have deleted it $\endgroup$
    – alphagamma
    Commented Dec 12, 2020 at 4:16

1 Answer 1

Reset to default
1
$\begingroup$

Both explained if inertia is added.

Case 1, real world example:

https://en.wikipedia.org/wiki/Hydraulic_rams

It works by utilizing fluid's inertia in a pipe. Inertia acts as a very large force when anything, like a fluid reaching a narrow point, tries to stop the fluid. Fluid will accelerate in the narrowing. Fluid will excert very large pressure on the whole pipe. This can rupture the pipe and narrowing.

Case 2: its simpler, but similar to case 1. It is a steady state process. Water accelerates in the narrowing, as a continuous process. Until energy stored as fluid inertia is dissipated on friction and acceleration of water in the narrowing, like in the first case. But in this case it is a smooth process, pressure peak is not any larger than during the initial force application. Force will gradually decrease as inertia exchaustes the energy provided.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Thanks, but what will accelerate the fluid in the narrowing? $\endgroup$
    – alphagamma
    Commented Dec 12, 2020 at 6:09
  • $\begingroup$ Inertia. Water tries to move and push the water in front. Moving object stores energy. If you try to stop a moving object, you get the energy out. This is how flywheel works. The water in the big pipe pushes the water in the narrowing. $\endgroup$
    – Surprised Seagull
    Commented Dec 12, 2020 at 6:34
  • $\begingroup$ The flow of water when it gets into the junction and thinner part won't be streamlined, will it? $\endgroup$
    – alphagamma
    Commented Dec 13, 2020 at 12:13
  • $\begingroup$ Streamlined can be the pipe. It is not streamined on images. When water flows through non-streamlined part, it will make a stremline path with maximum speed. The edges and corners will have less speed. If flow is laminar, then slow parts will remain directed as the main flow. If flow is turbulent, then slow parts will form vortexes. en.wikipedia.org/wiki/Reynolds_number if Re<1000 slow parts will likely be smooth. if Re>10000 slow parts will likely consist of vortexes. $\endgroup$
    – Surprised Seagull
    Commented Dec 13, 2020 at 12:21

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.