$\beta$ decay paths question

Question

Can the $\beta^-$ decay proceed by the absorption of a $W^{+}$ boson or the $\beta^+$ by the absorption of a $W^-$ boson?

The $\beta^-$ decay is known as the decay of a $d$ quark into an $u$ quark and a 'virtual' $W^{-}$ boson, which then decays further into an electron and an electron anti neutrino. Similarly, in the $\beta^+$, the $W^+$ boson emitted by the $u$ quark produces a positron $e^+$ and an electron neutrino $\nu_e$.

However the muon decay: $\mu^-\to e^{-}+\nu_{\mu}+\overline \nu_e$ can occur either (1) by the decay of the muon to a muon neutrino and a $W^{-}$ boson, which decays then into an electron and an electron antineutrino: 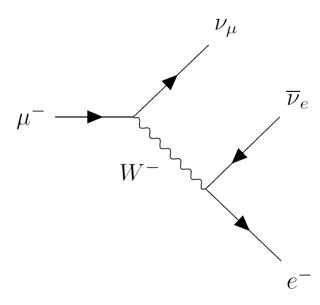or (2) by the production of a pair of $e^{-}$, $\overline \nu_e$ and a $W^{+}$ boson which changes the $\mu^-$ into the $\nu_{\mu}$:

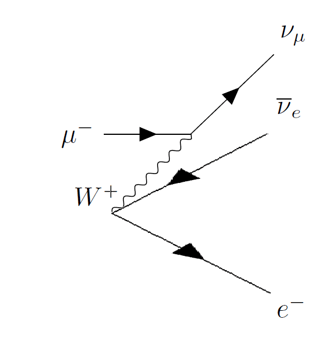
Can the same assumption, that there are two different possible paths (either with a $W^-$ or a $W^+$ boson as an intermediate), be made for the $\beta^-$ and $\beta^+$ decay?

Answer 1

Yes, the time-ordering of the vertices can have the $W$ field excited when the $\mu$ decays and de-excited when the $e$ and $\nu_{e}$ pair are created, or vice versa. However, as a matter of terminology and calculation, these two time orderings do not correspond to different Feynman diagrams. There is no time ordering in the placement of the vertices in a Feynman diagram; this one thing distinguishes “Feynman” diagrams from other types of interaction diagrams that may be used in particle physics. Consequently, the first Feynman diagram shown in the question—when it is converted into a formula for the matrix element—actually already encompasses both possible time orders for the $\mu$ decay process.

Answer 2

If I understand your question, the difference in the processes you are considering come from the fact that electrons are the lightest particles in their class. Muons can decay into electrons but electrons do not decay naturally to anything, they are stable. They have less routes for decays. I hope that helps.