0
$\begingroup$

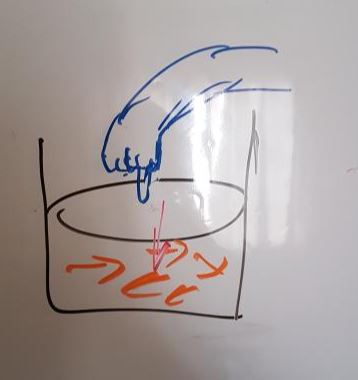
Suppose there is a piston as shown below,

enter image description here

And, let's say that I press down on the piston using one finger, this compresses the gas inside.

Now, in usual thermodynamic textbooks, we say that the force exerted by piston on gas is equal to the pressure of the piston on the gas times area of the piston but, here, I have applied the force on a small area only. How exactly did the pressure from my finger transmit for the previous statement to be true? I Find it strange that the whole force exerted at a small area and the other side the same force comes over a large area, does this mean pressure on each side is different? How would we work this out from the first principles about pressure?

Color key:

Orange is the gas molecules with different velocities moving about in the container

Red arrow denotes direction of gas

Note: the main point I wish to understand how the pressure is transmitted, because on one side it is concentrated at other point, on the other the whole face pushes down the gas.

Improve this question
$\endgroup$
6
  • 2
    $\begingroup$ No matter how small is the area you apply the external force, if the two forces (gas and external one) are balanced and the piston is a rigid body, the system will be at equilibrium. Of course if you use a small area like the tip of a finger the pressure there will be pretty high and could cause pain. $\endgroup$
    – Landau
    Commented Sep 5, 2020 at 9:49
  • $\begingroup$ @Landau This is an answer. Why not post it? $\endgroup$
    – Bob D
    Commented Sep 5, 2020 at 12:35
  • $\begingroup$ My question was more about how we can say that force is pressure times area of plate , when in this case I've concentrated everything into a point $\endgroup$
    – Brian
    Commented Sep 5, 2020 at 12:36
  • $\begingroup$ Like it is often said "area over which force is applied", but like you apply force at points on the body, no? $\endgroup$
    – Brian
    Commented Sep 5, 2020 at 12:37
  • $\begingroup$ I have posted a possible answer @BobD $\endgroup$
    – Landau
    Commented Sep 5, 2020 at 16:02

2 Answers 2

Reset to default
2
$\begingroup$

First of all, you consider the piston (mass $m_{p}$) as a rigid body which is constrained to move inside the cylinder. Let's call $S$ the surface of the piston. Now you put above the piston, on its center, a cylindrical weight with mass $m$ and surface $S_{w}$. At the equilibrium (when the piston is firm), the force equation on the vertical axe, gives you: $$(m_{p}+m)g + P_{0} S=P_{gas}S$$ Where $P_{gas}$ is the pressure of the gas inside the cylinder at the equilibrium (you can measure it directly) and $P_{0}$ is the atmospheric pressure. $P_{gas}$ acts on every point of the low side of the piston. While on the top side, acts the atmospheric pressure and under the weight, but only there, the pressure is: $$P_{w}=\frac{mg}{S_{w}}+ P_{0}$$ For example, to understand the magnitudes, if you consider $m=10kg$, you have: $$P_{w}^{(bar)}≈\frac{10^{3}}{S_{w}^{(mm^{2})}}+ 1$$ Which is the function rapresented below: enter image description here

So if $S_{w}=10mm^{2}$ the pressure on those points is about $100bar$ (extremely high).

Improve this answer
$\endgroup$
1
  • $\begingroup$ So only in that part would the bottom face of the piston ( one in contact with gas) exert a force? $\endgroup$
    – Brian
    Commented Sep 13, 2020 at 11:14
1
$\begingroup$

The piston will flex or temporarily deform slightly so as to distribute the force applied by your finger across its whole horizontal area. If the piston is not strong enough or rigid enough to do this then it will permanently deform (imagine hammering a nail into wood) or break (imagine hammering a nail into a sheet of glass).

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.