Why does light not propagate backward in dense media?

Question

It is easy to see how light propagating in a dense medium destructively interferes laterally and constructively interferes in the forward direction. This is why light will travel forward in such a medium but wont scatter laterally. This phenomenon is usually shown using a diagram like this one

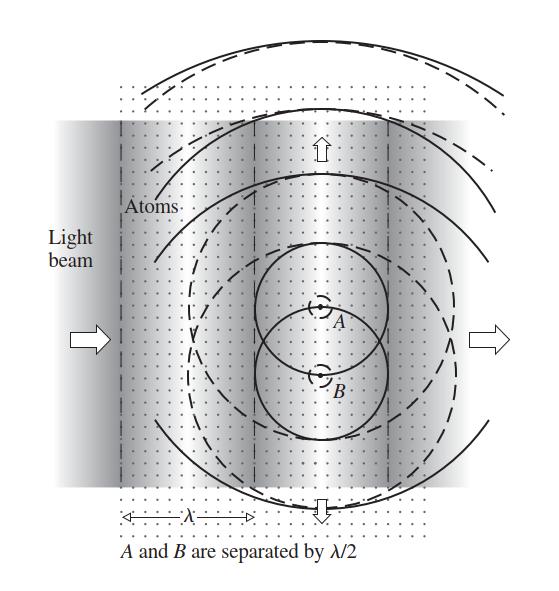
or this one

However in both cases light appears to be constructively interfering in the backward direction as well as the forward direction. This would also make sense taking the conservation of energy into consideration. The incoming radiation incident on a particular layer of atoms constructively interferes going forward causing an increase in forward intensity. This increase in forward intensity must be offset by a decrease in lateral intensity on the one side (say the right side) . The backscattering interferes constructively leading to an increase in backward intensity. This increase in intensity is offset by a decrease in intensity on the other side (say the left side this time).

This must however be wrong though since light travelling through thick glass does not appreciably back-scatter compared to the forward propagation. So how does destructive interference take place in the backward direction when the diagrams I have shown seem to indicate that it should be constructively interfering in that direction?

Any help on this would be most appreciated!

Answer 1

From Huygen's principle, light is scattered as a spherical wave from each scatterer (assuming a scalar wave so we ignore polarisation effects as these modify the strength of scattering). The backward scattered portion of the wave suffers a phase shift on propagation and so reaches a previous scattering point with a phase different from the light being scattered there. Thus there is a phase difference between the back scattered components from one scatterer and those just scattered further "upstream" of the incident wave, and so on. All these phase differences ultimately combine and cancel, thereby producing no backscattered wave. The forward scattered wave more or less propagates in phase with the incident wave and therefore combines in phase with the next scatterer along the forward path. Thus these waves reinforce resulting in forward propagation of the wave.

In the argument above, we assume the scatterers are either randomly placed or very close together, as would be found in a glassy material or a crystal, with the wavelength of the light large compared with the distance between scatterers. However, if the scatterers are located on a lattice (i.e. a uniform spacing as in a crystal) and the wavelength of the light is close to the spacing between the scatterers, then you can indeed get strong backward scattering since now the path difference in the backward direction adds a 2 pi phase shift bringing the backward wave into phase with the previous scatterer. In this case all the backward scattered waves combine in phase and reinforce. This is known as diffraction and is used extensively in crystallography to determine the structure of crystals. In this case the electromagnetic waves are x-rays, which have wavelengths close to the spacing between scatterers in the crystal (strictly it is the spacing between unit cells, which are the periodic unit in a crystal and which, themselves, may contain many closely spaced scatterers as in protein crystals used in protein crystallography). One can also get strong sideways propagation if the spacing of scatterers and the incident wavelength are such that the waves reinforce in a sideways direction.