Diffraction of sound - long versus short wavelengths

Question

I am having some problems finding an explanation why long sound wavelengths travel around objects easier than short ones, hence making lower frequencies audible across longer distances. Most online sources use a slit/opening for the explanation of diffraction but somehow I still fail to grasp what happens to air molecules as they hit an object. One answer to a similar question in this forum mentions phase discrepancies and wave cancelling which seems to occur more often with shorter lengths, but I just cannot get my head around it. Any input will be much appreciated by a frustrated non-physicist.

Answer 1

Sound is really not about air molecules hitting obstacles. It's about the collective motion of the air molecules, which translates to pressure waves in the air. When the wave hits an obstacle, it reflects or is absorbed, but does not continue on its original path. Portions of the wave that bypass the obstacle do continue, but on the far side of the obstacle they diffract into the "shadow" of the obstacle. Please look at this video clip to see what happens; and read this Wikipedia article. Another Wikipedia article describes "knife-edge diffraction" and plays a video clip illustrating what happens when the diffraction is just around a single edge rather than through a two-edged slit.

Answer 2

The answer would be that that is how waves behave. One main difference between acoustic and electromagnetic waves is that you might have obstacles that are comparable (in size) with your wavelength.

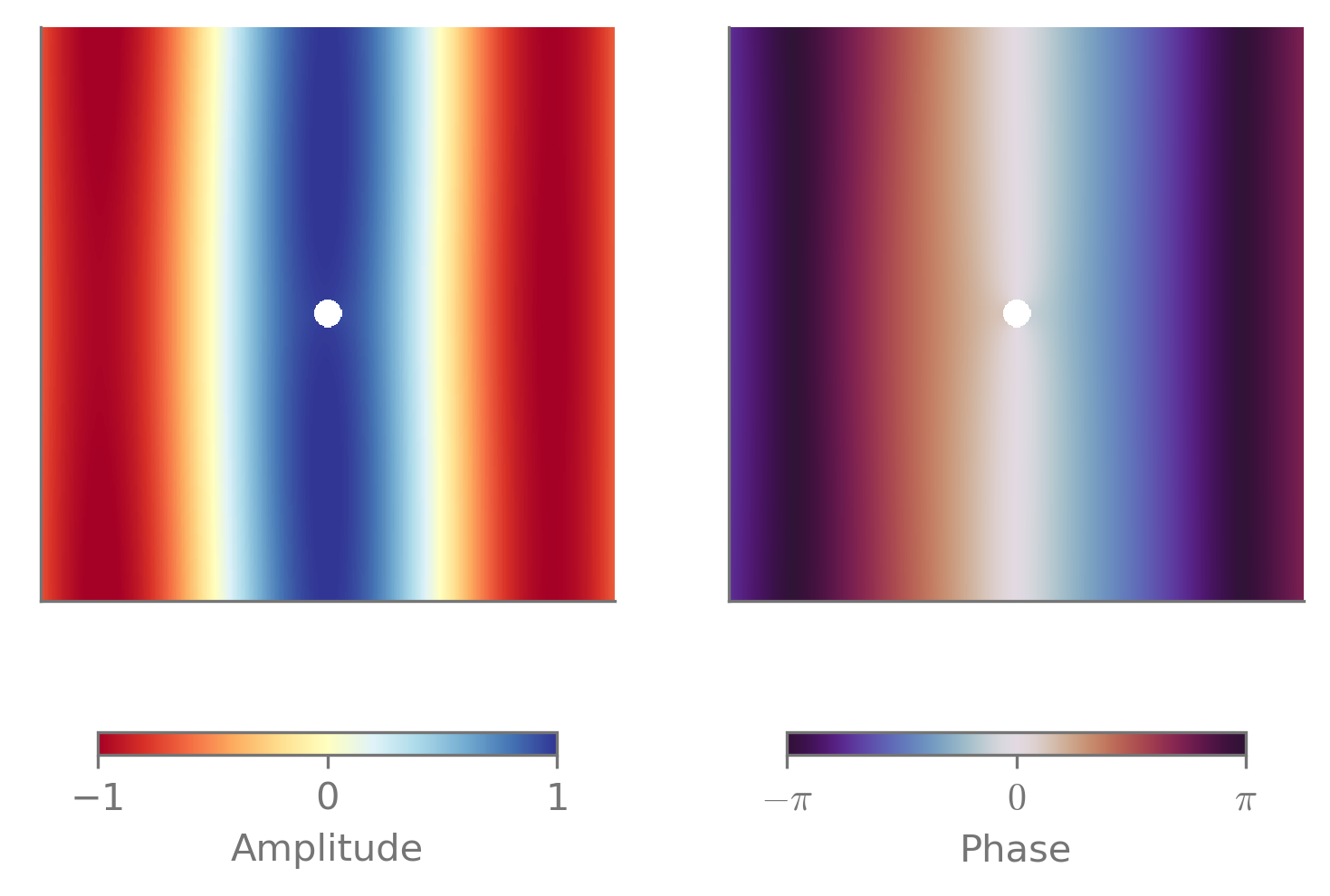
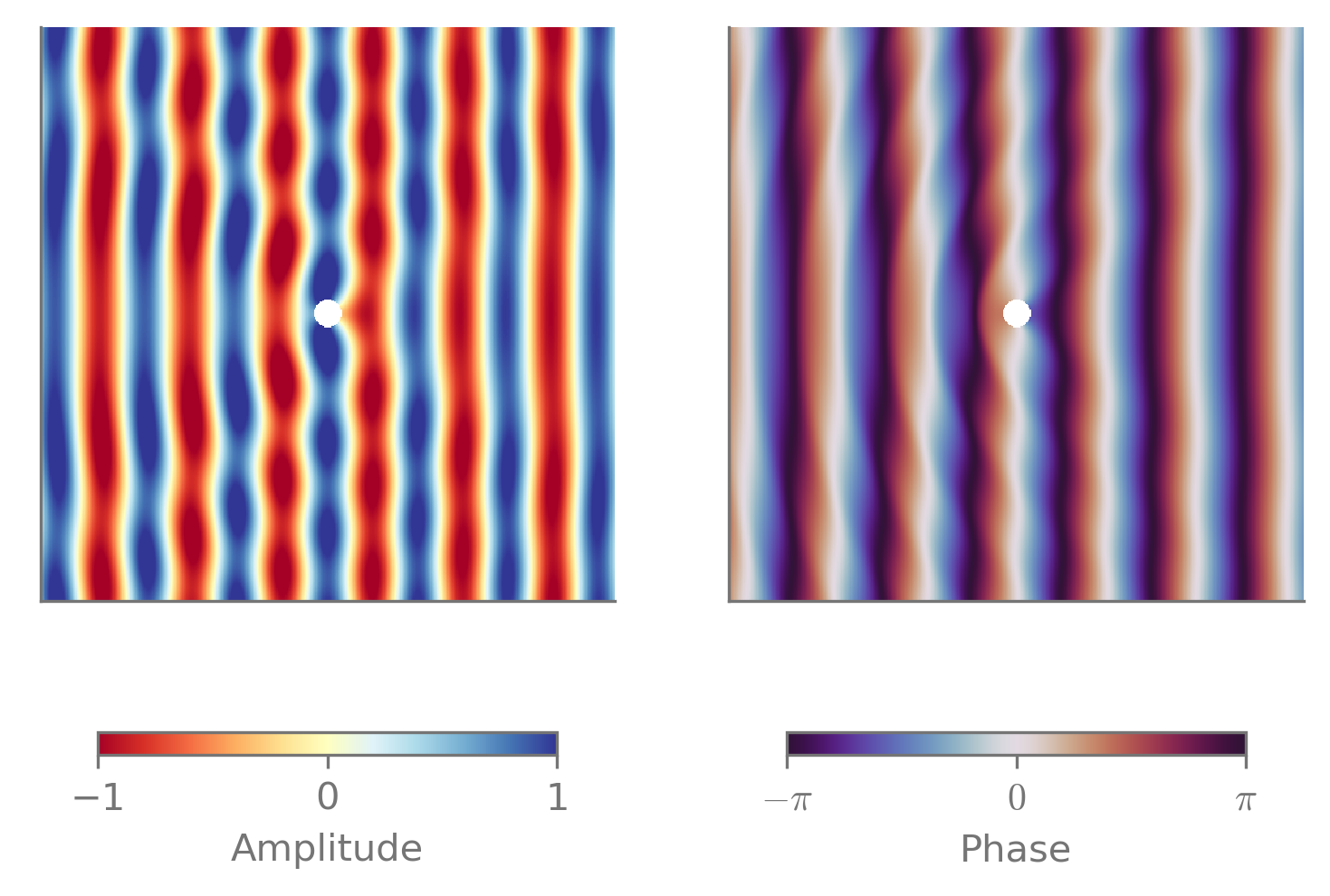
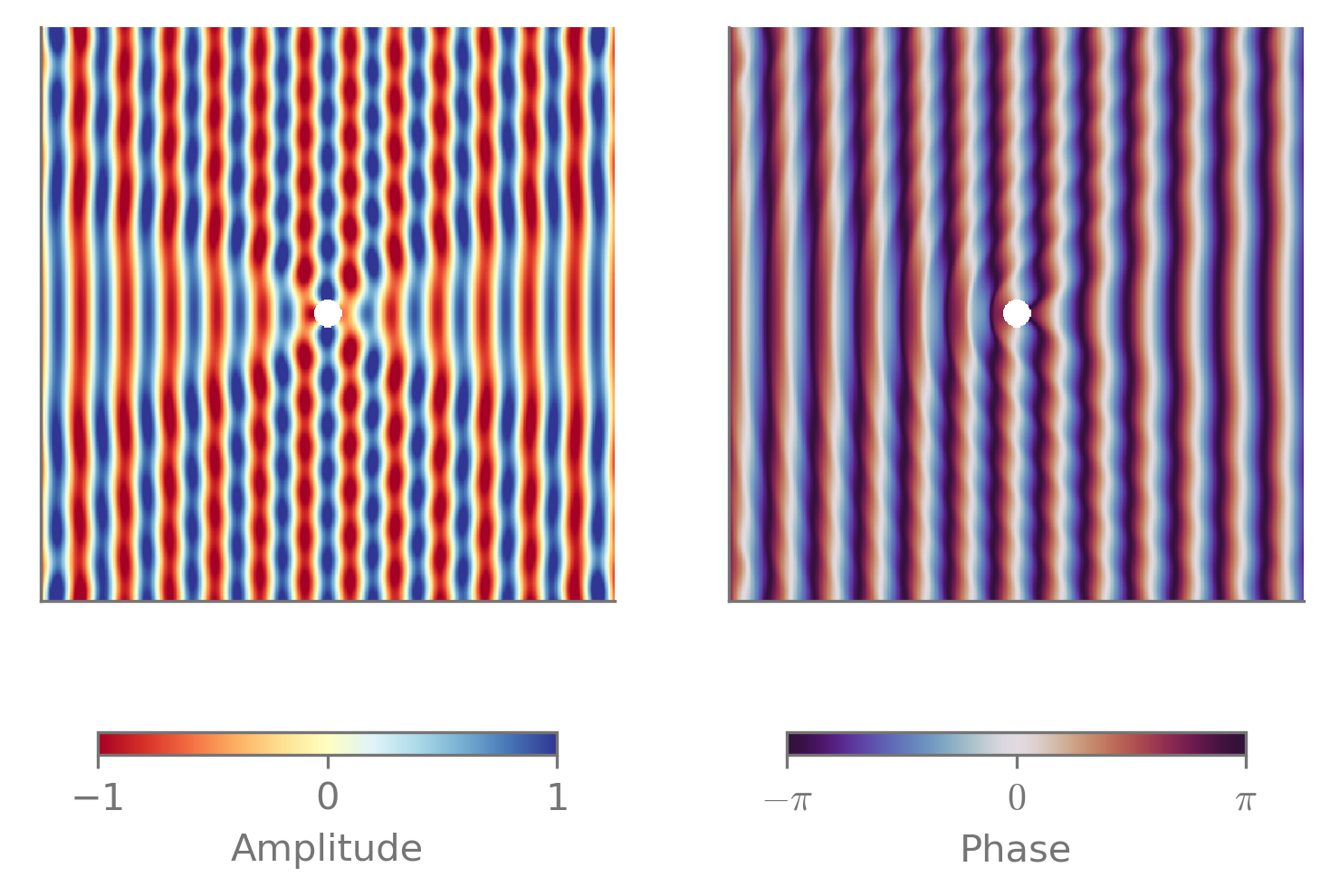
Let us consider the solution for a plane wave with amplitude $p_0$ and wavenumber $k$ incident in an infinite rigid cylinder with a radius $a$. The problem is a two-dimensional one and, for simplicity, we consider the origin of coordinates lying on the axis of the cylinder. The solution reads

\begin{equation} \frac{p(r,\theta)}{p_0} =\mathrm{J}_0(kr) + 2\sum\limits_{n=1}^{\infty} i^n\mathrm{J}_n(-kr)\cos(n\theta) - \sum_{n=0}^\infty A_n \mathrm{H}_n^{(2)}(kr)\cos n\theta \, , \end{equation}

with

\begin{align} &A_0 = - \frac{\mathrm{J}_1 (ka)}{\mathrm{H}_1^{(2)}(ka)}\, ,\\ &A_n = -2(-1)^n\frac{\mathrm{J}_{n+1}(ka) - \mathrm{J}_{n-1}(ka)}{\mathrm{H}_{n+1}^{(2)}(ka) - \mathrm{H}_{n-1}^{(2)}(ka)}\, , \end{align}

$\mathrm{J}_n$ the $n$th Bessel function of the first kind and $\mathrm{H}_n^{(2)}$ the $n$th Hankel function of the second kind. The first two terms represent the incident (plane) wave and the last one represents the scattered field.

This solution looks complicated, but the main takeaway is that the overall behavior depends on the number $ka$, i.e., in the ratio between the radius of the cylinder and the wavelength $ka = 2\pi a/\lambda$. Let us see the solution for different $ka$ values

• $ka = 0.2$

  

• $ka = 0.5$

  

• $ka = 1.0$

  

• $ka = 2.0$

  

References

1. Mow, C. C., & Pao, Y. H. (1971). The diffraction of elastic waves and dynamic stress concentrations (Vol. 482, No. PR). RAND CORP SANTA MONICA CALIF.

2. Morse, P. M., & Ingard, K. U. (1986). Theoretical acoustics. Princeton University Press.