For a rotation frequency higher than the pendulum frequency, the pendulum will fly outward, unless it is precisely vertical. Why this is so?

Question

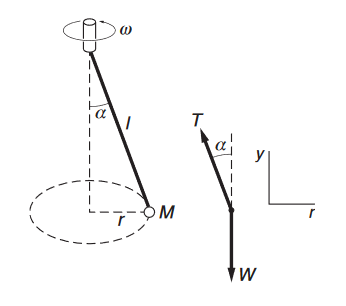
Mass M is fixed to the end of a rod of length l and negligible mass that is pivoted to swing from the end of a hub that rotates at constant angular frequency ω, as shown in the drawing. The mass moves with steady speed in a circular path of constant radius. The problem is to find α, the angle the rod makes with the vertical.

On solving this example 2.10(The conical pendulum) of the book "An introduction to mechanics by Kleppner D., Kolenkow R.", we have two solutions, $$\cos α =1,$$ $$\cos α =\sqrt{\frac g {lω^2}}.$$ First solution corresponds to the mass rotating rapidly but hanging vertically.

Second solution corresponds to the mass flying in a circular path with the rod at an angle with the vertical.

For $ω > \sqrt \frac gl$, first solution is unstable—if the system is in that state and is slightly perturbed, it will jump outward.

My question is how do we know that first is unstable?

Answer 1

To determine whether an equilibrium position is stable, you need to find the frequency of small oscillations about that point. This can be achieved by writing a differential equation for the motion when the object is slightly perturbed from the equilibrium position. In other words, if the object is in equilibrium at $\alpha = \alpha_0$, what is the net force on it if it is moved slightly to $\alpha = \alpha_0 + \Delta \alpha$?

Expanding to first order, you will obtain an equation of the form $$\frac{\text{d}^2 \alpha}{\text{d} t^2} = -\omega^2 \alpha$$

The value of $\omega$ can then be used to determine whether the object is in stable equilibrium.