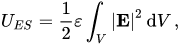0
$\begingroup$

If the total electric energy associated with a field is:

enter image description here

and the electric field of a point charge follows an inverse square law:

enter image description here

then $U_{ES}$ would be infnite if you compute the integral. Can someone shed light on what it means for "infinite total energy" to be associated with a particle?

Secondly, if charges are actually "points" and do not have a finite radius, shouldn't all matter collapse into black holes?

Could someone explain what it means for an electron or other fundamental particle to be associated with a finite radius? What is the "electron radius," and how is it measured? What is self-interaction energy?

equations from https://en.wikipedia.org/wiki/Electric_field

Improve this question
$\endgroup$
5
  • $\begingroup$ Related/possible duplicates: physics.stackexchange.com/q/34437/50583, physics.stackexchange.com/q/331354/50583, physics.stackexchange.com/q/62487/50583 and their linked questions $\endgroup$
    – ACuriousMind ♦
    Commented Mar 19, 2020 at 20:38
  • 3
    $\begingroup$ Also, please do not post formulae as screenshots, but use MathJax instead. $\endgroup$
    – ACuriousMind ♦
    Commented Mar 19, 2020 at 20:39
  • $\begingroup$ I did not understand how you compute the infinite energy ? $\endgroup$
    – seVenVo1d
    Commented Mar 19, 2020 at 22:26
  • 1
    $\begingroup$ How many questions do you have? Please ask one question per post. I agree that they are valid questions. $\endgroup$
    – my2cts
    Commented Mar 19, 2020 at 23:07
  • $\begingroup$ @Reign You write the volume integral in spherical coordinates and notice that it diverges. $\endgroup$
    – G. Smith
    Commented Mar 19, 2020 at 23:50

1 Answer 1

Reset to default
2
$\begingroup$

Infinities like this plague both classical and quantum theories of point particles. They are conventionally swept under the rug by “mass renormalization”. The basic idea is that one imagines that, if the particle had no charge, it would have a negatively infinite “bare” mass. One then adds in the positively infinite mass corresponding to the electrostatic self-energy, and assumes that the sum produces the finite observed mass.

From a practical point of view, physicists have gotten comfortable with this, because it allows theories like quantum electrodynamics to bury its infinities and produce spectacularly correct results. But they also see it as a reason to believe that theories of point particles are not the correct description of nature.

I have answered only your first question because you are supposed to ask only one at a time.

If you consider two (or more) point charges and compute their electrostatic field energy, you’ll get an infinite term for each particle, but the “interaction terms” like $\mathbf{E}_1\cdot\mathbf{E}_2$ integrate to produce finite results which are precisely the potential energy

$$U=\frac{1}{4\pi\epsilon_0}\frac{q_1q_2}{|\mathbf{r}_1-\mathbf{r}_2|}$$

of the pairs of charges. This provides a nice physical picture of where the potential energy is.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.