Would there be a potential difference across a cell connected with just a wire in complete circuit?

Question

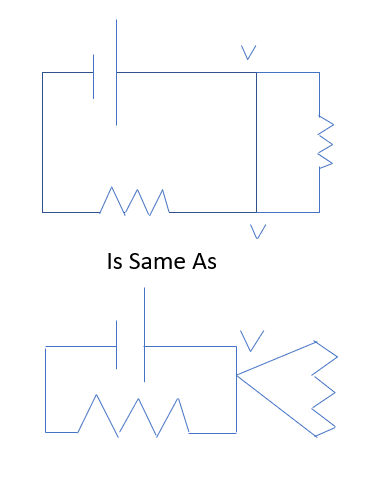
My textbook says, that across a battery or a cell there should be a potential drop equal to its e.m.f. Now consider a simple circuit with a cell of e.m.f 9 V. Let us assume a point on the negative terminal side of the cell to be of 0 potential. Then any point on the other side of the cell must be of electric potential 9 v. Now I've also learnt from sites that the potential at all points connected by a wire (or simply a conductor) remains same. Now consider any point on the wire and it is connected with the part having 9 v (with the positive terminal) and the 0 v part (the side close to negative terminal). This means that particular point must have both 9 v and 0 v at the same time instant. Unfortunately, I also read that potential at any point at any instant is unique - a point cannot have 2 potentials at the same time - implies - this circuit cannot exist.

Does this mean there will be no potential difference because two potentials cannot exist here? If that is true then no current should be flowing through the battery implying that the battery doesn't spend any of its chemical energy. But even when battery is simply connected by a wire into a complete circuit it slowly dies - it spends chemical energy by converting it to electrical energy to create the potential difference but from my assumption that shouldn't happen. How do I solve this confusion? -- There should be a potential difference but there shouldn't be a potential difference.

(note: potential difference means or is the alternative term for voltage) (assume the wire has zero resistance)

Answer 1

My textbook says, that across a battery or a cell there should be a potential drop equal to its e.m.f.

To be clear, you textbook is referring the potential measured across the battery terminals without any circuit connected to the terminals, i.e., the no load or open circuit potential across the terminals.

Now I've also learnt from sites that the potential at all points connected by a wire (or simply a conductor) remains same.

That would only apply if there was no resistance in the wire or conductor. With the exception of superconductors all wires and conductors have some resistance.

Now consider any point on the wire and it is connected with the part having 9 v (with the positive terminal) and the 0 v part (the side close to negative terminal). This means that particular point must have both 9 v and 0 v at the same time instant.

Now you are assuming that both the wire has no resistance and the battery has no resistance. All real batteries have internal resistance. When current is drawn by the wire there will be voltage drop across the battery's internal resistance, so the voltage across the battery terminals will equal the battery emf (no load voltage) minus the voltage drop across the internal resistance.

How do I solve this confusion?

You solve the confusion when you realize that no conductor (except superconductors) has zero resistance and no battery is an ideal voltage source (a source having zero internal resistance).

You said that a voltage equals e.m.f only when there is no current flowing . But according to Ohms law, V = IR. If I=0 then V=0 .How does pd exist then

Re-write Ohm's law as

$$I=\frac{V}{R}$$

Now think about the 9 V battery terminals not connected to anything. It's the equivalent as connecting a resistor across the terminals where the value of the resistance is infinite, or $R = ∞$. Then we have

$$I=\frac{emf}{∞}= \frac{9}{∞}=0$$

Hope this helps.

Answer 2

across a battery or a cell there should be a potential drop equal to its e.m.f.

Sometimes called the "open circuit voltage", this potential only exists when there is no current flowing through the battery. Internal resistance within the battery will reduce that potential as current increases.

I've also learnt from sites that the potential at all points connected by a wire (or simply a conductor) remains same.

This is a useful heuristic for low current applications. Wires do have resistance and they do have potential differences when current is flowing. It's just low enough that other portions of the circuit tend to dominate the resistance calculations. But it is not zero.

assume the wire has zero resistance

This is only a useful assumption when other resistors are present. In the case of a 9V battery, this is the battery itself. 9V tend to have rather large internal resistance and cannot generate high currents. If both the battery and the wire had very low resistance, then the current could climb quite high, possibly until one of the components failed from thermal overload (burning up).

Answer 3

• When you talk about a hypothetical situation with 0 resistance between the battery then you can refer to - Voltage drop along an idealized resistance-free wire in a circuit?

• "Potential at all the points connected by a wire remains same". They are talking about the 'wire' we use for representation purpose in a circuit diagram. Actual wires have resistance.