Do Feynman path integrals satisfy Bell locality assumption?

Question

There are generally two basic ways to solve physics models:

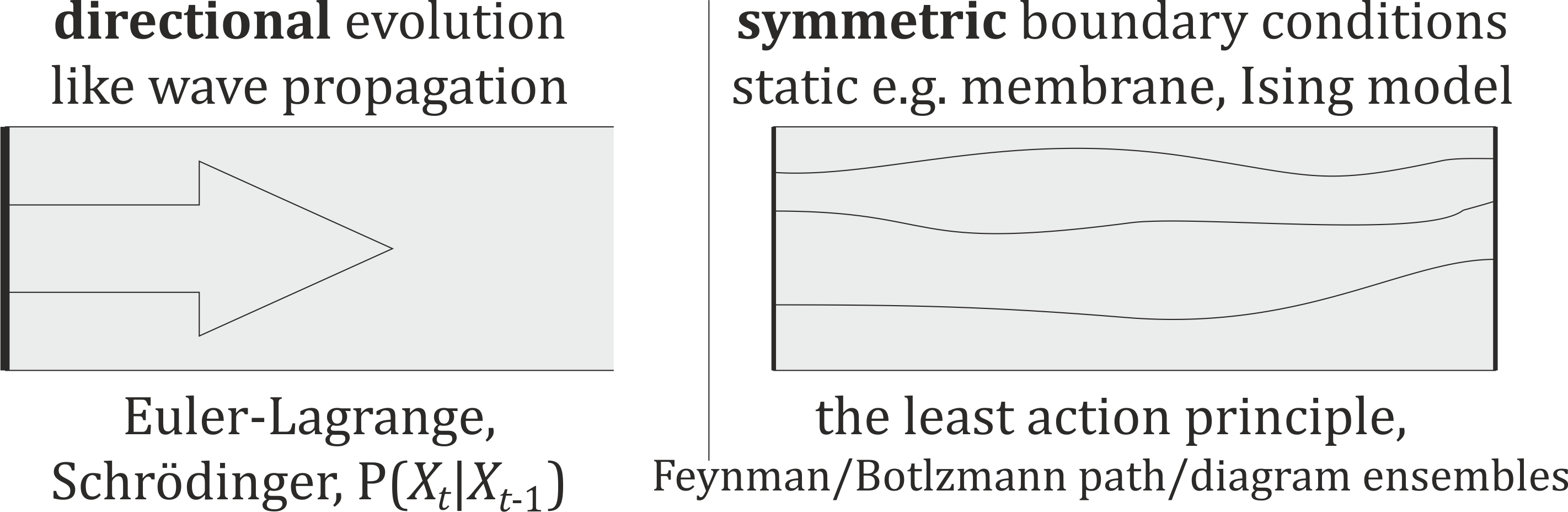
1. Directional, e.g. Euler-Lagrange equation in CM, Schrödinger equation in QM. We evolve some initial conditions in some direction, can imagine boundary conditions are e.g. in $t_0 = -\infty$.
2. Symmetric, e.g. the least action principle in CM, Feynman path integrals in QM, or of Feynman diagrams in QFT. Like choosing shape of a membrane, statistics inside Ising sequence - with symmetric boundary conditions, we can imagine they are e.g. in $t_0 = -\infty$ and $t_1=+\infty$ (measurement is between boundary conditions).

Having a solution found with 1. or 2., we can transform it into the second, but generally solutions originally found using 1. or 2. seem to have a bit different properties - for example regarding "hidden variables" in Bell theorem.

Transforming solution found in a symmetric way 2) to directional perspective 1), its state was originally chosen also accordingly to all future measurements - like in superdeterminism.

The directional ones (1.) like Schrödinger equation usually satisfy assumptions used to derive Bell inequality, which is violated by physics - what is seen as contradiction of local realistic "hidden variables" models. Does it also concern the symmetric ones (2.)?

We successfully use classical field theories like electromagnetism or general relativity, which assume existence of objective state of their field - how does this field differ from local realistic "hidden variables"?

Wanting to resolve this issue, there are e.g. trials to undermine the locality assumption by proposing faster-than-light communication, but these classical field theories don't allow for that.

So I would like to ask about another way to dissatisfy Bell's locality assumption: there is general belief that physics is CPT-symmetric, so maybe it solves its equations in symmetric ways (2.) like through Feynman path integrals?

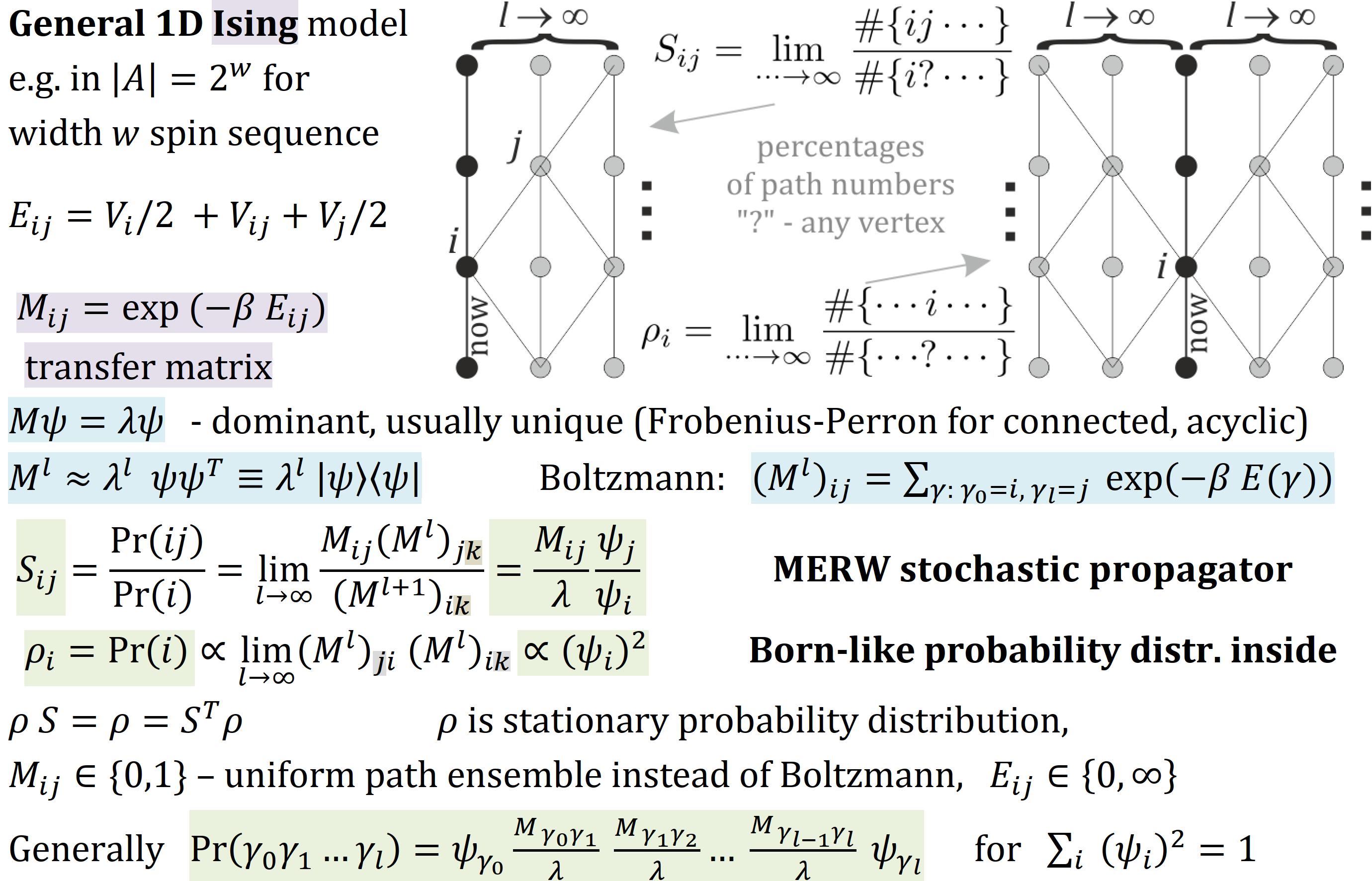
Good intuitions for solving in symmetric way provides Ising model, where asking about probability distribution inside such Boltzmann sequence ensemble, we mathematically get $Pr(u) = (\psi_u)^2$ where one amplitude comes from left, second from right, such Born rule allows for Bell-violation construction. Instead of single "hidden variable", due to symmetry we have two: from both directions.

From perspective of e.g. general relativity, we usually solve it through Einstein's equation, which is symmetric - spacetime is kind of "4D jello" there, satisfying this this local condition for intrinsic curvature. It seems tough (?) to solve it in directional way like through Euler-Lagrange, what would require to "unroll" spacetime.

Assuming physics solves its equations in symmetric way, e.g. QM with Feynman path integrals instead of Schrödinger equation, do Bell's assumptions hold - are local realistic "hidden variables" still disproven?

---

Update: Born-like formulas from symmetry in Ising model (Boltzmann sequence ensemble): $Pr(i)=(\psi_i)^2$ where one amplitude ("hidden variable") comes from left, second from right:

Answer 1

I’m answering the question you asked at the end:

Assuming physics solves its equations in symmetric way, e.g. QM with Feynman path integrals instead of Schrödinger equation, do Bell's assumptions hold - are local realistic "hidden variables" still disproven?

The short answer is “Yes.” Solving QM with path integrals is equivalent to using Schrödinger’s equation, so the details of Bell’s inequalities are irrelevant. Whether you use path integrals or Schrödinger’s equation you will get the exact same results.

There are two sides to Bell's inequalities. On one side there is a quantum mechanical calculation and on the other side there is a calculation using an unspecified local hidden variable theory.

If you do look at the details of the quantum side of Bell’s inequalities, you will notice that its derivation only depends on the initial entangled state and calculations of correlations. Neither path integrals nor Schrödinger’s equation are being used.

Answer 2

Assuming physics solves its equations in symmetric way, e.g. QM with Feynman path integrals instead of Schrödinger equation, do Bell's assumptions hold - are local realistic "hidden variables" still disproven?

Yes. To check this for spins, you can take a spin to a point $\hat{n}$ in the group manifold $SU(2)$. Then take wave function to be defined by the path integral over paths $\mathcal{C}_{\hat n(t)}\equiv \mathcal{C}(\hat{n}_0(t_0)|\hat{n}(t))$ where the system is a point $\hat{n}_0$ at time $t_0$ and at point $\hat{n}$ at time $t$.

$$\psi(\hat{n},t)\equiv\int\!d\mathcal{C}_{\hat n(t)} \exp\left(- \int_{\mathcal{C}_{\hat n(t)}}\hat{n}\cdot d\hat{n}\right). $$

The statistics of the the wavefunction $\psi$ are exactly those of quantum mechanics and will satisfy Bell's theorem at time $t$. This path integral is also exactly what you integrate over. Thus the path integral, on any time slice, contains Bell's theorem. In other words, Bell's theorem is a consquence of superpositions, which is exactly what you are constructing in the path integral.