We know a charge particle under the influence of external fields to be:
$$\vec{F}=q(\vec{E}+\vec{v}\times\vec{B}), $$
where $E$ and $B$ fields are from external fields not the field by the charge itself. Then, if we applied to the case of localized charged distribution, the formula looks like:
$$\vec{F}=\iiint_V\rho(\vec{E} +\vec{v}\times\vec{B}) dV,$$
$E$ and $B$ fields should have the same character as the point charge case, and we can derive out the Maxwell Tensor.
However, in Griffith's books(Introduction of Electrodynamics 4ed, Chapter 8 Ex8.2), the problem is: Determine the net force on the "northern" hemisphere of a uniformly
charged solid sphere of radius $R$ and charge $Q$.
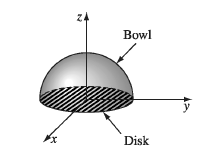
He substituted the electric field of the charged sphere $$\vec{E}=
\begin{cases}
{1\over4\pi\epsilon_0 }{Q\over R^2}{\hat{r}},&\text{on the bowl}\\{1\over4\pi\epsilon_0 }{Q\over R^3}{\vec{r}},&\text{inside the disk}
\end{cases}$$
into the Maxwellian stress tensor and calculated the net force on the hemisphere by the following formula.$$\vec{F}={\iint_S\overleftrightarrow{T}\cdot\vec{dS} }$$, where $$\overleftrightarrow{T_{ij}}\equiv\epsilon_0(E_iE_j-{1\over2}\delta_{ij}E^2)$$
According the original recognition to Lorentz force law, the force on the charge should be from the external $E$ and $B$ fields. If we used the field made of the hemisphere to calculate the force on it.
That means we used the field stemmed from the charge on the hemisphere exert a force on itself. Does that example make sense? or If I misled something?
