Why does the reversible adiabatic expansion do more work on the surroundings compared to the irreversible adiabatic expansion?

Question

I am wondering why a reversible adiabatic expansion results in a higher work output on the surroundings though the irreversible adiabatic expansion has to overcome the frictional force and therefore, in my opinion, has to do more work and its internal energy is reduced more even when some of the dissipated energy is "restored". Or should we consider the dissipated energy as energy gained from the surroundings. But this is an adiabatic process and there is no heat transfer possible. I am confused

Answer 1

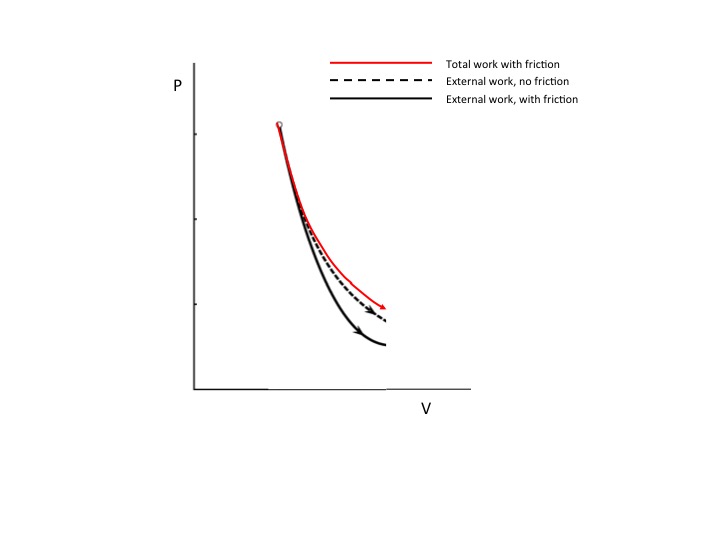
For a quasi-static adiabatic process, less adiabatic work is done on the surroundings with internal friction (irreversible quasi-static process) than without internal friction (reversible quasi-static process). That is because part of the total work done by the gas is internal friction work and winds up as internal energy. Thus at any given volume during the expansion, the internal energy is higher when internal friction work is done than without internal friction work. Per the first law $\Delta U=-W$ where $W$ is the external work done by the system and consequently less external work is done on the surroundings with internal friction then without. Refer to the PV curves below.

The dotted black curve shows the adiabatic work done on the surroundings (external work) if there were no friction.

The solid black curve is the external work done when there is friction. Note that the pressure for the solid black curve is the external pressure and not the gas pressure. The gas pressure (red curve) is higher than the external pressure because the gas has to do work to overcome the opposing friction force of the piston that is not present for the dotted black curve. The external work done is always a function of the external pressure.

The red curve shows the work done by the gas on the inside face of the piston. The external work done by the outside face of the piston on the surroundings is less because work is done by the gas on the piston to overcome friction. The pressure for the red curve is the gas pressure, not the external pressure. Both the pressure and temperature of the gas is higher due to the friction work.

Could you please explain the same for an irreversible adiabatic compression because if the external pressure compress the gas there also has to be a reduced work "output" (from the point of the view of the environment) due to piston friction. That is the thing I do not understand. In my opinion it is the same as an expansion, just reversed albeit we have more work "output" and a higher internal energy after the compression, respectively. Somehow I have a fallacy. I try to understand this for three weeks. –

The effect of friction is not the same for compression and expansion.

Mechanical friction between the piston and cylinder wall elevates the temperature of the cylinder wall that rubbed against the piston.

During expansion the piston moves out and the gas (the system) is exposed to the elevated wall temperature. Heat is transferred from the wall to the gas increasing the internal energy of the gas.

During compression the piston moves inward exposing the surroundings to the elevated wall temperature on the side of the piston opposite the gas. Heat is therefore transferred from the walls to the surroundings and not the gas. This is not contrary to the process being adiabatic. The heat $Q$ in the first law equation is heat transfer between the system and the surrounding. The system is the gas. There is no heat transfer between the gas and the surroundings. The piston face and portions of the cylinder walls that enclose the gas are the system boundaries.

Bottom line: More work must be done by the surroundings on the gas in order to increase its internal energy with friction than without friction, because some of the work done by the surroundings results in heat transfer back to the surroundings.

Hope this helps

Answer 2

Here the work output means how much useful work a system has done. Since it is an adiabatic process , dU is converted to dW , and for a fixed dU , obiusly reversible system will give you more output. Understand it by an analogy, Its like you have fixed amount of energy. And in one case you have to run on highway , and in other on an marshy , muddy road .

Of course. You have no energy drink available ( dQ =0) Now definitely you run more distance on highway than on the marshy road. You will not say I did more work by struggling with mud , stone and terrain.

Because I am not concerned about that. I just want in which way you can run the greater distance . Same is with thermodynamics.we are just calculating the useful work. In engine we want efficiency , we don't care whether its truck engine aur motorbike engine.

Answer 3

The essence of the distinction between a reversible and irreversible process is that in the latter, some energy is "degraded" (i.e. entropy is generated), while in the former, it is not.

(Macroscopic) force generation, however, is not energy degradation - it is doing work (ordered, or structured, use of energy). Hence, in a reversible process, the greatest possible energy will go into producing work.

If, however, it is irreversible, then the part of the energy that got degraded into entropy is no longer available for doing work. Hence less work is done. The push from the expanding gas is weaker.