Consider an arbitrarily shaped body immersed partly in one fluid and partly In another. Can an expression be derived for two different forces applied by the the two fluids on the immersed volumes by using Archimedes principle separately for the displaced fluids?
If so how will an upward force be applied by the upper fluid layer
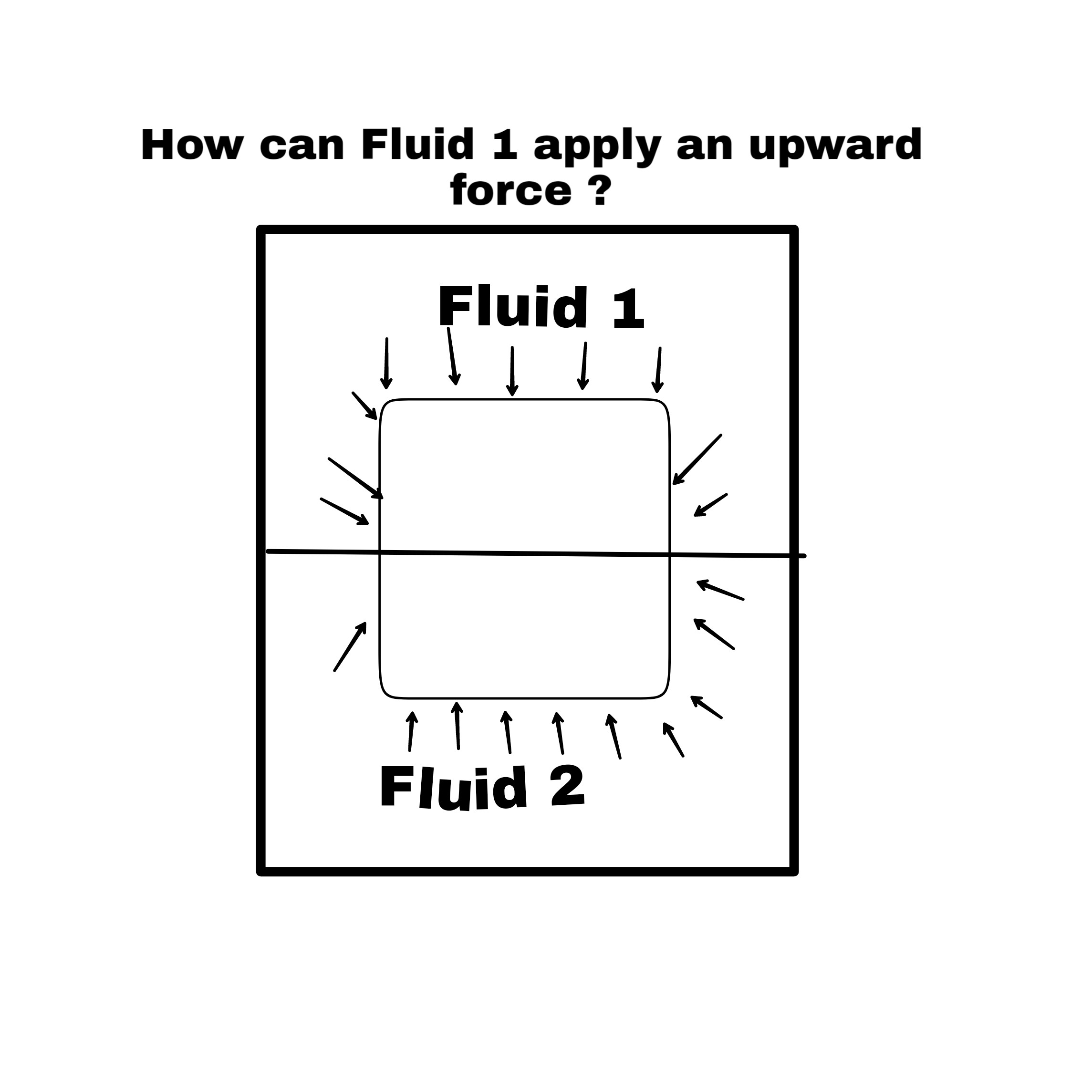
The total buoyant force on the object comes out of integrating all of the forces per unit area acting on the surface of the object—in this case, due only to pressure.
Because all the forces per unit area add up—the physics of buoyancy is linear—you can think of this physical system as two separate blocks immersed in each fluid, bridged by an infinitesimally thin horizontal layer of fluid at the interface. Using Archimedes' principle on each of these blocks, correctly determining the pressure distribution of the liquid, and adding each of these resultant forces up, you can determine the buoyant force on the single block.
Notice that in that calculation, there is indeed an upwards-pointing contribution to the force on the top block coming from the "fictional" bottom face. This contribution isn't "real", since all of the actual upwards-pointing forces per unit area come from the true bottom of the block, but it generates an equivalent mathematical solution when combined with the forces on the "fictional" bottom block due to the linearity of buoyancy.
