Time period of a simple pendulum in an accelerated frame

Question

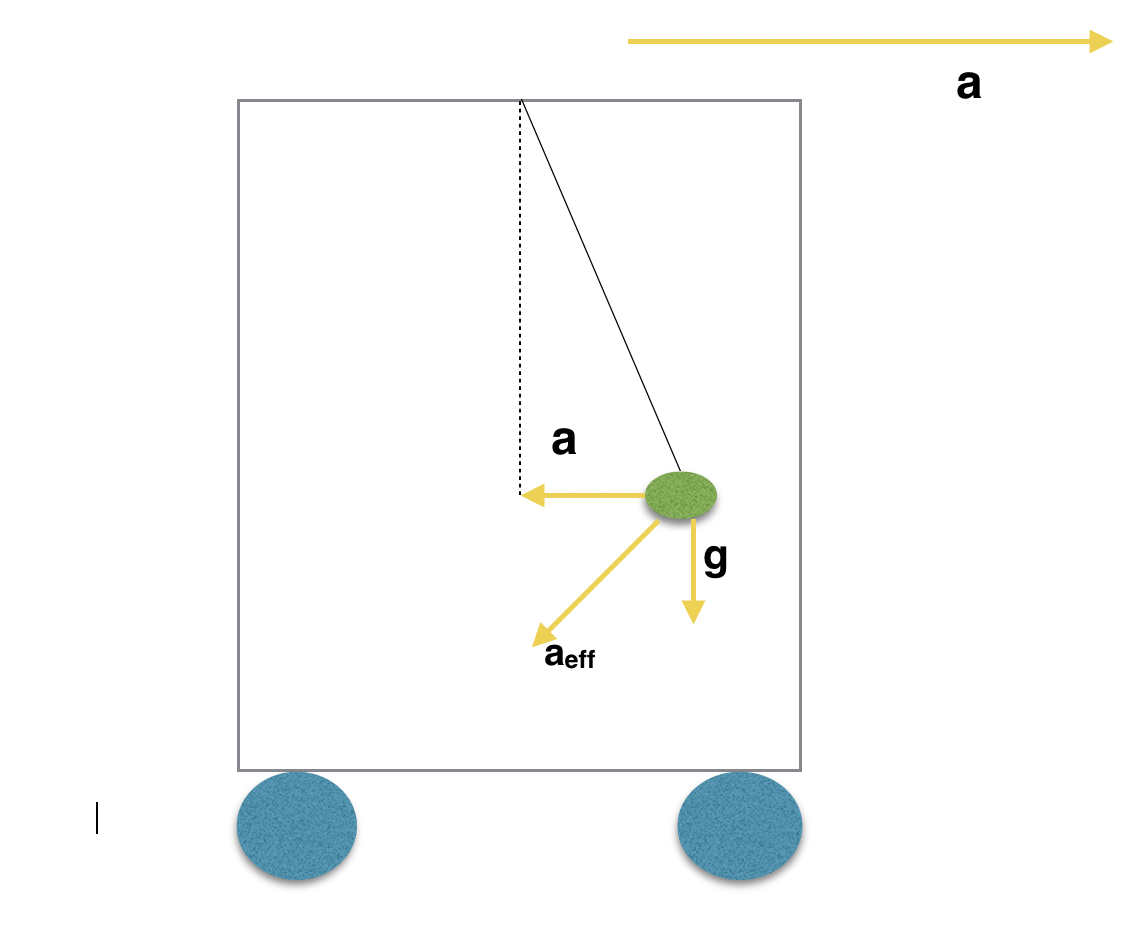
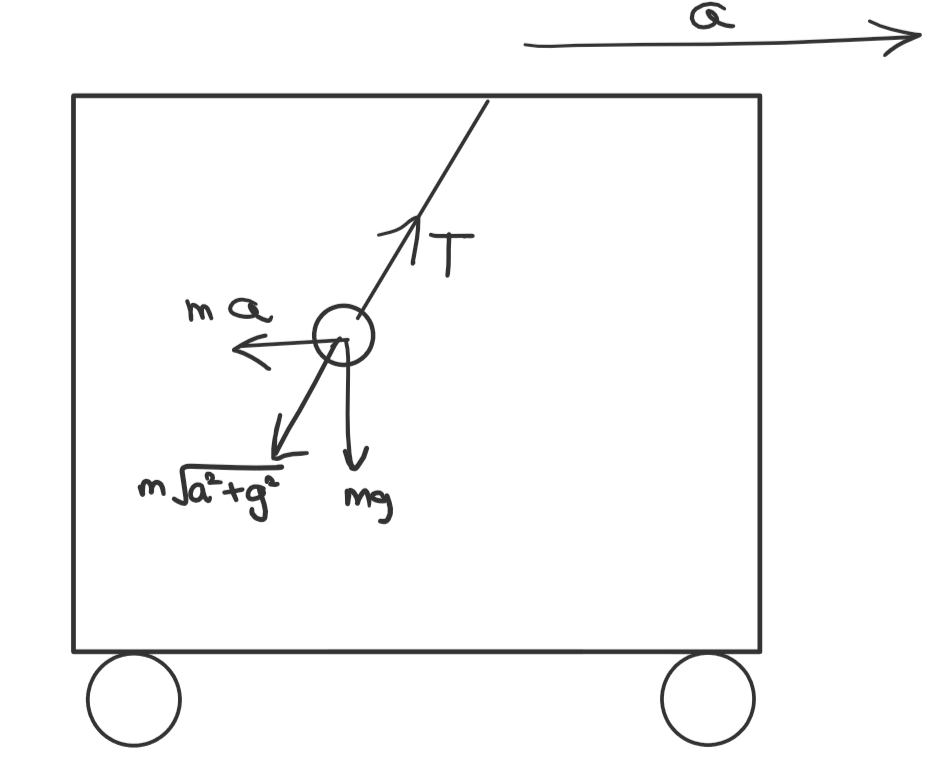
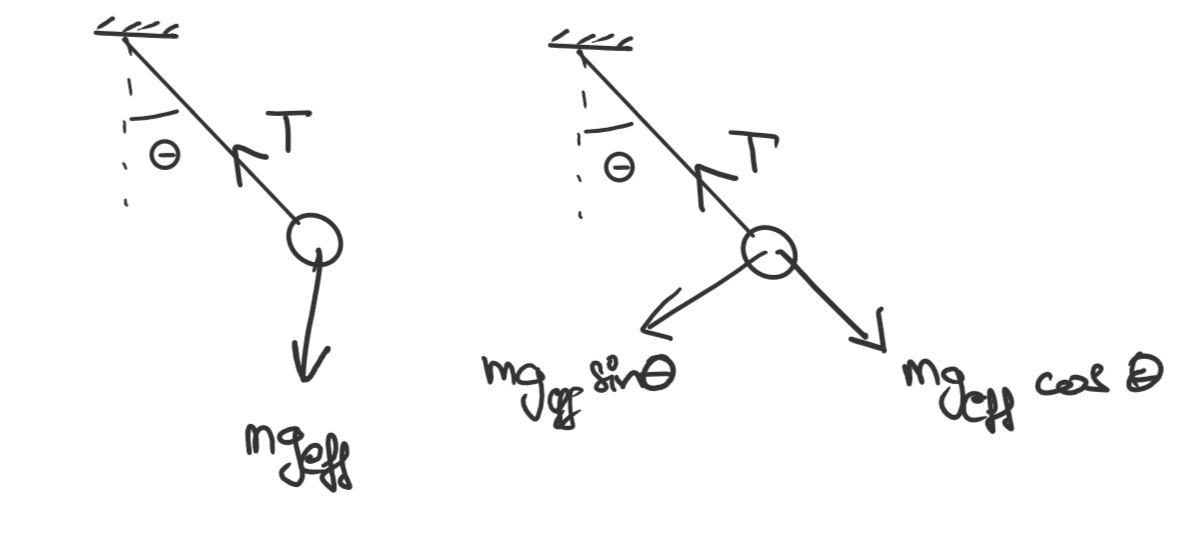
Suppose I have a simple pendulum oscillating in an accelerated frame then my textbook says that the time period of the pendulum is no longer given by:

$$ T = 2\pi\sqrt{\frac{L}{g}} $$

but by:

$$ T = 2\pi\sqrt{\frac{L}{a_{eff}}} $$

where $a_{eff}$ is the magnitude of vector sum of the acceleration due to gravity, $g$ and acceleration of the frame $a$.

Can anyone explain why it is so?

Answer 1

In the frame of the cart a pseudo force acts towards the left. Notice that this is the new equilibrium position of the bob:

Now, let us turn the axis of the drawing so as to make it easier for us to understand.

Here $g_{eff} = \sqrt{a^2+g^2}$

Now, we take a small angular displacement of $\theta$ and analyse the motion.

We get: $$ \tau = mg_{eff}l\sin\theta\\ $$ But, $ \theta << 1$ $$ \tau = mg_{eff}l\theta\\ $$ So, we get, $$ C = mg_{eff}l\\ and\ I = ml^2\\ $$ Finally, for time period, $$ T = 2\pi \sqrt{\frac{I}{C}}\\ T = 2\pi \sqrt{\frac{ml^2}{mg_{eff}l}}\\ \implies T = 2\pi \sqrt{\frac{l}{g_{eff}}}\\ \implies T = 2\pi \sqrt{\frac{l}{\sqrt{g^2+a^2}}} $$ Hope this helps!

Answer 2

That's because of Pseudo Force (Fictitious Force) acting on the bob as you're considering an accelerated frame. Along with earth's gravitational force acting on the bob, there is another force called "Fictitious force" acting on the bob whose direction is opposite to the direction of the accelerated frame of reference. So, it's no more just $g$ rather it is the vector resultant of $g$ and $a$, where $a$ is the acceleration of the frame. The magnitude of the resultant is $\sqrt{a^2 + g^2}$ which is $a_{eff}$.