3
$\begingroup$

From an article on Wikipedia:

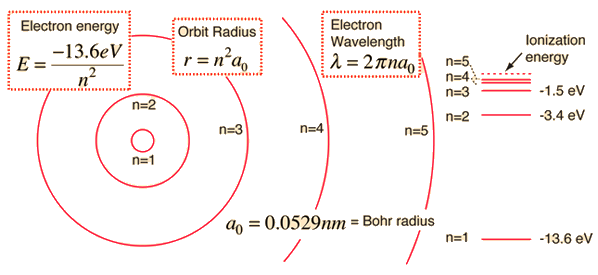
For an electron to transition between two different states, (...), it must absorb or emit a photon at an energy matching the difference in the potential energy of those levels

Lets consider scenario when we have an atom with some electron in its ground state and a photon with matching energy (exactly enough to excite our electron) encountering that atom.

Questions are:

  1. Theoretically: do we have 100% absorption probability here?

I realize that many factors might be missing, so another version of this question is: "How physicist can correct my scenario to explain how we could (or why we could not) theoretically have 100% absorption probability?".

  1. Practically: I assume that we can not know the state of electron before the measurement. Therefore, the state of electron must be uncertain (being in a superposition of states). However, can we somehow prepare our electrons in a lab (so they will occupy only its ground state) to actually produce "100% absorption probability"?
Improve this question
$\endgroup$

2 Answers 2

Reset to default
5
$\begingroup$

There are cross sections that one can measure or compute for an atom. That cross section can be expressed as an area. If that is close to $\pi r^2$ of the atom, I suppose you would call that a probability of 100 %. (But for example neutron absorption cross sections can be much larger than the size of a nucleus.)

Here some data for photoionization:

enter image description here

Image source

PS: See also this answer about the amount of light absorbed by graphene (a single layer of carbon atoms).

Improve this answer
$\endgroup$
1
  • 3
    $\begingroup$ This is indeed correct ─ for free-space interactions. In restricted dimensionality (say, if the photon is confined to a waveguide), the cross-section language needs to be dropped, and the interaction probability can go as high as 100% if the photon is on resonance (example, example). $\endgroup$
    – Emilio Pisanty
    Commented Dec 9, 2019 at 11:05
1
$\begingroup$

Probability is a function, mathematically the same for quantum states and for classical states.

Take the probability curve for a dice to come up face, 1,2,3,4,5,6 (top left)

enter image description here

The curve is normalized to 1, i.e. your 100%. You cannot get a probability 1 , In general a probability 1 means a sure thing, and in a quantum mechanical probability, a stable state.

Lets consider scenario when we have an atom with some electron in its ground state and a photon with matching energy (exactly enough to excite our electron) encountering that atom.

Let us take the possible energy levels of the hydrogen atom:

hydeogenatom

It is only in the n=1 level that an electron has a probability of 1, and the atom is stable.

If a photon impinges on the atom with energy the energy difference to higher lines, there is a calculable probability for the electron to be excited to the higher level, a distribution. It cannot be 100% because of the nature of probabilities and the nature of quantum mechanical scattering amplitudes.

In general though, electrons bound in atoms do not scatter independently, it is the whole atom that transitions in energy levels, and may decay back to the ground state by emitting characteristic photons, in the above example the spectrum of the hydrogen atom.

Improve this answer
$\endgroup$
11
  • $\begingroup$ Thank you for a great source! I assume that by "calculable probability" you mean this distribution: hyperphysics.phy-astr.gsu.edu/hbase/quantum/hydrng.html - ? So, if I got you correctly: even if we somehow will take "prepared" hydrogen atom (with its electron on, say, n = 1 level), and if we will be going to impinge this hydrogen atom with the "right" photon - we will never succeed to set "right colliding conditions" for absorption to be 100% certainly happen, is that right? $\endgroup$
    – Victor Novak
    Commented Dec 8, 2019 at 18:47
  • 1
    $\begingroup$ Note that the probability of finding the electron at a certain radius is not measurable for radii larger than the ones shown. . This is for the stable atom, that is shown in the solution. One would have to solve the scattering of photon+hydrogen amplitude, which will be a different function. $\endgroup$
    – anna v
    Commented Dec 8, 2019 at 19:29
  • 1
    $\begingroup$ "It cannot be 100% because of the nature of probabilities" - this is hogwash, I'm afraid. It's perfectly possible for single-photon states to have 100% interaction with atomic systems. This turns out not to happen in 3D, but it's an everyday tool in cavities. "because of the nature of quantum mechanical scattering amplitudes" is a non-explanation. $\endgroup$
    – Emilio Pisanty
    Commented Dec 9, 2019 at 9:45
  • $\begingroup$ @EmilioPisanty Sorry but I have to remind you of Hesenberg's uncertainty principle. In quantum mechanics . It could be 99.999999%, never 100. Only stable levels have a probability of finding the electron at that level 100%, because of the nature of the wavefunctions. $\endgroup$
    – anna v
    Commented Dec 9, 2019 at 10:37
  • $\begingroup$ @annav Apologies, but that is yet again hogwash; the only sense in which it's true is the parts that are platitudes about probabilities in the real world with no connections to QM. The HUP has nothing to do with it. $\endgroup$
    – Emilio Pisanty
    Commented Dec 9, 2019 at 10:51

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.