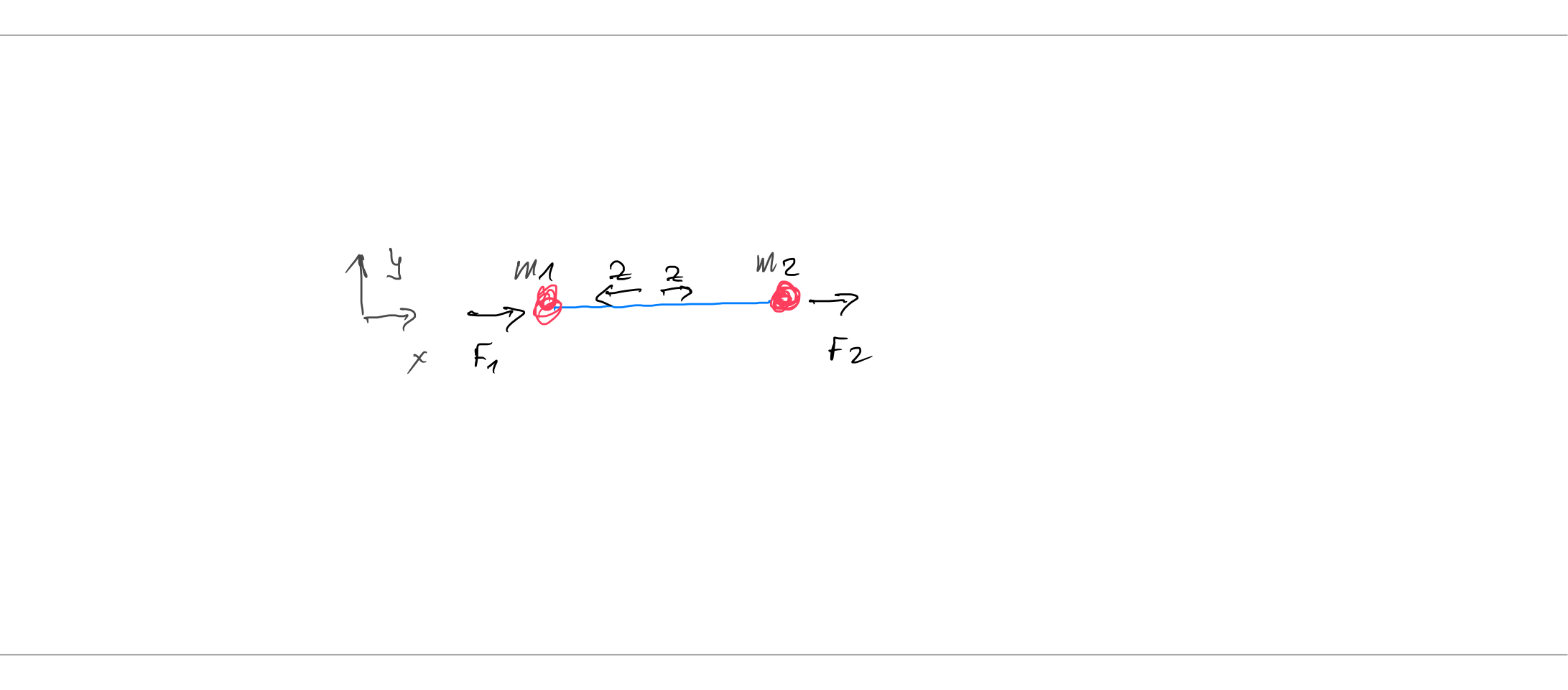
My mechanics book claims that the total force on the $i$-th particle is $$ F_i=K_i+Z_i \tag{2.5} $$where $Z_i$ is the force due to constraints and $K_i$ the real, dynamic force. Then, the book states without proof that $$ \sum Z_i\cdot \delta \mathbf r_i=0. $$ The only reason why it is true that I can think of is that the constraining force must be "perpendicular" (or normal) to the surface on which the particles can move. However, the notion of perpendicularity only makes sense for one-particle systems restricted to a surface. It does not make sense, for example, for the system in which two particles are connected by a light, rigid rod.
This generalisation of perpendicularity to more than two particles does not seem clear to me. Is there any mathematically formal way to talk about this notion of "perpendicularity"? Or is there a better explanation for why the total work done by forces from constraints vanishes?
References:
- Florian Scheck, Mechanics, p. 92.