Is there more than one legitimate way to determine whether resistor are in series or parallel?

Question

On various other threads I noticed, that

"If all of the current leaving one resistor enters another resistor, the two resistors are in series."

similarly,

"If the current must travel down two or more paths, items on those paths are in parallel until those paths reunite"

"If all of the voltage across one resistor is across another resistor, the two resistors are in parallel."

"If two resistors share the same nodes at both ends of resistors, then they are parallel. If they share only one node then they are in series."

Are these all legitimate ways to work determine whether resistors are in series parallel or are there any exceptions / complex circuit problems which cannot be solved with these rules? Also which method is best and least likely to go wrong with?

Answer 1

"If the current must travel down two or more paths, items on those paths are in parallel until those paths reunite"

I don't believe this is generally true. Consider the case of one path being a resistor and the other path being two series connected resistors. For this case, there are no parallel connected resistors.

"If two resistors share the same nodes at both ends of resistors, then they are parallel. If they share only one node then they are in series."

I don't believe the final sentence of this quote is generally true. Consider the case I gave above.

which method is best and least likely to go wrong with?

As I've written before, two series connected circuit elements have identical (not equal but identical) current through (all of the current through one circuit element is through the other circuit element). If this doesn't hold, the two circuit elements are not series connected.

Similarly, two parallel connected circuit elements have identical (not equal but identical) voltage across...

Answer 2

Are these all legitimate ways to work determine whether resistors are in series parallel

The first and third, with the exception of some slight tweaking, are the most common versions I have seen and seem legitimate to me. The other two are, at least to me, problematic, for the following reasons.

"If the current must travel down two or more paths, items on those paths are in parallel until those paths reunite"

This doesn't sound right to me, particularly the last part "until those paths reunite". It seems that it should say "provided that those paths reunite". But maybe I'm not understanding it correctly.

"If two resistors share the same nodes at both ends of resistors, then they are parallel. If they share only one node then they are in series."

The first sentence looks OK. I'm not sure about the second sentence. Two resistors can share one node, but then other resistors could also be connected to that same node and then obviously the two resistors would not be in series. No matter how many resistors are connected to the same node, it's still only one node.

I would add the italics wording as follows:

"If they share only one node, and there are no other resistors connected to that node, then they are in series".

or are there any exceptions / complex circuit problems which cannot be solved with these rules? Also which method is best and least likely to go wrong with?

I think the first and third version are the least ambiguous and you are not likely to go wrong with them. That said, there are some complex circuits that don't lend themselves to simplification by these rules. But there may be other tools to simplify complex circuits. One tool that comes to mind is the $\Delta$-Y transformation, also known as the Pi -T transformation. See the following link on how to use it:

https://www.allaboutcircuits.com/textbook/direct-current/chpt-10/delta-y-and-y-conversions/

Hope this helps.

Answer 3

This is a very good question and you are not alone in finding it difficult to decide which resistors are in series and which resistors are in parallel.

So go back one step and think about the reason that you want to know if resistors are in parallel or in series.

The reason is that if you can identify series and parallel resistors you have formulae which enable you to find the equivalent resistance.

So go back another step and think about the assumptions which were made when the series and parallel formulae for equivalent resistance were derived.

• For the resistors in series the crucial condition was that the current through each of the resistors is the same.
• For resistors in parallel the crucial condition is that the voltage across the resistors was the same.

Consider the following circuits:

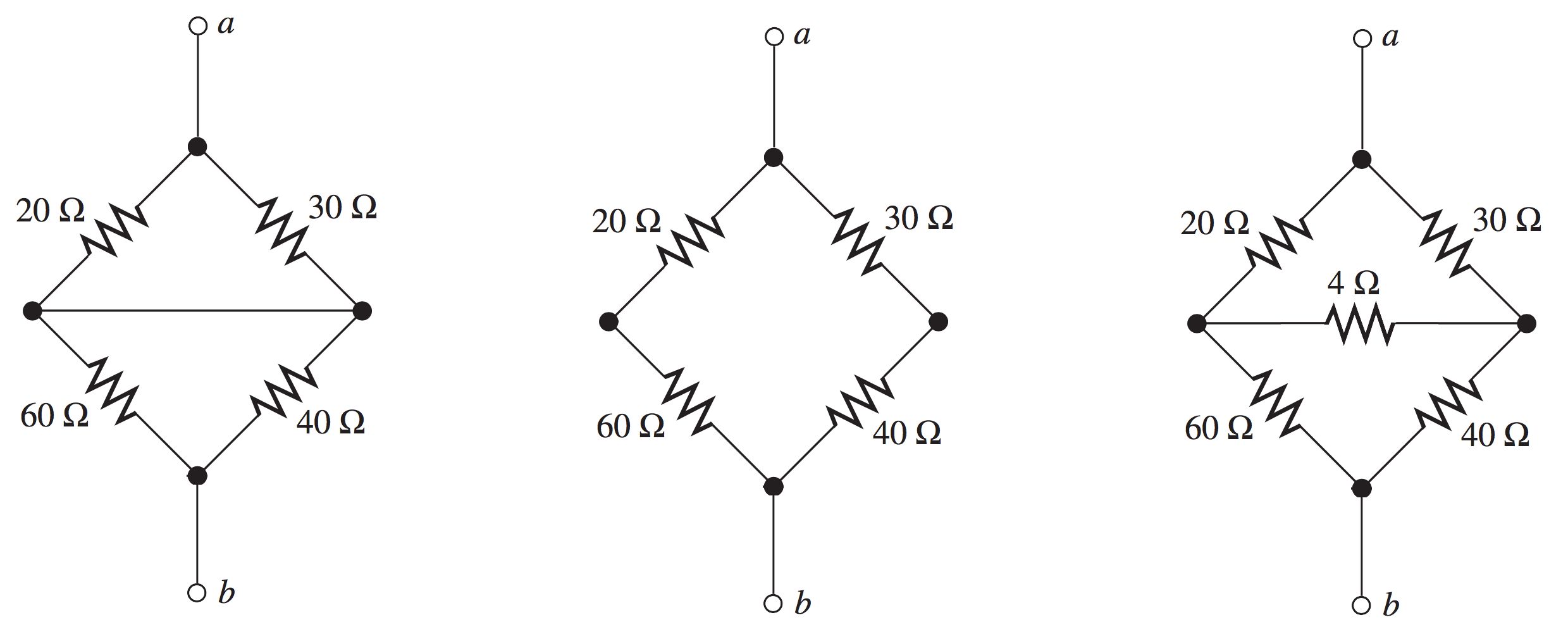
So looking at the left hand circuit can it be decided whether or not the 20 Ω and the 60 Ω are in series?
Because the current through the 20 Ω resistor is not the same as the current through the 60 Ω resistor you cannot consider these two resistors to be in series.
So for these two resistors the series equivalent formula should not be used.

Are the 20 Ω and the 30 Ω in parallel?
The answer is “yes” because the voltage across them is the same and so you can use the parallel equivalent formula with them.

What about if the connection across the middle was removed as in the middle circuit?
Then for the 20 Ω and 60 Ω resistors the series equivalent formula can be used because the current passing through them is the same.

Now suppose that instead of the connection across the middle there was a 4 Ω resistor as in the right hand diagram.

Are the 20 Ω and 60 Ω resistors in series?
“No”, because the current through them is not necessarily the same.

Is the 30 Ω resistor in parallel with the 20 Ω and 4 Ω resistor combination?
At first glance it looks like they are because the voltage across the 30 Ω resistor is the same as the voltage across the 20 Ω and 4 Ω resistor combination.

Here one must go one step back and ask:
“How am I going to use my parallel equivalent formula?”
The answer is that first one must find the equivalent resistor for the 20 Ω and 4 Ω resistor combination.

Can that be done?
“No”, because the current through them is not necessarily the same.
That means that the series equivalent formula cannot be used which in turns means the parallel equivalent formula cannot be used.