Deriving the Integral Form of the Navier Stokes equation

Question

I'm trying to follow the book Turbulence by Davidson. Currently I'm having trouble in converting the differential NS equation to its integral form but I cannot see clearly how the Divergence theorem is applied to obtain it.

Given that the NS equation is:

$$\frac{Du}{Dt} =\frac{\partial u}{\partial t} + (u\cdot\nabla)u= -\nabla\left(\frac{P}{\rho}\right) + \nu\nabla^2u $$

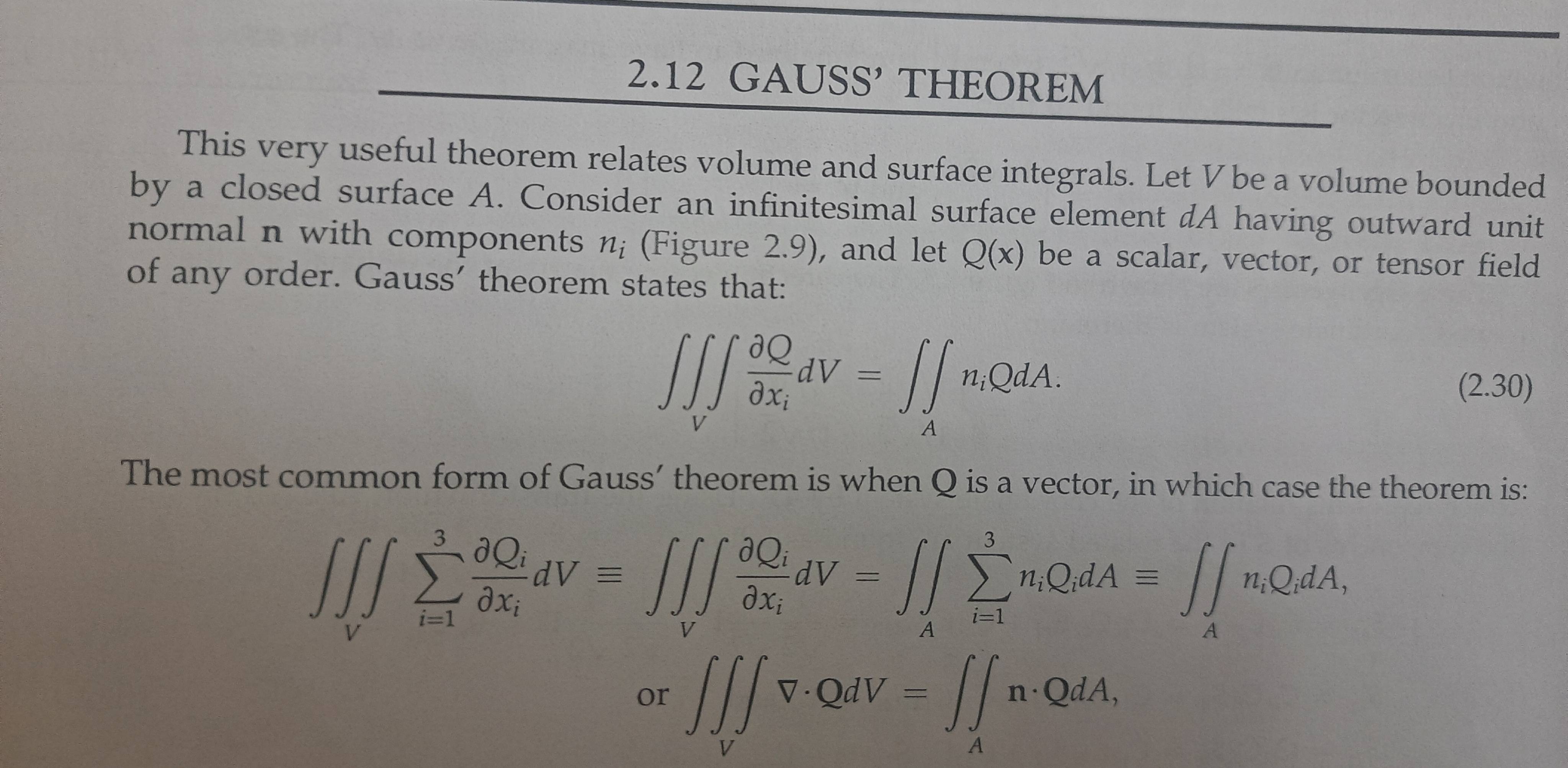
And knowing that the divergence theorem gives

$$\oint F\cdot dS = \iiint \nabla\cdot F\,dV$$

Therefore the integral form will be:

$$ \frac{\partial }{\partial t} \iiint \rho u_i\,dV = -\oint u_i(\rho u\cdot dS) - \oint PdS +\text{viscous term}$$

I've been trying for hours to convert $ \iiint(u\cdot \nabla)u\,dV$ to $\oint u_i(\rho u\cdot dS) $ but I just can't seem to get it.

I have found that there is an identity:

$$\rho u\cdot\nabla u + \rho u \nabla\cdot u = \nabla\cdot(\rho u u ) $$

from this Physics Forums thread, but I have no idea where it comes from.

and since mass is conserved, it becomes

$$\rho u\cdot\nabla u = \nabla\cdot(\rho u u ) $$

so I've basically focused my attention on trying to convert $\nabla\cdot(\rho u u ) $ to $\oint u_i(\rho u\cdot dS)$ but my question is how come one of the $u$ can be broken off and placed at the front with the subscript $i$ and why is it $(pu\cdot dS)$ and not $(puu\cdot dS)$ instead?

Answer 1

Answer to question1: ui uj, the product is a tensor of 9 components, so Gauss Divergence theorem for a tensor has to be used rather than the one you used for a vector. In this case, since the tensor is symmetric, ui can come out and dangle outside the divergence operator. The image from Kundu, Fluid Mechanics. !

Answer to question2: Look at page 35 of the turbulence book you mentioned, since ds is specified as a surface integral it is indeed integration over an area, and not over a line. Also the circular symbol on the integral specifies it is a closed integral, means the surface over which this integral is evaluated is closed, which is exactly what happens on a control volume. Rest of the derivation is same as you have derived. !

Answer 2

Before proceeding, let us prove the identity $\nabla\cdot(\vec{a}\vec{b}) = \vec{b}\cdot\nabla\vec{a} + \vec{a}\nabla\cdot\vec{b}$. The derivation becomes easy when we use the cartesian tensor form, \begin{equation} \frac{\partial}{\partial x_j}(a_ib_j) = b_j\frac{\partial a_i}{\partial x_j} + a_i\frac{\partial b_j}{\partial x_j} \end{equation} If we now put $\vec{a} = \rho\vec{u}$, $\vec{b} = \vec{u}$ and assume that $\rho$ is a constant then we have $\nabla\cdot(\rho\vec{u}\vec{u}) = \rho\vec{u}\cdot\nabla\vec{u} + \rho\vec{u}\nabla\cdot\vec{u}$. If the fluid is incompressible, then $\nabla\cdot(\rho\vec{u}\vec{u}) = \rho\vec{u}\cdot\nabla\vec{u}$. Thus, \begin{equation} \iiint\nabla\cdot(\rho\vec{u}\vec{u})dV = \iiint \rho\vec{u}\cdot\nabla\vec{u}dV \end{equation} The integral on the left hand side can be transformed to surface integral and \begin{equation} \iint \rho\vec{u}\vec{u}\cdot\hat{n}dS = \iiint \rho\vec{u}\cdot\nabla\vec{u}dV. \end{equation}