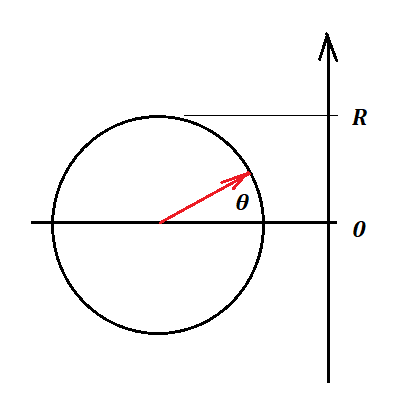
Can we consider $\omega$ (angular frequency) in equation of simple harmonic motion (SHM) as the angular velocity of the object in circular motion, when we see simple harmonic motion as projection of circular motion?
$\begingroup$
$\endgroup$
5
-
$\begingroup$ Short answer: yes, but if and only if the circular motion is uniform circular motion $\endgroup$– EagleCommented May 13, 2019 at 20:48
-
$\begingroup$ Then if it's correct, it means angular frequency w=2pie/T should be equal to angular velocity w=v/r of the particle executing shm $\endgroup$– Nikhil PantCommented May 13, 2019 at 20:56
-
$\begingroup$ Yes, you're right. $\endgroup$– EagleCommented May 13, 2019 at 21:06
-
$\begingroup$ Have you learned about rotations in phase space? $\endgroup$– Cosmas ZachosCommented May 13, 2019 at 22:09
-
$\begingroup$ In fact, this explains why the harmonic motion is sinusoidal, but not along any other periodic function. $\endgroup$– safesphereCommented May 14, 2019 at 6:39
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
3
Yes. The point on the circle with radius $R$ revolves around the centre, with angular velocity:
$$\omega=\dot{\theta}=\frac{\mathbf{d}\theta}{\mathbf{dt}}$$
The projection on the RHS axis, call it $y$, is:
$$y(t)=R\sin(\omega t+\phi)$$
where $\phi$ is the angle $\theta$ at $t=0$.
-
$\begingroup$ but do u really think the ω in (ω=θ) is equal to the ω in (y(t)=Rsin(ωt+ϕ)). basically my Que is can we really think the angular frequency in a shm as angular velocity of the object when we manipulate the motion as the shadow projection of circular motion (the the object is moving in a circular motion) $\endgroup$ Commented May 14, 2019 at 8:01
-
$\begingroup$ Please note: $\omega=\dot{\theta}=\frac{\mathbf{d}\theta}{\mathbf{dt}}$ $\endgroup$– GertCommented May 14, 2019 at 14:22
-
$\begingroup$ It's more an analogy. But mathematically the projection of the position vector (red) of a particle moving uniformly on a circle onto any axis corresponds exactly to the description of an SHO. $\endgroup$– GertCommented May 14, 2019 at 14:32