3
$\begingroup$

I'm very curious about inductors in general, and how similar they are to capacitors.

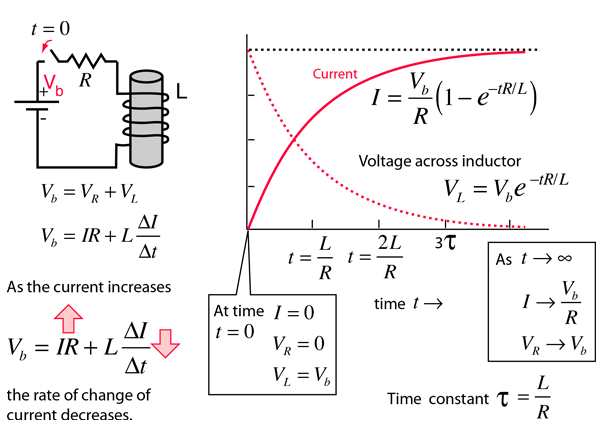
Top right formula

In the above picture the formula of current over time in an inductor with wire resistance $R$ is written.

I'm curious whether this formula works for superconductors. As I've been taught, superconductors have a resistance of exactly 0, and the formula is not mathematically defined at 0, so how do we define this state?

My professor told me that I have to calculate the limit approaching 0, but I found that doesn't make sense when resistance is exactly 0; So if someone could explain to me I would be thankful.

Edit: Thanks for all the answers they were all helpful in some way or another, but i chose the one i felt was most helpful.

Improve this question
$\endgroup$
1
  • $\begingroup$ Just as a general consideration, most (all) superconductors have a critical current beyond which they are no longer superconducting. This comes from the variations in the magnetic field generated by the current. and these variations disrupt the cooper pair flow. (So no ∞ current, not a problem) $\endgroup$
    – ElderDelp
    Commented Sep 27, 2022 at 20:18

4 Answers 4

Reset to default
1
$\begingroup$

Let us try as your professor suggested as lim R->0 the equation becomes 0/0 which is undetrmied so let's use L'Hospital's Rule. we get $$I(t)=\frac{V_b }L .t e^{-tR/L}=\frac{V_b.t}L \, .$$ Yes because an ideal inductor is short circuit for steady state. as you might know inductive reactance $X_L=j \omega L$ as it's DC, frequency is zero it's practically a short circuit since it violates Kirchhoff's voltage law hence a large current flows in the circuit.

Improve this answer
$\endgroup$
2
  • $\begingroup$ limiting R to 0 would give us I(t) = Vt/L which means the current would grow more as time passes and, unlike the original formula, there no limit for the current. This doesn't make much sense.. $\endgroup$
    – Septim
    Commented May 6, 2019 at 14:25
  • $\begingroup$ I added some explanation if still need more comment me in the comment section. $\endgroup$
    – Ch.Siva Ram Kishore
    Commented May 6, 2019 at 15:37
1
$\begingroup$

The case you presented in within the "regular" ohmic regime, for which the Ohm's law has the following formulation -

$$J=\sigma E$$ Here $J$ is current density, $\sigma$ is the conductivity (inverse of resistivity) and $E$ is the electric field (see Drude model for the intuitive derivation of it). Integration of this relation recreates the more popular version, $I=V/R$. Notice that this is a simplified relation, which doesn't hold in general but in certain parameters regime. Now, the meaning of this relation is interesting - the current is proportional to the force! The analogy from mechanics is that of terminal velocity of a mass subjected to a constant force. This is of course great deviation from Newton't law, where the acceleration is proportional to the force, and not the velocity.

A great advancement in understanding superconductivity was to recreate "Newton's law for currents" (which is one of the London equations), there the electric field (~the force) causing the current density to increase (~acceleration), $$\frac{\partial J}{\partial t}=\sigma E$$

Practically speaking, any physical circuit always has resistive parts (the battery, the wires at least), and even the measurement devices (ampermeter) are not ideal. Thus the finite resistance of the circuit will balance the voltage drop of the battery, and you will see similar properties.

Notice that superconductivity is a quantum phenomena, and classical explanations are at best good intuitive phenomenological models.

Improve this answer
$\endgroup$
1
  • $\begingroup$ What if we have a special setup where the wires are completely isolated and at superconductive state, and the voltage in it is coming from an external magnetic field(which we somehow make sure creates a constant voltage in the wire). How do you explain the voltage drop here? $\endgroup$
    – Septim
    Commented May 7, 2019 at 4:14
0
$\begingroup$

If you look at what happens to maximum (final) current as $R$ goes to zero, it increases without any limit. So if you connect superconducting coil to ideal source of voltage, current will increase until it is so high that it destroys the superconducting state in the coil. Then the coil resistance will massively increase in a short time and energy stored in the superconductor will turn to heat, possibly melt the coil. Superconductors can't carry too high currents.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Interesting, but given that literally any closed circuit has some self-inducting effect, does this mean any closed superconducting circuit will destroy itself over time? $\endgroup$
    – Septim
    Commented May 6, 2019 at 19:14
  • $\begingroup$ No, only those where current increases beyond the maximum value that the superconductor at hand can handle. If there is no hard source of voltage in the circuit, the current will not increase in such way. $\endgroup$
    – Ján Lalinský
    Commented May 6, 2019 at 19:17
  • $\begingroup$ Really helpful answer, but i still don't understand why we calculate current as resistance approaches zero. This suggests resistance in this circuit never truly becomes zero, even though superconductors are said to have exactly 0 resistance. $\endgroup$
    – Septim
    Commented May 7, 2019 at 4:08
  • $\begingroup$ It is possible to write down and solve the same kind of equation for an ideal coil without any resistance. The result is the same, the current increases without a limit. Superconducting coil is more complicated than ideal coil in that for high enough current, its resistance ceases to be zero. $\endgroup$
    – Ján Lalinský
    Commented May 7, 2019 at 10:45
0
$\begingroup$

Let's start from the initial equation $$V_b = IR + L \frac{dI}{dt} \, .$$ When the resistance is zero, the equation reduces to $$V_b = L \frac{dI}{dt} \, .$$

Integrating, we get $I = V_b t / L$ (assuming initial current was zero). There was no need to take any limits of $0/0$ form.

To keep the voltage constant, the current must change in such a way that the rate of change of current is constant, i.e. current is a linear function.

Improve this answer
$\endgroup$
2
  • $\begingroup$ So assuming voltage is constant, the current increases indefinitely? And according to the relation of current and energy in an inductor, that would mean infinite energy could be stored in almost any inductor in this state.. is that right? $\endgroup$
    – Septim
    Commented May 6, 2019 at 18:09
  • $\begingroup$ Yes. For an ideal battery that can keep supplying current endlessly and yet maintain a constant voltage. An ideal battery can supply endless energy. Proof: Connect a resistor to the battery (there is no other component like inductors). It will produce heat $V^2/R$ per unit time. Keep this circuit on for an infinite amount of time. $\endgroup$
    – Archisman Panigrahi
    Commented May 6, 2019 at 18:20

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.