Muon decays are almost always written as $$\mu^- \rightarrow e^-+ \bar\nu_e +\nu_\mu.$$
The reason given on wikipedia is that one of the product neutrinos of muon decay must be a muon-type neutrino and the other an electron-type antineutrino due to conservation of leptonic family numbers.
Does this mean that muons can't decay into quarks plus a muon-type neutrino, which can be lighter particles than the muons itself and with electric charge added up to -1? If they can't, why?
No, muons can't decay into quarks because quarks are confined; the final product cannot be quarks, but rather composite particles made of quarks, such as mesons and baryons. The lightest mesons are the pions, which are already heavier than the muon, so any such decay is forbidden by energy conservation.
On the other hand, the extremely heavy tau can and does decay to light mesons quite often, as you can see in the PDG entry.
Maybe my answer is non-mainstream physics (though I'm sure it's gonna be mainstream one day) and I give the answer nonetheless. It cán be enlightening, who knows?
As might be known, I'm a big fan of the Rishon Model, concocted up by the Israelian physicist Haïm Harari.
He sees quarks and leptons as composite particles made up out of just two (!) truly elementary particles, the T-rishon, and the V-rishon.
The muon is built up out of three anti-T-rishons (which each have an electric charge $-\frac 1 3$): $\bar T \bar T \bar T$.
Now if a $VVV$, a neutrino, in this case, a muon neutrino $\nu_{\mu^-}$ (each V-rishon carries an electric charge $0$) and a $\bar V \bar V \bar V$ appear as a virtual particle pair, the rishons can rearrange themselves (a $\bar T$ and a $\bar V$ interchange) into an anti-up-quark, $\bar u$ ($\bar T \bar T \bar V$), a down quark, $d$ ($\bar T \bar V \bar V$), and an muon neutrino ($VVV$). The two quarks form a meson, so we are left with a meson ($\pi^{-}$) and a muon-neutrino. Check that the number of ${\bar T}$'s, $V$'s, and ${\bar V}$'s are the same on both sides:
$$\bar T\bar T\bar T+ VVV+ \bar V \bar V\bar V\rightarrow \bar T\bar T\bar V+\bar T\bar V\bar V+VVV,$$
which reads in the standard formulation as:
$$\mu^{-}\rightarrow\bar u +d+\nu_{\mu}.$$
However, as knzhou wrote, the energy of a muon is too small to produce a pion and a muon-neutrino.
But if we take the third generation tau particle (which, according to the model, is also built up out of three anti-T-rishons, $\bar T \bar T \bar T$) this tau will be heavy enough to produce a pion and an anti-tau neutrino by the mechanism described above.
Look at this picture, the standard Feynman diagram of tau decay:
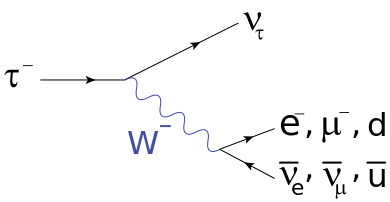
As can be seen, the decay is induced by the weak interaction, which in the Rishon Model is considered as a residue force, just as the "old" strong force (conveyed by massive pions, just as the $W^{+/-}$ and $Z^0$ are massive) is now considered as a residue force of the color force (the force of which the weak interaction is a residue is called the hyper-color force). The $W^-$ is in the Rishon Model built up out of three anti-T-rishons and three anti-V-rishons: $\bar T \bar T\bar T \bar V \bar V \bar V$ ($Z^0$ is built up out of three $V$'s and three $\bar V$'s: $\bar V \bar V \bar V VVV$ where I referred to). So you can just as well say that the tau ($\bar T \bar T \bar T$) becomes part of a virtual $Z^0$ ($\bar V \bar V \bar V VVV$) after which the shown outgoing particles can be produced.
