Flow equation for system of coupled tanks

Question

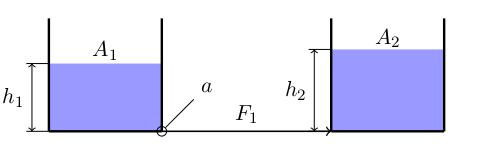
Consider the following system of interconnected tanks:

The two tanks have cross-section areas $A_1$ and $A_2$ respectively and the levels of the liquid are denoted by $h_1$ and $h_2$ respectively.

The liquid can run from one vessel into the other through a tube of cross-section area $a$, which is significantly smaller than $A_1$ and $A_2$.

Let us denote the density of the liquid by $\rho$ and the gravitational acceleration by $g$.

I need to derive a dynamical model of this system that describes the evolution of $h_1$ and $h_2$ and I see in various publications (example) that

$$ F_1 = \rho a \sqrt{2g(h_1-h_2)}.\tag{*} $$

I would like to use Bernoulli's principle to derive this formula. I will assume that the head loss in the pipe due to friction is negligible.

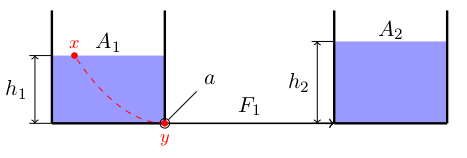
From Bernoulli's principle between a point $x$ on the surface of the first tank and a point $y$ at its exit

we have

$$ P_{atm} + \rho g h_1 = P_y + \frac{1}{2}\rho v_y^2\tag{1a} $$

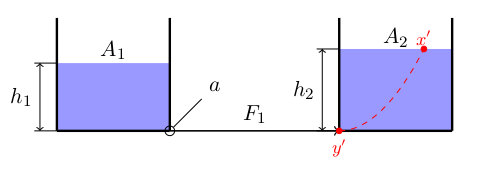
Similarly, in Bernoulli's principle in the second tank gives

$$ P_{atm} + \rho g h_2 = P_{y'} - \frac{1}{2}\rho v_{y'}^2\label{1b}\tag{1b} $$

I am not sure about Equation \eqref{1b} - I used the negative sign because I used the convention that a positive $v_y$ means that the liquid flows from the first tank into the second one, so the second tank gains kinetic energy.

Question 1. Can you please clarify whether this is correct?

From point $y$ to $y'$, Bernoulli's equation gives

$$ P_{y} + \frac{1}{2}\rho v_{y}^2 = P_{y'} + \frac{1}{2}\rho v_{y'}^2\tag{1c} $$

Because of the mass balance equation between the two ends of the tube (assuming incompressible flow), it is $v_y = v_{y'}$.

The mass balance between the two tanks is

$$ \rho A_1 \dot{h}_1 + \rho A_2 \dot{h}_2 = 0\tag{2}. $$

Lastly, we know that

$$ F_1 = \rho a v_y, $$

so it suffices to show that $v_y = \sqrt{2g(h_1-h_2)}$.

Question 2. I have tried to combine Equations (1a-1c) and (2) to derive Equation (*), but did not make it.

Answer 1

the speed changes with time as the levels change

This is a crucial bit of information because it may be tempting to believe the flow will stop when the tank levels become equal but due to inertia this is not the case. Instead the system will enter a simple harmonic oscillation.

Firstly a bit of tedious algebra. Determine the equilibrium level $h_0$ (both tanks at same level), from total volume considerations.

$$h_1A_1+h_2A_2=h_0(A_1+A_2)$$ $$h_0=\frac{h_1A_1+h_2A_2}{A_1+A_2}$$ Now find the volume $V$ above the equilibrium level $h_0$ for any $h_1$,$h_2$: $$V=(h_1-h_0)A_1+(h_0-h_2)A_2$$ with: $$h_2=\frac{h_0(A_1+A_2)-h_1A_1}{A_2}$$ $$V=(h_1-h_0)A_1+(h_0-\frac{h_0(A_1+A_2)-h_1A_1}{A_2})A_2$$ $$V=(h_1-h_0)A_1+h_0A_2-h_0(A_1+A_2)+h_1A_1$$ $$V=2h_1A_1-2h_0A_1$$ Its mass $m$ is with density $\rho$: $$m=\rho(2h_1A_1-2h_0A_1)$$ So the net force acting on the total mass $M$ is: $$mg=\rho g(2h_1A_1-2h_0A_1)$$ The Equation of Motion, with $M$ the total mass, is: $$Ma+\rho g(2h_1A_1-2h_0A_1)=0$$ Where: $$a=\frac{\mathbf{d^2}h_1}{\mathbf{d t^2}}=\ddot{h_1}$$ Use the following substitution: $$y(t)=\rho g(2h_1A_1-2h_0A_1)$$ $$\dot{y}=2\rho gA_1\dot{h_1}$$ $$\ddot{y}=2\rho gA_1\ddot{h_1}$$ $$\ddot{y}+\frac{2\rho gA_1}{M}y=0$$ Set: $$\omega^2=\frac{2\rho gA_1}{M}$$ $$\Rightarrow y=y_0\cos(\omega t+\phi)$$ Where at $t=0$: $$y_0=\rho g(2h_1(0)A_1-2h_0A_1)$$ and if $\dot{y}(0)=0$, then $\phi=0$.

Note that this derivation only works for completely inviscid liquids. Where there's viscosity, there are friction losses and thus damping.