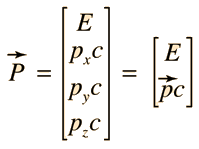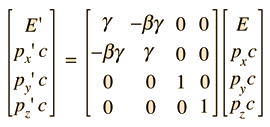7
$\begingroup$

(a) When there is a red or blue shift, is there an actual change in the energy of the photons or not?

(b) If there are two observers, one moving toward the light source and one away from it, they would see different red/blue shifts of the same set of photons from the same source, so that gives me a clue that actually the energy of the batch of photons is not changing, this is just some apparent effect that we are seeing. Then how can we say for both observers that the energy of the photons is proportional to the frequency? (the frequency observed by both observers is different, but it is the same batch of photons from the same light source presumably having the same energy)

(c) Assuming light is emitted by a bandgap transition, and the emitted photons have energy equal to the bandgap, then intuitively speaking, why does the motion of the emitter alter that energy?

I can understand how a number of cycles of waves can get compressed or expanded into a smaller or larger space depending on the motion of the emitter and hence change the wavelength. I can also understand how, if a gun is firing a bullet, and the gun is mounted on a fast-moving train, then the velocity and KE of the bullet will change as a result.

However, the photons travel at fixed velocity, and being massless, have no KE resulting from mass. So how is it possible that the physical motion of the emitter "relative to the observer" translates into a different energy of the photons than the bandgap energy from which they were emitted?

(d) If it is the observer that is accelerating towards the light source (e.g. the observer is in an accelerating rocket ship), then why would the energy of the distant photons change or why would the observer see a continuous change in red/blue shift?

Improve this question
$\endgroup$
5
  • $\begingroup$ It is preferable to ask one question at a time. To answer a) and b), E=hf. So yes the energy changes. $\endgroup$
    – my2cts
    Commented Mar 22, 2019 at 10:10
  • $\begingroup$ I think is all about duality. Propagation as a wave and interaction as a photon. More formal answer needed, of course. It is always tricky to transition from a train of waves to single photons, there are already questions different from yours but boiling to the same point. $\endgroup$
    – Alchimista
    Commented Mar 22, 2019 at 10:12
  • $\begingroup$ Not all observers will measure the same energy of the photon, it doesn't lose energy until it collides with something else. $\endgroup$
    – user6760
    Commented Mar 22, 2019 at 10:12
  • $\begingroup$ The question is, if the photon has energy X, why would different observers measure it as having different energy? $\endgroup$
    – Khushro Shahookar
    Commented Mar 22, 2019 at 10:28
  • $\begingroup$ @KhushroShahookar, different observers in different frames of reference. $\endgroup$
    – Solomon Slow
    Commented Mar 22, 2019 at 12:05

2 Answers 2

Reset to default
3
$\begingroup$

You ask why it is that the energy of the photon/wave packet is not the same in different frames of reference. I cannot offer a good explanation for the why but I think the following might "fix" the intuition where the question seems to originate.

Imagine an object just standing there in vacuum. Its energy is zero. But what would another observer (moving with constant velocity with respect to it) think its energy is? Well, not zero. The situation of a photon (i.e., a massless object which is not just standing there) is not the same, sure, but still the same question applies to both situations: Why would its energy be the same in reference frames moving at different velocities anyway? It simply would not.

I'll speculate that perhaps you are thinking "Energy is a conserved quantity; it must be the same." If that is the case, you must remember that implicit or explicit in such statements of invariance there is a transformation in mind. The transformation relevant to your question is the one that converts quantities from one (inertial) observer's reference frame to another's. Namely the Lorentz transformation. And energy of a particle (or the sum of energies of a bunch of particles) does not remain the same under this transformation. A quantity made out of both the energy and the momentum does, but this is perhaps not the place to dig into that.

If you would still appreciate an itemized answer, in light of the discussion above:

(a) There is an actual change in the energy of the photons.

(b) The energy of the batch of photons is changing. The intuition you voice in your parenthetical remark about the same batch of photons having the same energy is flawed. That's how intuition is sometimes.

(c) I would avoid the bullet analogy because the addition of velocities there does not apply to light at all. So it would only increase confusion. However your contemplations about the wavelength getting stretched or compressed are quite well-placed. In your band-gap transition example (which is a good one), it's not so much the motion of the emitter that affects the energy of the emitted photon (because the emitter is stationary according to its own reference frame!), rather it's the motion of an observer relative to it. As you pointed out, the wavelength of the oscillation will be different in that observer's frame. (This is true even for slow waves, not just photons, but the relation of the new wavelength to the old one is a bit more sophisticated in the latter case.)

(d) I'm not sure I understand this one. But perhaps once it fixes itself once you are not worried about the energy of a photon being different in reference frames with different velocities.

Improve this answer
$\endgroup$
8
  • $\begingroup$ It is virtually impossible to believe that a body can have different KEs when viewed from different frames of reference. Suppose the observer is in a rocket ship and is accelerating towards an object with no external force acting on it, he would know that he is increasing his own KE, but he would see the other object's KE increase with no source of energy being supplied to it. How is that possible? $\endgroup$
    – Khushro Shahookar
    Commented Mar 22, 2019 at 15:58
  • $\begingroup$ @KhushroShahookar KE is not an absolute thing, it is frame dependent. If the object is moving with respect to you, you can harvest energy from its KE (perhaps with a rope and a generator). If you are both moving at the same speed, there is no relative motion and no energy is there for you to harvest. $\endgroup$
    – BowlOfRed
    Commented Mar 22, 2019 at 16:03
  • $\begingroup$ Also, suppose a light source is on an accelerating rocket ship, then its dopler shift is continuously increasing, as its velocity increases relative to an observer moving without any acceleration. Now the mere motion of the light emitter does really change the energy of the emitted photons, beyond the bandgap due to which they are emitted. Why? I am using acceleration (which can be detected in absolute terms) to prove that it is really the light source's motion that contributes to change in energy, instead of anything else. $\endgroup$
    – Khushro Shahookar
    Commented Mar 22, 2019 at 16:04
  • $\begingroup$ If KE is frame-dependent and not absolute then energy is not conserved. In the above example, the observer accelerating in a rocket towards an object with no force acting on it, the observer observes that the observed object is increasing in KE, (at least relative to the observer the object seems to increase its speed), although it has no source of energy to provide this increasing KE. True the observer can detect in absolute terms that he is accelerating and the object is not, but based on the speed of the object relative to himself he does observe increase in objects KE with no power source $\endgroup$
    – Khushro Shahookar
    Commented Mar 22, 2019 at 16:18
  • $\begingroup$ @KhushroShahookar, you are thinking of (kinetic) energy as absolute; as though something has a "true" value of it. But no inertial frame is more true than another, and since observed velocities depend on the frame, so does the energy. As for when your frame itself is accelerating (noninertial), a system viewed in that frame is not supposed to have a conserved energy. In fancy terms, the Hamiltonian depends explicitly on time. Also, your example of accelerating the emitter doesn't make the effect special to the acceleration of the emitter: the effect remains if the observer is accelerating. $\endgroup$
    – Cem Yolcu
    Commented Mar 22, 2019 at 16:25
1
$\begingroup$

The photon is a relativistic elementary particle and is described by a four vector:

fourv

four vector

The length of this 4-vector is the rest energy of the particle. The invariance is associated with the fact that the rest mass is the same in any inertial frame of reference.

E is the total energy, of which the kinetic part is the one carrid by the momentum vector. The only invariants in frame changes is the mass, the vector itself changes according to the Lorentz transformation

lorenz

which depends on the velocity. So the mass is the only invariant to velocity changes, the momentum vector changes to p', and for the photon this means that the energy changes , according to the equation for the mass.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.