In a lot of plots they use dimensionless quantities, why really we don't let quantities in their physical dimension and plot the curves normally.
$\begingroup$
$\endgroup$
1
-
$\begingroup$ In my opinion, the dimensionless forms are special. Once you have a plot which is dimensionless on both axes, the line or curve on that plot describes all combinations of the variables that were involved in the dimensionless quantities on each axis. And as pointed out below, there will not be a requirement for multiple lines or curves on such a plot ... one line or curve tells you everything that you need to know. $\endgroup$– David WhiteCommented Mar 19, 2019 at 15:33
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
The reason why this is done is because dimensionless data usually follows a single curve rather than multiple curves for different values of the parameters. This is nice because the figures become nicer and the data more organized.
This dimensional reduction of data occurs because, by the Buckingham-$\Pi$ theorem, there are fewer combined dimensionless parameters than there are individual dimensional parameters. These dimensionless parameters give more information about the underlying phenomena than the dimensional parameters do by themselves.
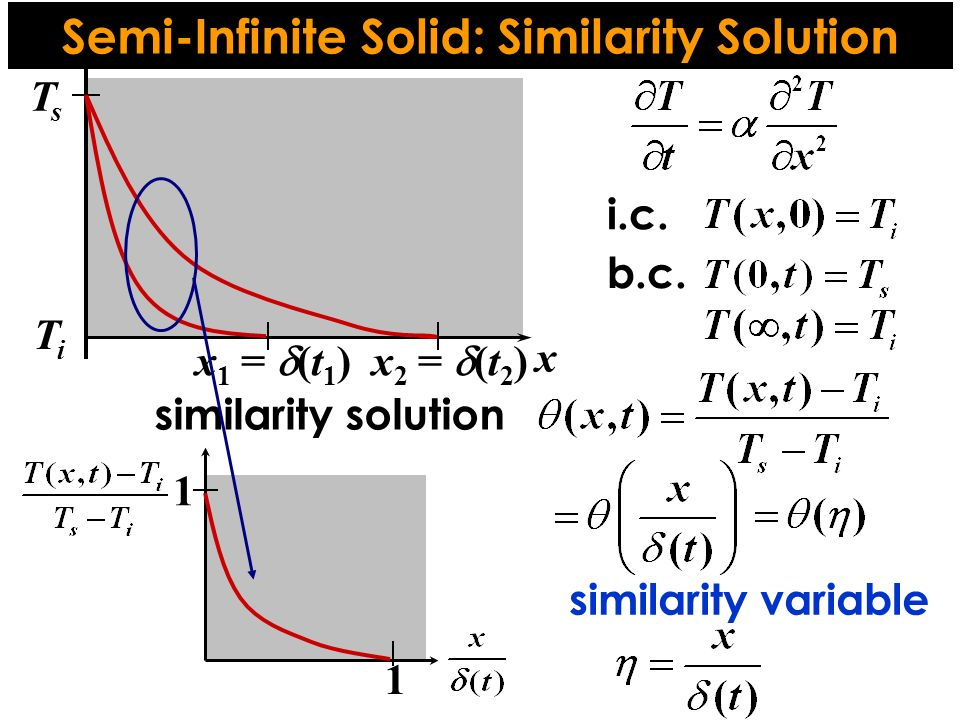
Let's take an example; below is a figure of transient heat transfer through a semi-infinite solid. If you look at the top figure it shows that at different times the cross-sectional temperature profiles are different; this makes sense because the solid is heating up. However if you properly scale the temperature and spatial coordinate and make them dimensionless: $$\theta = \frac{T-T_i}{T_s-T_i} \quad \eta = \frac{x}{\sqrt{4\pi t}}$$ then all profiles (for limited short time scale) collapse onto a single curve, the figure below.
I think this single curve in general is much more useful because it no longer depends on the values of the individual parameters (e.g. initial and wall temperatures) but on a dimensionless number which i can replicate if i ever have a similar situation but with different values for the initial and wall temperatures. I can then still apply this graph.
In my opinion most scientific figures should be presented in dimensionless form. Then it also doesn't matter anymore what units system the author worked in; he can use imperial units and i can work in metric units, it doesn't matter as long as the combination of parameters into dimensionless parameters is the same value.
$\begingroup$
$\endgroup$
Dimensionless constants have a special significance in physics. This is well described in the answers to the question Dimensionless Constants in Physics.
Having said this, whether or not you use dimensionless quantities in data analysis is to some extent a matter of taste. In my time as an industrial colloid scientist I generally didn't manipulate plots to be of dimensionless quantities, but then this was very much applied physics. If I were studying fundamental issues, e.g. at CERN, I might be more inclined to work with dimensionless quantities.
answered Mar 19, 2019 at 7:41