Optical convolution processor

Question

I was reading Chapter 4, on Fourier Optics, of the course notes on Photonics by Roel Baets and Günther Roelkens (Ghent University, 2009).

I am struggling with the following section:

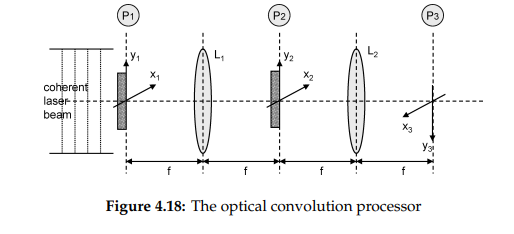
4.3.5 Optical convolution processor

It is possible to realize, in an optical set up, a convolution between two functions $$ \left|\int \int_{-\infty}^{+\infty}g (ξ, η) h (x − ξ, y − η) dξdη\right| \tag{4.129} $$ The set up is shown in figure 4.18. The "input"-function $g$ is realized as a mask with transmittance function $g(x_1, y_1)$ and placed in plane $P_1$, which is the first focal plane of a lens $L_1$. It is normally illuminated with a monochromatic plane wave. In the second focal plane $P_2$ of this lens one obtains the Fourier transform $k_1 G(−x_2/λf, −y_2/λf)$ of $g$ (in which $k_1 =$ complex constant). In this plane $P_2$ one puts a second mask with transmittance function $$ t(x_2, y_2) = k_2 H\left(\frac{-x_2}{\lambda f},\frac{-y_2}{\lambda f}\right), \tag{4.130} $$ with $$ H (fx, fy) = F_{2D} \left\{h (x, y)\right\} \tag{4.131} $$ Behind this mask, the field is proportional to $G.H$ ; hence in the focal plane $P_3$ of the second lens $L_2$ one finds the following irradiance: $$ I (x_3, y_3) = K \left|\int \int_{-\infty}^{+\infty}g (ξ, η) h (x_3 − ξ, y_3 − η) dξdη\right|^2 \tag{4.132} $$ This kind of convolution processor can be practically realized by inserting in the planes $P_1$ and $P_2$ a LC-SLM (Liquid Crystal Spatial Light Modulator). This is a two-dimensional transmission screen, with which one can realize an arbitrary two-dimensional function with the help of a computer. In plane $P_3$ one puts a CCD (Charged Coupled Device) Camera which transforms the optical information in an electrical one. An advantage of this processor is the fact that the "calculations" are done immediately (the speed is only limited by the input and output speed of the LC-SLM and CCD). Disadvantage (as compared to the calculations with a digital computer) is the analog character of the calculations, with its inaccuracy, the non-linearity in the LC-SLM and CCD, and also the aberrations in the lenses. Moreover there is also a technological problem: the function H is usually a complex function. This implies that in plane $P_2$ one needs an SLM in which not only the amplitude, but also the phase transmittance should be simulated electronically; and this is a very difficult technological problem

But I do not agree with its conclusion (I think it is wrong). Indeed, we have (I use a slightly different notation, hope it's clear): $$U(x,y,2f)=const \ F[g(x_{1};y_{1})]_{(\frac{kx}{f};\frac{ky}{f})}$$ and after the second mask: $$U(x,y,2f)=const \ F[g(x_{1};y_{1})]_{(\frac{kx}{f};\frac{ky}{f})} F[h(x_{1};y_{1})]_{(\frac{kx}{f};\frac{ky}{f})}$$ Finally: $$U(x,y,4f)=const \ F(F[g(x_{1};y_{1})]F[h(x_{1};y_{1})])_{(\frac{kx}{f};\frac{ky}{f})}$$ And so I can't say that $U(x,y,4f)$ is the convolution product of $g$ and $h$ because to say it, it should be: $$U(x,y,4f)=const \ F^{-1}(F[g(x_{1};y_{1})]F[h(x_{1};y_{1})])_{(\frac{kx}{f};\frac{ky}{f})}$$ not?

Answer 1

Imagine a slightly different optical setup, in which light representing the product of Fourier transforms which forms at P is simply reversed and sent back through lens L1 to form an image at P1. Since L1 forms at P2 the Fourier transform of whatever is at P1 for light passing from left to right, it forms the at P1 the inverse Fourier transform of whatever is at P2.

Now notice that L1 and L2 are the same. That means the right-hand half of your diagram is equivalent to the second step of the different optical setup described above. So, light passing from P2 to P3 through L2 forms the inverse Fourier transform of whatever is at P2.

Answer 2

The problem seem to be the difference between the Fourier transform and the inverse Fourier transform. In optics, the Fourier transform is readily implemented with a lens. However, in the definition of the inverse Fourier transform, the only thing that is different is a sign change in the exponent of the Fourier kernel. In the optical setup, that sign change corresponds to a rotation of the axis on the transverse output plane. That is why a 4f system reproduces an image but with a 180 degree rotation. Therefore, the output of such a 4f system would be the 180 rotated image of the convolution of the input image and the Fourier transform of any transmission function placed in the filter plane between the two lenses.

Best is to work through it long hand, putting all the fancy notation aside until you understand what is happening.