Snell's law of refraction at the interface between 2 isotropic media is given by the equation: \begin{align} \tag{1} n_1 \,\text{sin} \,\theta_1 = n_2 \, \text{sin}\,\theta_2 \end{align}
$\qquad$ where $\theta_1$ is the angle of incidence and $\theta_2$ the angle of refraction. $n_1$ is the refractive index of the optical medium in front of the interface and $n_2$ is the refractive index of the optical medium behind the interface.
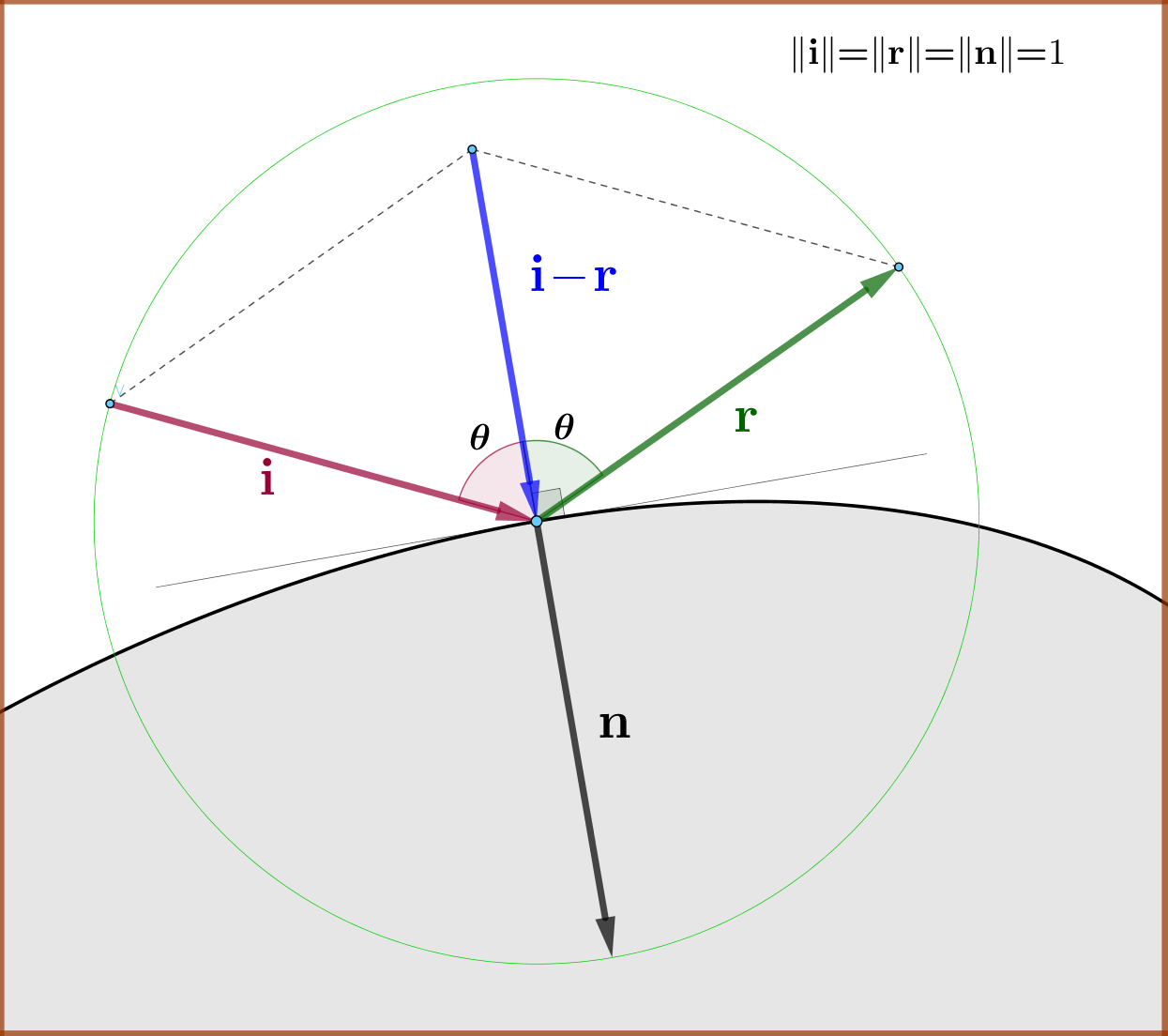
Eq.(1) can be expressed in vector form as \begin{equation}\tag{2} n_1(\textbf{i} \times \textbf{n}) = n_2 (\textbf{t} \times \textbf{n}) \end{equation} $\qquad$ where $\textbf{i}$ and $\textbf{t}$ are the unit directional vector of the incident and transmitted ray respectively. $\textbf{n}$ is the unit normal vector to the interface between the two media pointing from medium 1 with refractive index $n_1$ into medium 2 with refractive index $n_2$. Similarly $\textbf{r}$ is the reflected ray vector.
How can the equation \begin{align}\tag{3} \textbf{t} = \mu \textbf{i} + n\sqrt{1- \mu^2[1-(\textbf{ni})^2]} - \mu \textbf{n}(\textbf{ni}) \end{align} be used to derive the equation \begin{equation}\tag{4} \textbf{n} = \dfrac{\textbf{i}-\textbf{r}}{\sqrt{2[1-(\textbf{i}\textbf{r})]}}? \end{equation}
$\qquad$Here $\mu = \dfrac{n_1}{n_2}$ and $\textbf{n}\textbf{i}= n_{\text{x}} i_{\text{x}} + n_{\text{y}}i_{\text{y}} + n_{\text{z}} i_{\text{z}}$ denotes the dot (scalar) product of vectors $\textbf{n}$ and $\textbf{i}$.
In Ref.[1] it says that from Eq.(3) follows \begin{align}\tag{5} \textbf{r} = \textbf{i} - 2\textbf{n}(\textbf{n}\textbf{i}) \end{align} By simple modification \begin{align}\tag{6} \textbf{n} = \dfrac{\textbf{i}-\textbf{r}}{2(\textbf{n}\textbf{i})} \end{align} It says that "...by calculating the dot products of vector $\textbf{n}$ with both sides of Eq.(6), one can express the dot product $(\textbf{n}\textbf{i})$ in the form as shown in Eq.(4)" which I can't follow )=
Could someone explain how equation (4) is derived?
References:
- Antonín Mikš and Pavel Novák, Determination of unit normal vectors of aspherical surfaces given unit directional vectors of incoming and outgoing rays: comment, 2012 Optical Society of America, page 1356