I'm considering the muon neutrino/anti-quark interaction with the nucleons of ice in the IceCube experiment. The purpose is to decide which antiquarks are $\bar{q}$ and $\bar{q}'$. I have the following process: $\nu_\mu+\bar{q}\rightarrow \mu^-+\bar{q}'$. Since we are dealing with nucleons, I suppose in this case anti-nucleons, the $q's$ can only be either $\bar{u}$ or $\bar{d}$. In order to have charge conservation, I've drawn the following:
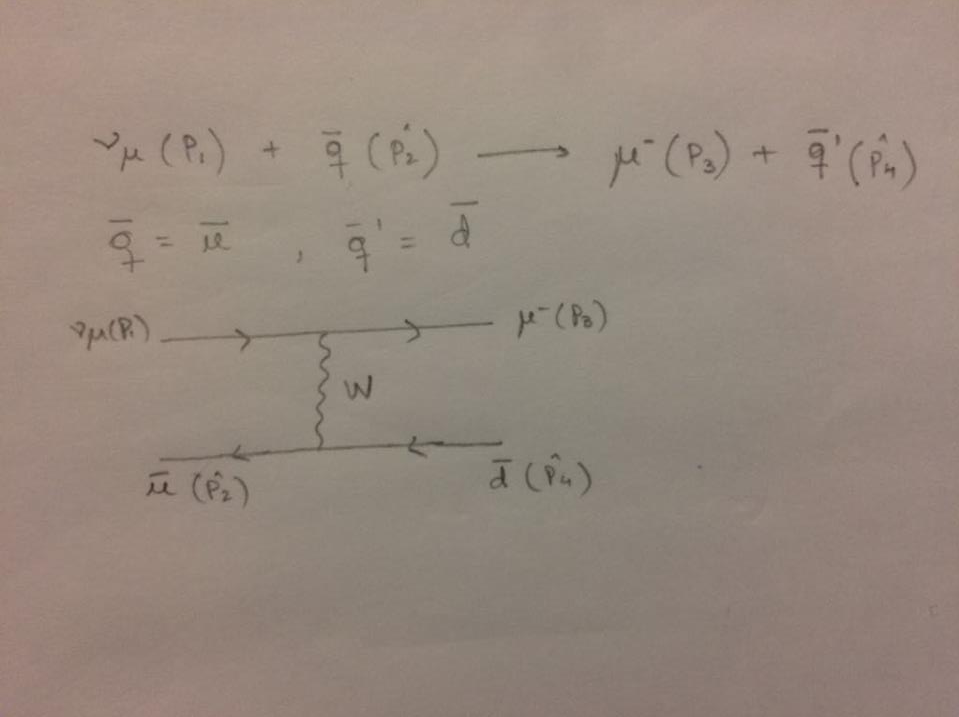
My problem concerns the $W$ boson. If I assume that the $\bar{u}$ decays into a $W$, the latter has to necessarily be a $W^+$ boson, in order for the $\bar{u}$ change its flavor to $\bar{d}$. However, the $\nu_\mu$ won't turn into muon by absorbing a +1 charged boson, correct? It had to be a $W^-$ boson then. However, if $\bar{u}$ is to transform into a $\bar{d}$, then it can't be a $W^-$ boson, right? Hence, my confusion. (vice-versa if it were the muon neutrino emitting the muon).
