How far can water rise above the edge of a glass?

Question

When you fill a glass with water, water forms a concave meniscus with constant contact angle $\theta$ (typically $\theta=20^\circ$ for tap water):

Once you reach the top of the glass, the water-air interface becomes convex and water rises up to a height $\Delta h$ above the edge of the glass, allowing you to fill the glass beyond the naive capacity $\pi r^2 h$:

So when getting myself a glass of water, I came to wonder exactly how much this increases the capacity of a glass, and what physical constants are involved.

My intuition would be that for a very large glass, $\Delta h$ converges to a constant so that the effective water capacity of the glass grows like $\pi r^2 (h+\Delta h)$ (to make things simple I'm assuming that the glass is very thin: $\Delta r\ll r$). Perhaps such a constant depends on the precise shape of the rim of the glass. But if not, perhaps it is a constant multiple of the capillary length?

So, what can we say about $\Delta h$, the "rim contact angle" $\alpha$, or the shape of the water-air interface when the glass is filled at maximum capacity?

Answer 1

As is given in Jamie's answer I'll assume the surface is a revolution about $r=0$, that the mean curvature is proportional to the pressure difference, and that the radius of the cup is much larger than the inverse of this mean curvature. In this case the mean curvature can be specified as $$ K_m = \frac{r''}{2(1+r'^2)^{\frac32}}$$

As in Jamie's answer the Young-Laplace Equation and hydrostatic pressure give $$ 2\,\gamma\,K_m = \Delta P = -\rho\,g\,z$$ Placing the origin at the surface and positive z in the direction of gravity.

Combining yields

$$ -\rho\,g\,z = \frac{\gamma\,r''}{(1+r'^2)^{\frac32}}$$

Substituting $q=r'$ yields a first order differential equation

$$ -\rho\,g\,z = \frac{\gamma\,q'}{(1+q^2)^{\frac32}}$$

Integrating

$$ -\frac12\,\rho\,g\,z^2 = \frac{\gamma\,q}{\sqrt{1+q^2}}+C$$

We know that at the very top of the water the surface is flat which would correspond to $q=r'=\infty$ this condition is gives $C=-\gamma$

$$ z= \sqrt{\frac{2\,\gamma}{\rho\,g}(1-\frac{q}{\sqrt{1+q^2}})}$$

Now since $q=r'=tan\,\alpha$ where $\alpha$ is described in the question, $z$ simplifies to

$$ z= \sqrt{\frac{2\,\gamma}{\rho\,g}(1-sin\,\alpha)}$$

Which is indeed the formula given in John Rennie's answer.

So now the question is what alpha to use. I propose that the water will continue expanding around the curved lip of a glass maintaining its contact angle until the point at which going farther along the lip would lower the top of the surface according to the above equation as at that point the surface would be unstable. This depends on the curve of the lip of the glass $r_l$.

If the water edge on the lip of the glass is at polar coordinate $\phi$ and the liquid has a contact angle $\theta$, then $\alpha=\phi-\theta$, and my total height $h$ will be given as $$ h=\sqrt{\frac{2\,\gamma}{\rho\,g}(1-sin(\phi-\theta))}+r_l\,(sin\,\phi-1) $$

Unfortunately this does not have a closed form maximum over theta, but we can see that for small values of $r$ the maximum will be when $\phi\lt 0$. This is not physical as the liquid would start creeping down the side of the glass and become unstable first. We can solve for the value of r at which this occurs $$r_l=cos\,\theta\sqrt{\frac{\gamma}{2\,\rho\,g\,(1+sin\,\theta)}}$$ For any lip radius under this value the maximum liquid height would be $$ h=\sqrt{\frac{2\,\gamma}{\rho\,g}(1+sin\,\theta)}-r_l $$

For water this radius calculates out to about $1mm$ and for a glass with a very small radius the height calculates out to about $4mm$ a bit higher than I've managed, but not unreasonable for a theoretical upper bound.

For glasses with a larger radius the maximum height can be solved numerically. Here's a plot.

And the corresponding "lip contact angles"

Answer 2

I experimented with my tea cup, a nice long cylinder.

The water was slightly climbing the walls making a concave surface. When it reached the edge I dripped water until the outer edge became convex and the water surface is almost a dome, though I only see the curvature at the rim where the water does not flow, displaying surface tension (both to the water and the ceramic).

I am shining a flashlite and the reflections seen are on the water. The curvature at the right is on water. It retained its shape after it overflowed ( I was dripping the water). Radius of cup 3.5cm, height of water about 1mm .

Answer 3

I can't answer your question because it depends on the shape of the rim, however I can answer a related question that should be easily adaptable to your problem.

If you have a puddle of water on a flat surface the thickness of the water film, $h$, is given by:

$$ h = \sqrt{ \frac{2\gamma_{al}(1 - cos\theta)}{g\rho} } $$

where the variables have their usual meanings: $\gamma_{al}$ is the air/liquid surface tension, $\theta$ is the contact angle, $g$ is the acceleration due to gravity and $\rho$ is the density of the liquid.

I think that if the rim of the glass has a semicircular cross section then this will give the maximum height of the liquid above the glass, and will apply when the edge of the liquid is at the top of the rim i.e. the point where the glass surface is horizontal.

Answer 4

I've been banging my head against this all day long, without getting to a real final answer, but I did get some progress...

Across the air-liquid boundary there is a pressure difference given by the Young-Laplace Equation:

$$\Delta p = 2 \gamma K_m,$$

where $K_m$ is the mean curvature of the surface. Assuming the interface is a surface of revolution, $z = z(x)$, with $x$ the radial coordinate, the mean curvature comes out to be:

$$K_m = -\frac{1}{2\sqrt{1+z'^2}}(\frac{z'}{x}+\frac{z''}{1+z'^2}).$$

This is of course highly intractable, so you usually hope for the slope $z'$ to be small, so that $z'^2$ is negligible, so that you can get away with the much simpler approximation

$$K_m \approx -\frac{1}{2}(\frac{z'}{x}+z'').$$

On the air side of the free surface, you have the constant atmospheric pressure, $p_0$, while on the other side there will be a hydrostatic pressure distribution, $p_1-\rho g z$, so

$$\Delta p = p_1 - p_0 -\rho g z.$$

Overall the shape of the free surface is governed by the equation

$$z'' + \frac{z'}{x} -\frac{1}{\lambda^2} z= \frac{p_0 - p_1}{\gamma},$$

where $\lambda = \gamma / \rho g$ is the capillary length. Now, taking the capillary length as the unit of distance, the above simplifies to

$$z'' + \frac{z'}{x} - z= \frac{p_0 - p_1}{\rho g}.$$

If the sign on the $z$ above where a plus, the above could be converted, choosing a suitable origin for z, into a Bessel equation of order $0$, but I am pretty sure the sign is correct, so no luck there.

But if you look at an actual glass of water filled to the brim, you will see that most of the bending of the surface happens close to the border, while the central region is mostly flat. So if $z'$ is only large when $x$ is much larger, the last equation simplifies to

$$z'' - z= \frac{p_0 - p_1}{\rho g},$$

and if the central portion is perfectly flat, then there will be no pressure difference there, an $p_1=p_0$ if the origin of $z$ is set at the level of water in the center point, so

$$z'' = z,$$

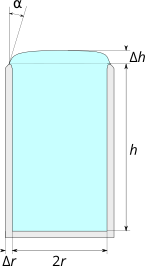
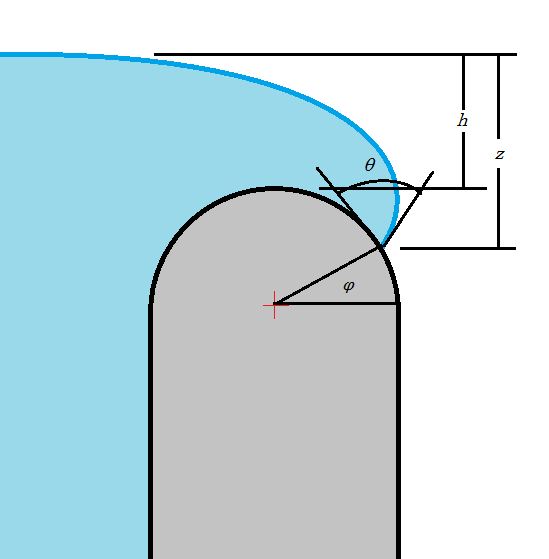
with boundary conditions $z(0) = 0$ and $z(r)=\tan \alpha$, where we still need to figure out what $\alpha$ is, more on this later.

The solution to the above equation is

$$z = \tan \alpha \frac{e^x -e^{-x}}{e^r -e^{-r}},$$

and the difference between the center point and the border is $\tan \alpha$ measured in capillary length units, or alternatively

$$\Delta h = \sqrt{\frac{\gamma }{\rho g}}\tan \alpha.$$

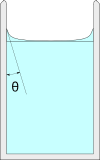
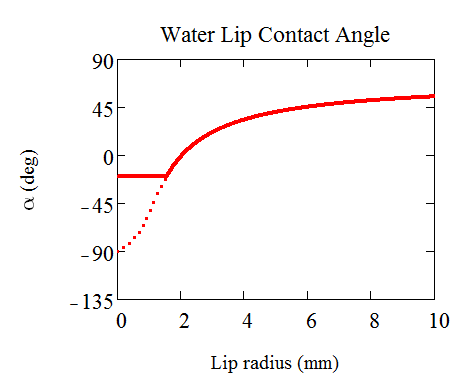
So what value does $\alpha$ take? In a cylindrical container, as Paul indicates, $\alpha$ is $\pi/2-\theta$, where $\theta$ is the contact angle, and the center of the glass is actually below the borders. But when you fill a glass to its rim, the rounded nature of this starts bending the outside borders of the water, eventually making $\alpha$. If we suppose contact is happening at the highest point of the rim, then $\alpha$ is the contact angle, only negative, and the center will be $\sqrt{\gamma / \rho g} \tan \theta$ above the rim.

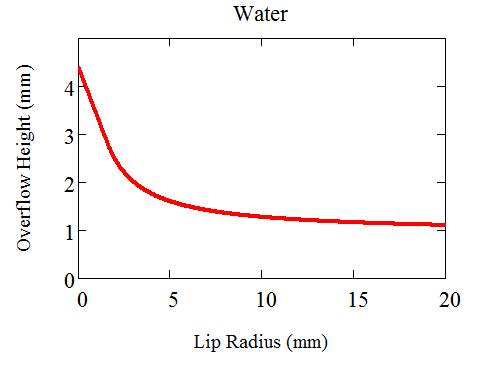
Of course the water may go beyond the highest point of the rim, but I am not sure of how far it can go before everything becomes unstable and you get spilling...

Answer 5

The answer lies in surface tension. If one puts water into a uniform cylinder and looks at the air-water interface at the edge where the water comes into contact with the cylinder, one will see that the water creeps up the glass a small amount. This is because the water "wets" the cylinder.

If the water does not wet the cylinder the water will be depressed at the wall.

The common case is wetting. In the situation here the cylinder can be filled until the water is high enough for the "creep" to reach the edge. Adding more water will allow water to "climb" over the edge.

So in this case the answer is that you can't even quite fill the cylinder.

By the way, the "creep" is a capillary effect. Indeed they are both surface tension effects. Making the radius of the cylinder large minimizes the effect, but it won't go away no matter how large $r$ is.