2
$\begingroup$

As the picture shows below

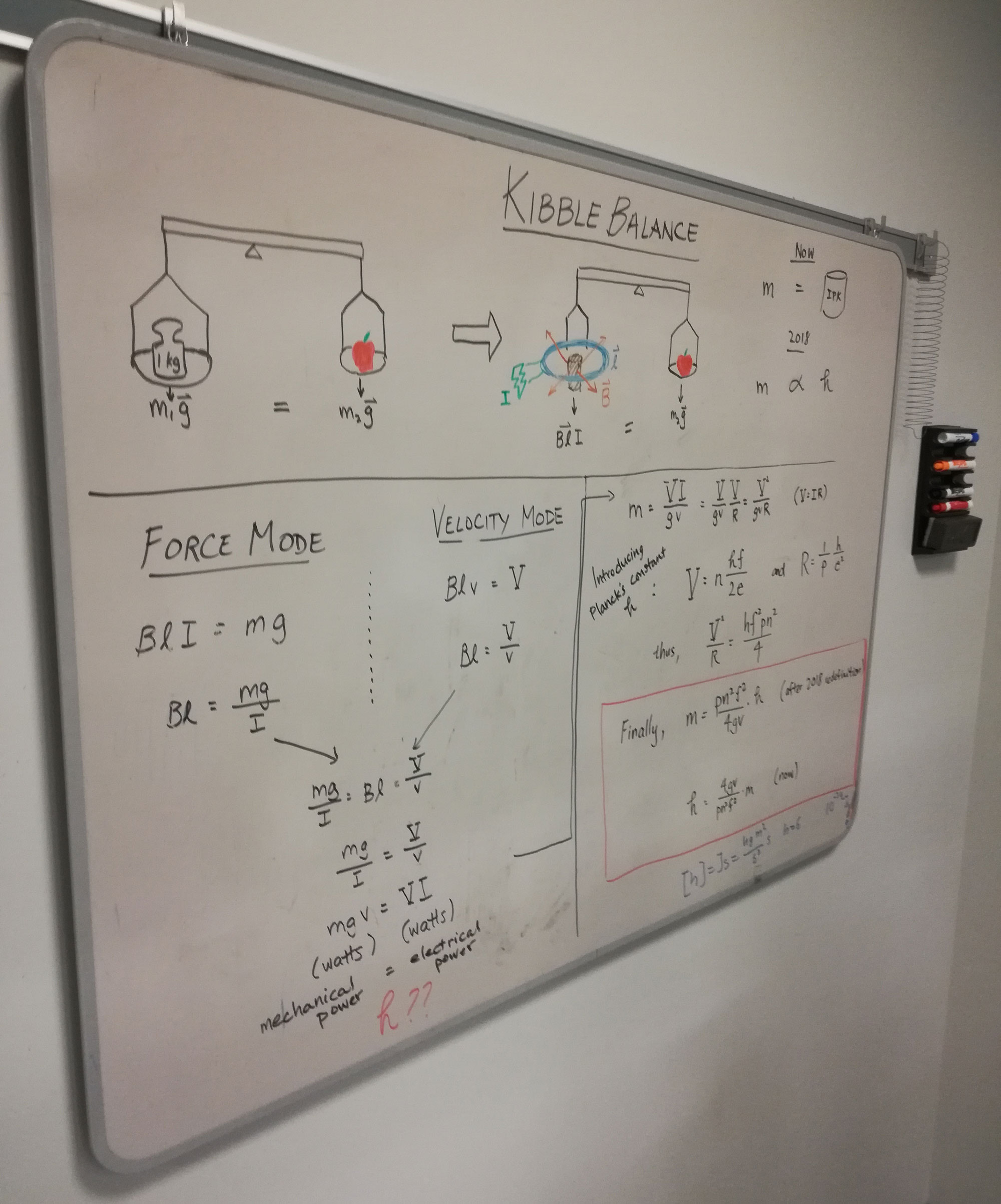[][1]

in a Kibble balance, one can drop out the measurement uncertainty of $B$ (magnetic flux intensity) and $L$ (length of coil) by the use of two modes, force mode and velocity mode.

And the voltage and electric current (resistor) value is determined by the Josephson effect and the quantum Hall effect; these two phenomena are what permits the measurement of electrical quantities in terms of the Planck constant to the precision required for the watt balance and redefinition.

So before the 2018 Nov 16 General Conference on Weights and Measures (CGPM) in Versailles, France, we measure the Planck constant using a known reference mass, such as Le Grand K. And, at the 2018 Nov 16 BIPM,the Planck constant, as defined by the ISO standard, was set to $6.626070150\times 10^{-34}\:\rm J \cdot s$ exactly.

That is, before the 2018 redefinition of units, the equation in the red rectangle is used to measure $h$ from a mass traceable to the IPK. After the redefinition, the equation will be used to realize the definition of the kilogram from a fixed value of $h$ in joule-seconds.

And I saw a Lego version kibble balance, designed by NIST people, reported in the paper

  • A LEGO Watt balance: An apparatus to determine a mass based on the new SI. LS Chao et al. Am. J. Phys. 83, 913 (2015); L. S. Chao, S. Schlamminger, D. B. Newell, and J. R. Pratt

which states

Before the 2018 redefinition of units, the equation [...] is used to measure $h$ from a mass traceable to the IPK. After redefinition, the equation [...] will be used to realize the definition of the kilogram from a fixed value of $h$ in joule-seconds.

In a classroom setting, quantum electrical standards are typically unavailable. However, it is still possible to measure the Planck constant due to the way the present unit system is structured. While the SI is used for most measurements, a different system of units has been used worldwide for almost all electrical measurements since 1990. For these so-called conventional units, the Josephson and von Klitzing constants were fixed at values adjusted to the best knowledge in 1989. These fixed values are named “conventional Josephson” and “conventional von Klitzing” constants and are abbreviated $K_{J–90}$ and $R_{K–90}$, respectively. Since 1990, almost all electrical measurements are calibrated in conventional units. By comparing electrical power in conventional units to mechanical power in SI units, $h$ can be determined.

How to understand this?

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ I do not really understand what kind of classroom setting the people at NIST had in mind. It is unfortunate that the new definition of the kg is unexplainable in school. $\endgroup$
    – user137289
    Commented Dec 12, 2018 at 9:10

2 Answers 2

Reset to default
2
$\begingroup$

Basically, since 1990, the bulk of all electrical measurement equipment has been calibrated to measurements that are traceable to fixed values of $h$ and $e$. When we talk about redefining the SI units, on the electrical side, this is best understood as simply elevating those previously non-SI units to full SI status. (For more details see this larger thread.)

When you're doing the measurement in the Chao et al. paper, you're making a comparison between two measurements of power which are traceable to two different standards:

  • On one side, you're measuring mechanical power using a test weight whose mass is traceable to the IPK.
  • On the other side, you're measuring electrical power using electrical measurement equipment whose calibration is traceable to the conventional electrical units, i.e. to $K_{J\text{-}90}$ and $R_{K\text{-}90}$.

The connection to $h$ is via the latter, since your electrical equipment has been calibrated to a fixed (though non-SI) value of $h$. By comparing this value to a measurement of an identical quantity made exclusively using SI-traceable measurements of mass, speed and time.

Ultimately, of course, in a classroom setting, you've lost so much precision in the calibration chain on both sides that you're unlikely to get more than two significant figures out of the process (their result, $h/h_{90}=0.998$, looks to me to be rather more precise than what you'll be able to do in a regular classroom). But the traceability principles look sound to me.

Improve this answer
$\endgroup$
0
$\begingroup$

The measurement provided by the LEGO Kibble balance does NOT constitute a primary mass standard. This is because the measurement is NOT in any way directly related to Plancks constant $h$.

Rather, the authors claim, the volt and ammeters used to measure the voltages and currents in the lego machine are $h$-traceable in that they were calibrated to standards that were at point calibrated to standards... that were calibrated in a way related to the Josephson effect.

What's impressive about the LEOG Kibble balance is that it can pedagogically demonstrate how the complex Kibble balances used at advanced metrological labs work and that it is surprising accurate.

Improve this answer
$\endgroup$
11
  • $\begingroup$ A note on semantics: A primary calibration simply refers to one which does not reference another measurement of the same type (e.g. a mass against another mass). You could build a Kibble balance out of dried mud and a galvanometer from 1850, and it would be “primary,” but it would also have large uncertainty. Even the best Kibble balances in the world have a nonzero uncertainty because there are many intermediate measurements which connect the exactly defined physical constants to the mass measurement (e.g. local gravity, force constant vs. position, laser alignment, mechanical repeatability). $\endgroup$
    – Gilbert
    Commented Jul 18 at 17:34
  • $\begingroup$ @Gilbert yeah I think you're right.. I think what I say in my answer is basically wrong. They have electronics calibrated to $h$ and they use those to measure some velocities, calibrate some forces etc. and ultimately measure mass. In the real Kibble balances the voltages and currents don't need to be calibrated because they're directly realized locally. But for the LEGO Kibble balances it's ok that the voltages/currents are just calibrated and not direct realizations of the voltage standards because Kibble balance isn't a voltage standard, it's a mass standard. Just like you say. $\endgroup$
    – Jagerber48
    Commented Jul 18 at 17:51
  • $\begingroup$ @Gilbert NO! I take it back. The question asks how a LEGO Kibble balance can measure $h$. The answer is it can't. The voltages and currents involved in it are calibrated to $h$. So it's circular to say the LEGO balance measure $h$. $\endgroup$
    – Jagerber48
    Commented Jul 18 at 18:29
  • $\begingroup$ I think a Kibble balance using such $h$-calibrated voltages could be used to measure mass. But it can't be used as a primary standard for $h$ or mass. $\endgroup$
    – Jagerber48
    Commented Jul 18 at 18:29
  • $\begingroup$ Kibble balances are in principle used as a standard for mass. All a standard is is a protocol/apparatus for making a primary measurement (likely with the minimum uncertainty available) and which is recognized and adopted by the relevant standards organization(s). Kibble balance can fill this role for the SI, following the SI redefinition of 2019. But it happens that the situation for mass is currently a little complicated because there has been some slight disagreement between Kibble balances from different countries during "key comparisons" (where they all take turns measuring the same mass). $\endgroup$
    – Gilbert
    Commented Oct 25 at 13:41

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.