3
$\begingroup$

I am currently in Physics 1 and we are learning about Energy. This may sound like a dumb question but I am currently trying to understand this. Here is the question of the problem:

A 10kg block is dropped from a height of 20meters onto a spring with a spring constant of 2500Nm. How much will the spring be compressed?

My initial equation was: $$PE_i = PE_f \implies mgh = \frac12kx^2$$ I was wrong and the actual equation that should have been used was: $$mg(h+x) = \frac12kx^2$$ My question: Why is it $mg(h+x)$ instead of $mgh$?

Improve this question
$\endgroup$
2
  • $\begingroup$ Depends. When they say "from a height of 20 meters," is the 20 meters measured from the top of the spring or from the bottom of the spring? $\endgroup$
    – probably_someone
    Commented Nov 24, 2018 at 22:39
  • $\begingroup$ @probably_someone i think the h is the height which is measured if the spring was in equilibrium with the block's weight if the block didn't fall . As op's mention of mg(h+x) as the potential energy. $\endgroup$
    – Nobody recognizeable
    Commented Nov 24, 2018 at 23:03

2 Answers 2

Reset to default
3
$\begingroup$

The other answer is right, but I find it a little hard to read. Here is my attempt to clarify.

The total amount of energy never changes. It just moves between three compartments: gravitational potential energy, spring potential energy, and kinetic energy.

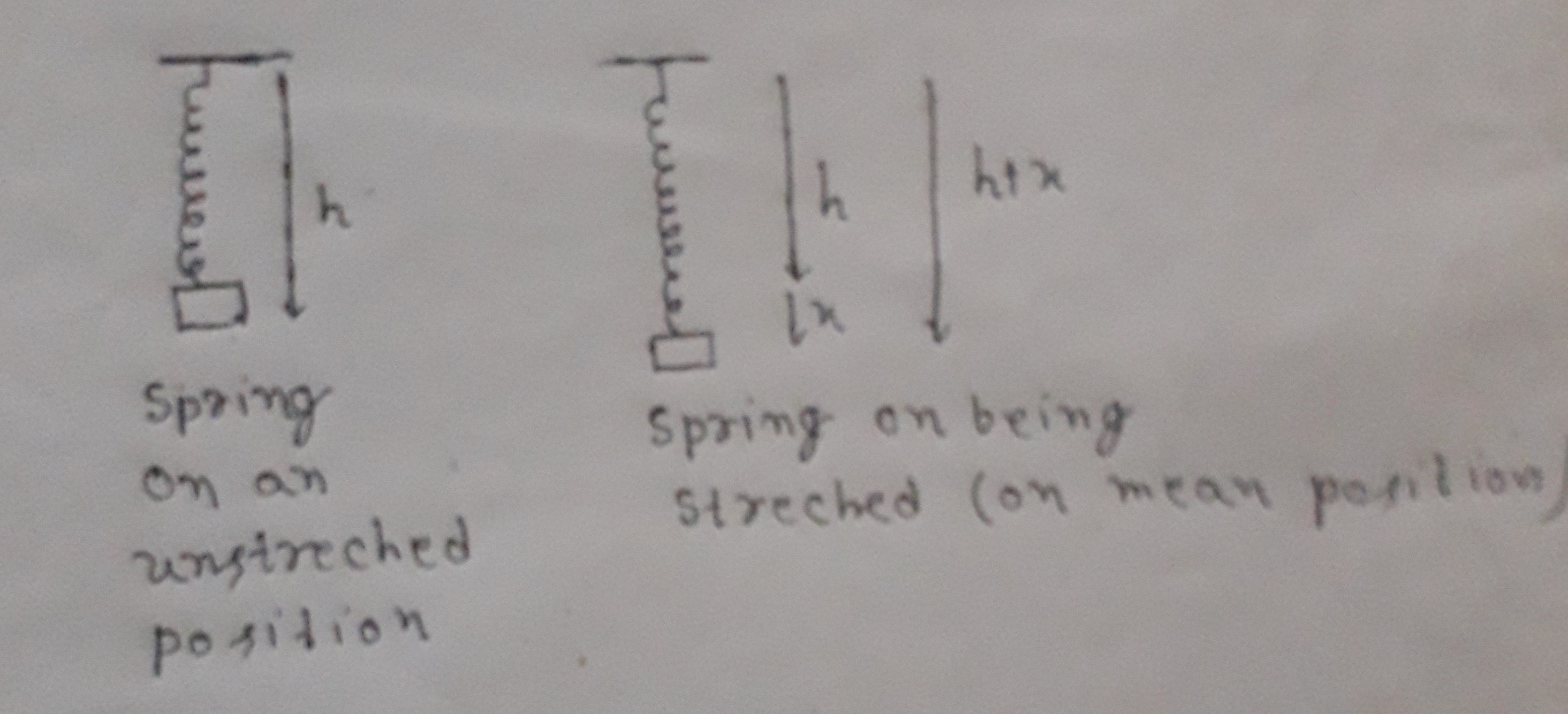
Two of these compartments have natural zeros: kinetic energy is zero when the block isn't moving, and spring potential energy is zero when the spring is not compressed. But when it comes to the third compartment--gravitational potential energy--you get to choose where zero is. In your answer, you have chosen it to be zero when the block first touches the spring: that's how you get $PE_i=mgh$.

Since kinetic energy is zero when the block reaches its lowest point, all the energy at that point is in the other two compartments. The spring potential energy, as you surmise, is $\frac{1}{2}kx^2$, but the gravitational potential energy is not zero--you already decided that it's zero when the spring is uncompressed. Since you've moved down from that point by a distance $x$, the gravitational potential energy is negative: it's $-mgx$.

So, $$PE_i = PE_f \implies mgh = \frac12kx^2 - mgx.$$

Improve this answer
$\endgroup$
2
  • $\begingroup$ Technically you can choose where the $0$ is for the spring potential energy too $\endgroup$
    – BioPhysicist
    Commented Nov 25, 2018 at 3:46
  • 1
    $\begingroup$ True enough. I guess I just mean it’s a natural choice. $\endgroup$
    – Ben51
    Commented Nov 25, 2018 at 12:02
0
$\begingroup$

The spring is not stable state even after the ball fell h height ie the ball continues to move a x distance . Now due to restoring force and velocity the ball proceeds furuher to extend the spring. Now with respect to the heighest extension of spring the potential energy of the spring is $mg(h+x)$ which is equal to work done by the wire for extending and also the gravity does work +mgx after the fall of height h of the block so the potential energy stored indeed was mg(h+x). (Note that the spring only applies restoring force after passing the equilibrium position because before that the spring was slack . So energy loss by spring is just $kx^2/2$ ; not $k(x+h)^2/2$. ) And also note that the potential energy is calculated with respect to the mean position of the spring ie the most bottom point of the spring after being streched). enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.