Graphing $\psi(x)$ given $\mid \Psi(x) \mid^2$ piece-wise

Question

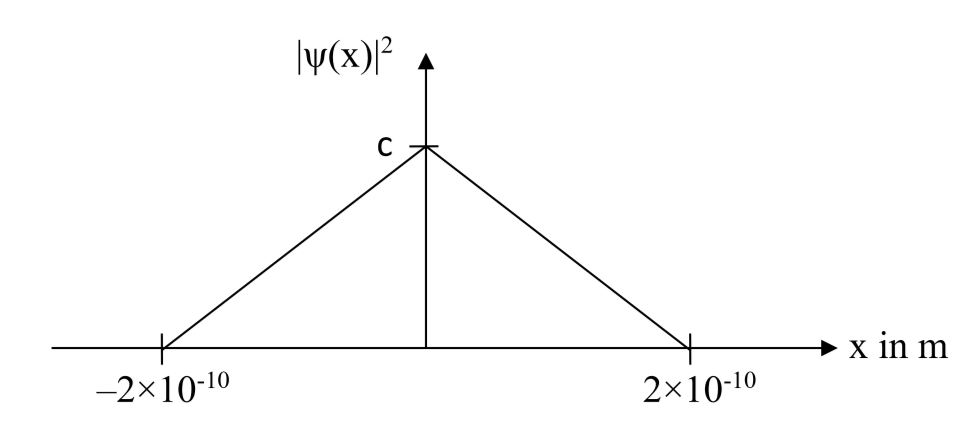
I am tasked with finding $\psi (x)$ of the following piecewise function:

This function obviously appears to not be differentiable, but am asked to consider it to approximate to a smooth wavefunction. I am asked to sketch this $\psi(x)$ pertaining to this function.

Firstly, I believe I (rightly) described this function with piecewise as:

$$\mid \Psi(x) \mid^2 \ = \begin{cases} 2.5*10^{19}x+\frac{1}{2*10^{-10}}, & \text{$(-2*10^{-10}) \le x \le 0$} \\ -2.5*10^{19}x+\frac{1}{2*10^{-10}}, & \text{$0 \le x \le (2*10^{-10})$} \end{cases}$$

We are then asked to assume that $\psi(x)$ is real only.

However, I feel a bit weird about my sketch for $\psi(x)$. I'll type it here. I'll also simplify the mess for my piecewise function a bit above.

$$ \psi(x) \ = \begin{cases} \sqrt{2.5*10^{19}x+\frac{1}{2*10^{-10}}}, & \text{$(-2*10^{-10}) \le x \le 0$} \\ \sqrt{-2.5*10^{19}x+\frac{1}{2*10^{-10}}}, & \text{$0 \le x \le (2*10^{-10})$} \end{cases} $$

However, due to the extremely high gradients of this function, trying to graph it ends up basically approximating $\psi(x) = \delta (x)$, Where $\delta(x)$ is the dirac delta function. However, this seems to take away the piecewise nature of this function, and, given $\psi(x)$, I can't seem to understand how normalizing it would bring me back to $\mid \Psi(x) \mid^2$ as I would need to. Perhaps the devil's in the details, and this isn't truly the dirac delta function? I just would have to state this is the function's arguments and basically draw it as the dirac delta function?

How should I approach this sketch? And how can this end up resembling $\mid \Psi(x) \mid^2$? I'm essentially not confident with my work but I'm not sure what I did wrong.

Answer 1

Your equation to your own question is correct. But take a look in a more compact form : \begin{equation} \psi(x)\boldsymbol{=} \left. \begin{cases} \sqrt{c\boldsymbol{-}c^2\boldsymbol{\vert} x\boldsymbol{\vert}\vphantom{\tfrac12}}\,, \quad x \boldsymbol{\in} \left[\boldsymbol{-}c^{\boldsymbol{-}1},\boldsymbol{+}c^{\boldsymbol{-}1}\right]\\ \hphantom{\sqrt{c\boldsymbol{-}}}0\hphantom{\sqrt{c\boldsymbol{-}}} \,, \quad x \boldsymbol{\notin} \left[\boldsymbol{-}c^{\boldsymbol{-}1},\boldsymbol{+}c^{\boldsymbol{-}1}\right] \end{cases} \right\} \,, \quad c=\boldsymbol{+}5\cdot10^9 \tag{01}\label{01} \end{equation} Note that $\:\boldsymbol{\vert}\psi(x)\boldsymbol{\vert}^2\:$ is very close to the Dirac $\:\delta-$function \begin{equation} \lim_{c\boldsymbol{\rightarrow}\boldsymbol{+}\infty}\boldsymbol{\vert}\psi(x)\boldsymbol{\vert}^2\boldsymbol{=}\delta(x) \tag{02}\label{02} \end{equation}

$================================================$

\begin{equation} \psi(x)\boldsymbol{=}\alpha^{{\boldsymbol{-}1}}\sqrt{\bigl[\theta(x\!\boldsymbol{+}\!\alpha)\!\boldsymbol{+}\!\theta(x\!\boldsymbol{-}\!\alpha)\!\boldsymbol{-}\!2\theta(x)\bigr]x\!\boldsymbol{+}\!\bigl[\theta(x\!\boldsymbol{+}\!\alpha)\!\boldsymbol{-}\!\theta(x\!\boldsymbol{-}\!\alpha)\bigr]\alpha}\,, \quad\alpha=c^{\boldsymbol{-}1} \tag{99}\label{99} \end{equation}