Static equilibrium - Ladder against a wall, how far can person ascend

Question

A uniform ladder of weight $W$ and length $2L$ rests against rough wall(coefficient of friction $2/3$) and has the lower end on a rough floor(coefficient of friction $1/4$) the ladder is inclined at an angle $X(\theta)$ with the horizontal where $\tan X=5/3,$ calculate how far a person weighing $3W$ can ascend the ladder.

Now I've resolved the forces acting vertically where we have $R$(the normal force acting vertically from the bottom of the ladder) is equal to $W$(the weight of the ladder) + $2/3S$(our friction force working vertically at the top end of the ladder with $S$ being the normal force reacting at that point) This gives us $R=W+\frac23S$

Resolving Horizontally I get $S=\frac14R$ as thats the friction force at the bottom of the ladder equal to the normal force working the other way at the top of the ladder.

Now for taking moments I decided to put my point of axis at the bottom of the ladder so having clockwise = anticlockwise I get this equation $W\cdot2L\cos X=S\cdot2L\sin X+\frac14S\cdot L\cos X$

Unfortunately this is where I am stuck I don't know how to apply the person weighing $3W$ into the question at this point and how to answer how far they could ascend the ladder I have watched so many separate torque videos online and none of them go into how far a person who isn't already on the ladder could ascend it so any help would be greatly appreciated on what i need to do next

Answer 1

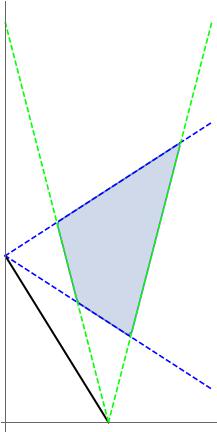
A way of simplifying the problem is to combine the two weights using the idea of centre of gravity.

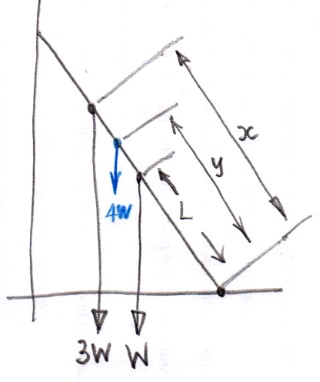
In your example the weight of the ladder $W$ positioned at a distance $L$ from the bottom of the ladder and the weight of the person positioned at an unknown distance from the bottom of the ladder $x$ can be combined to be a weight $4W$ at a position $y$ from the bottom of the ladder if $W\,L +3W\, x = 4W \, y$.
So you can now proceed having one weight $4W$ on the ladder and then find $y$ and hence find the required distance $x$.

However there is still a problem in that there is static friction at both ends of the ladder.
The simple way of solving such a problem is to assume that when the person gains maximum height the friction forces at both ends of the ladder are at a maximum given by $F = \mu \, N$ where $\mu$ is the coefficient of static friction and $N$ is the normal reaction.
Proving this to be the case is a little more difficult.

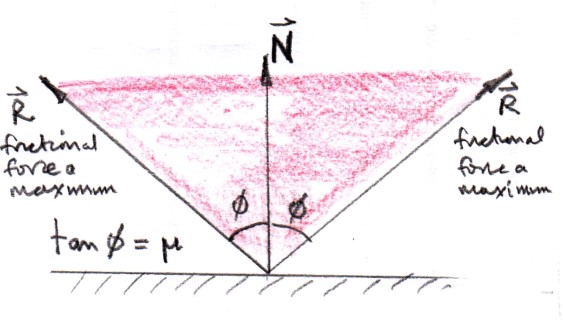
To do so let the resultant of the frictional force $\vec F$ and the normal reaction $\vec N$ be $\vec R$.
The frictional force can vary from a maximum value in one direction through zero to a maximum in the opposite direction so the resultant force $\vec R$ can point anywhere within the shaded area shown below.

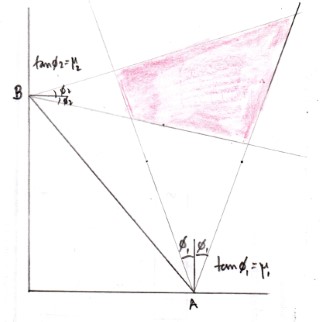
The coefficient of static friction differs at each end of the ladder is different so the range of directions of the resultant forces (friction and normal reaction) at each differ as shown below with the shaded area showing the region where the lines of action the two resultant forces can intersect.

The problem has now been reduced to having three forces acting on the ladder.
A force on the ladder due to the ground $\vec R_{\rm g}$ , a force on the ladder due to the wall $\vec R_{\rm w}$ and a force on the ladder and person climbing it due to the gravitational attraction of the Earth $4\vec W$.

For these three forces to be in equilibrium their lines of action must intersect at a point.
Remembering that you are asked for the maximum height to which the person can climb the diagram below shows that if this condition is to be satisfied the static frictional force at both the ground and the wall must be a maximum.

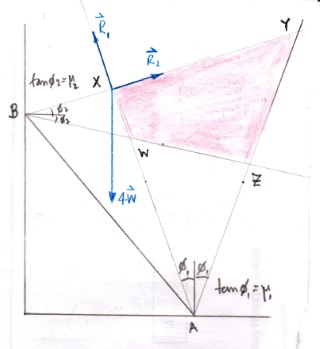
Vertex $X$ of the quadrilateral $WXYZ$ is at the furthest point to the left for which there can be stable equilibrium and that represents the furthest to the left that the force $\vec 4W$ can act where the person is at the highest point on the ladder.

Update because of a comment by @AaronStevens
If the coefficient of friction at the bottom of the ladder gets smaller point $X$ in the diagram will move to the right.
The ladder alone cannot be in stable equilibrium if the coefficient of static friction at the bottom of the ladder is such that point $X$ is to the right of the centre of gravity of the ladder alone.
If there is no friction at the bottom of the ladder the ladder cannot be in stable equilibrium as there is no way of compensating for the horizontal component of force $\vec R_2$, the normal reaction at the top of the ladder.
It is possible for the ladder to be in stable equilibrium if there is no friction at the top of the ladder and friction at the bottom of the ladder.
The quadrilateral $WXYZ$ then degenerates into a horizontal straight line and if the left-hand end of that line is to the right of the centre of mass of the centre of mass of the ladder alone, stable equilibrium is not possible for the ladder alone.

So you need to look at each problem individually and rhere are some mechanics problems which you cannot solve without extra information.
For example if the line of action of the weight $4\vec W$ passed through the vertex $W$ of quadrilateral $WXYZ$ there is a range of values of $\vec R_1$ and $\vec R_2$ for which the ladder and person is in stable equilibrium,

It is a pity that the edX course 2.01 x Elements of Structures no longer exists even in archived form but you can still view a nice video about a Statically Indeterminate Problem which shows that for some mechanics problems one needs extra information to solve the problem.

Answer 2

Calling

$$ \begin{array}{lcll} p_1 & = & (0,2L\sin\theta) &\mbox{ladder wall contact point}\\ p_2 & = & (2L\cos\theta) &\mbox{ladder floor contact point}\\ p_x & = & p_2 + x(-\cos\theta,\sin\theta)&\mbox{person location on the ladder}\\ p_W & = & p_2 + L(-\cos\theta,\sin\theta)&\mbox{ladder barycenter}\\ F_1 & = & (h_1,v_1)&\mbox{Ladder contact force at $p_1$ }\\ F_2 & = & (-h_2,v_2)&\mbox{Ladder contact force at $p_2$ }\\ W & = & (0,w)&\mbox{ladder weight}\\ P & = & (0, 3w)&\mbox{person weight}\\ \mu_1 & = & &\mbox{wall friction coefficient}\\ \mu_2 & = & & \mbox{floor friction coefficient} \end{array} $$

The equations for static equilibrium are

$$ R = F_1+F_2+W+P = 0\\ M_O = p_1 \times F_1 + p_2 \times F_2+p_W \times W + p_x\times P = 0 $$

The non slipping conditions are

$$ \left|\frac{v_1}{h_1}\right| \le \mu_1\\ \left|\frac{h_2}{v_2}\right| \le \mu_2\\ $$

In the attached figure in dashed blue, the limits for non slipping at $p_1$ representing the region $\left|\frac{v_1}{h_1}\right| \le \mu_1$ analogously for $\left|\frac{h_2}{v_2}\right| \le \mu_2$ in dashed green.

In non slipping condition, the support lines for $F_1$ and $F_2$ will cross inside or at the boundary of the delimited light blue region.

The slipping condition over the ladder demands that for non slipping they must be observed at least one of

$$ \left|\frac{v_1}{h_1}\right| \le \mu_1\\ \left|\frac{h_2}{v_2}\right| \le \mu_2\\ $$

now solving the equilibrium equations for $h_1,h_2,v_1$ we have

$$ \left\{ \begin{array}{rcl} h_-h_2 & = & 0 \\ v _1+v_2-4 w & = & 0 \\ 2 L v_2 \cos \theta-7 L w \cos\theta+3 w x \cos\theta-2 h_1 L \sin\theta& = &0 \\ \end{array} \right. $$ giving $$ \frac{v_1}{h_1} = \frac{w(L+3x)}{2Lh_1}-\tan\theta\\ \left(\frac{h_2}{v_2}\right)^{-1} = \frac{(7L-3x)w}{2L h_2}+\tan\theta $$

We leave the exercise at this point, so that the reader is in charge of solving the final steps.